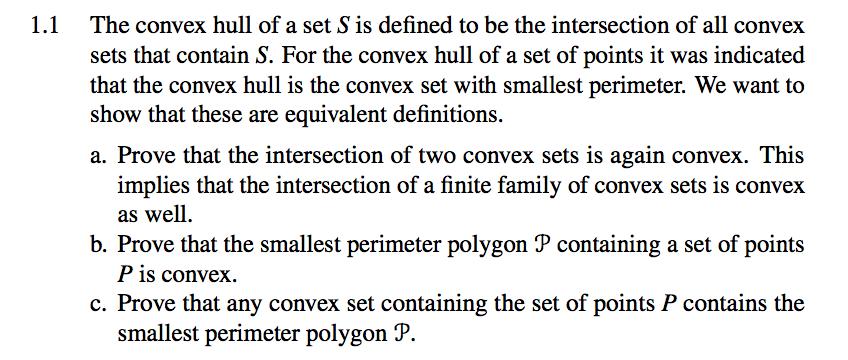
1.1 The convex hull of a set S is defined to be the intersection of all convex sets that contain S. For the convex hull of a set of points it was indicated that the convex hull is the convex set with smallest perimeter. We want to show that these are equivalent definitions. a. Prove that the intersection of two convex sets is again convex. This implies that the intersection of a finite family of convex sets is convex as well. b. Prove that the smallest perimeter polygon P containing a set of points P is convex. c. Prove that any convex set containing the set of points P contains the smallest perimeter polygon P.