Answered step by step
Verified Expert Solution
Question
1 Approved Answer
1.2, Elementary properties Let gamma :z=z(t),tin(a,b) . We define the opposite arc, written -gamma , by -gamma :z=z(-t),tin(-b;-a) Then, int_(-7) f(z)dz=int_(-b)^(-a) f(z(-t))(d)/(dt)[z(-t)]dt=-int_(-b)^(-a) f(z(-t))(dz)/(dt)(-t)dt=-int_a^b f(z(t))(dz)/(dt)(t)dt
1.2,Elementary properties\ Let
\\\\gamma :z=z(t),tin(a,b). We define the opposite arc, written
-\\\\gamma , by\
-\\\\gamma :z=z(-t),tin(-b;-a)\ Then,\
\\\\int_(-7) f(z)dz=\\\\int_(-b)^(-a) f(z(-t))(d)/(dt)[z(-t)]dt=-\\\\int_(-b)^(-a) f(z(-t))(dz)/(dt)(-t)dt=-\\\\int_a^b f(z(t))(dz)/(dt)(t)dt\ where the last equality is obtained with a simple change of variable. Hence\
\\\\int_(-\\\\gamma ) f(z)dz=-\\\\int_(\\\\gamma ) f(z)dz 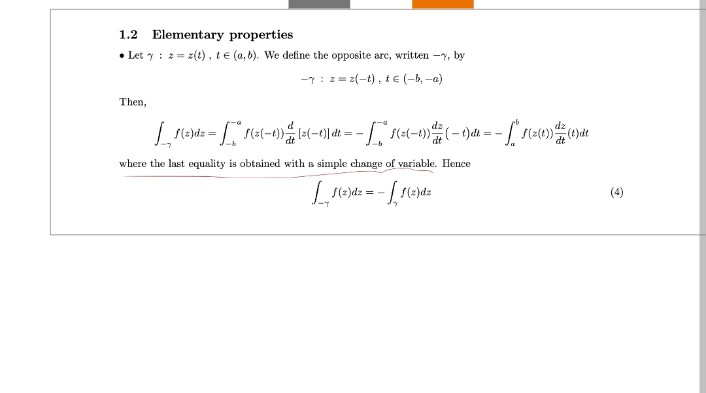
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


