Question
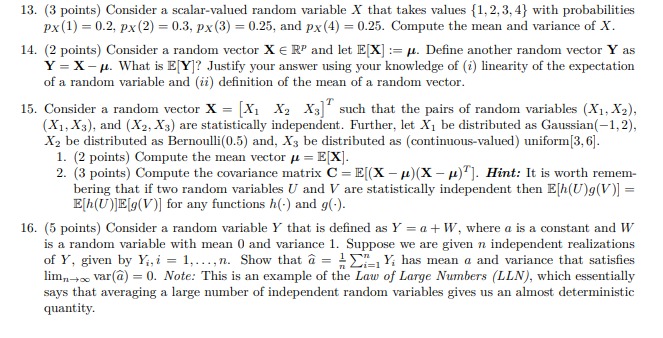
13. (3 points) Consider a scalar-valued random variable X that takes values {1,2,3,4} with probabilities Px(1) = 0.2, px (2) = 0.3, px(3) = 0.25,
13. (3 points) Consider a scalar-valued random variable X that takes values {1,2,3,4} with probabilities Px(1) = 0.2, px (2) = 0.3, px(3) = 0.25, and px (4) = 0.25. Compute the mean and variance of X. 14. (2 points) Consider a random vector X ERP and let E[X] :=u. Define another random vector Y as Y = X-u. What is E[Y]? Justify your answer using your knowledge of (i) linearity of the expectation of a random variable and (ii) definition of the mean of a random vector. 15. Consider a random vector X = X1 X2 X3' such that the pairs of random variables (X1, X2), (X1, X3), and (X2, X3) are statistically independent. Further, let X be distributed as Gaussian(-1,2), X2 be distributed as Bernoulli(0.5) and, X3 be distributed as continuous-valued) uniform(3,6). 1. (2 points) Compute the mean vector i=EX. 2. (3 points) Compute the covariance matrix C = E(X- X-H)]. Hint: It is worth remem- bering that if two random variables U and V are statistically independent then Eh(U)g(V) = E[h(U)]E[9(V)] for any functions h) and g). 16. (5 points) Consider a random variable Y that is defined as Y = a +W, where a is a constant and W is a random variable with mean 0 and variance 1. Suppose we are given n independent realizations of Y, given by Yi, i = 1,...,n. Show that a = - Y; has mean a and variance that satisfies limto var(a) = 0. Note: This is an example of the Law of Large Numbers (LLN), which essentially says that averaging a large number of independent random variables gives us an almost deterministic quantity.
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


