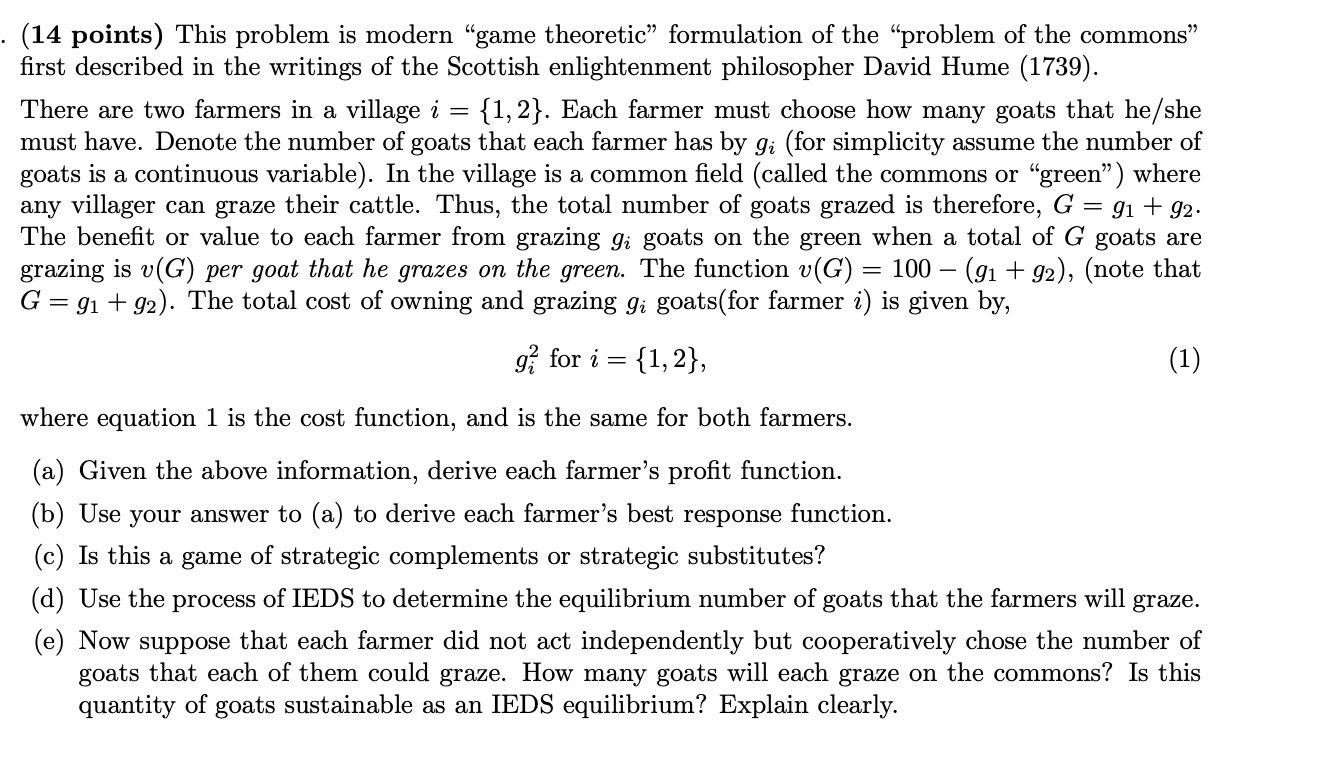
(14 points) This problem is modern "game theoretic" formulation of the problem of the commons" first described in the writings of the Scottish enlightenment philosopher David Hume (1739). There are two farmers in a village i = {1,2}. Each farmer must choose how many goats that he/she must have. Denote the number of goats that each farmer has by gi (for simplicity assume the number of goats is a continuous variable). In the village is a common field (called the commons or green) where any villager can graze their cattle. Thus, the total number of goats grazed is therefore, G 91 + 92 The benefit or value to each farmer from grazing gi goats on the green when a total of G goats are grazing is v(G) per goat that he grazes on the green. The function v(G) = 100 (91 +92), (note that G = 91 +92). The total cost of owning and grazing gi goats(for farmer i) is given by, g for i = {1,2}, (1) where equation 1 is the cost function, and is the same for both farmers. (a) Given the above information, derive each farmer's profit function. (b) Use your answer to (a) to derive each farmer's best response function. (c) Is this a game of strategic complements or strategic substitutes? (d) Use the process of IEDS to determine the equilibrium number of goats that the farmers will graze. (e) Now suppose that each farmer did not act independently but cooperatively chose the number of goats that each of them could graze. How many goats will each graze on the commons? Is this quantity of goats sustainable as an IEDS equilibrium? Explain clearly. (14 points) This problem is modern "game theoretic" formulation of the problem of the commons" first described in the writings of the Scottish enlightenment philosopher David Hume (1739). There are two farmers in a village i = {1,2}. Each farmer must choose how many goats that he/she must have. Denote the number of goats that each farmer has by gi (for simplicity assume the number of goats is a continuous variable). In the village is a common field (called the commons or green) where any villager can graze their cattle. Thus, the total number of goats grazed is therefore, G 91 + 92 The benefit or value to each farmer from grazing gi goats on the green when a total of G goats are grazing is v(G) per goat that he grazes on the green. The function v(G) = 100 (91 +92), (note that G = 91 +92). The total cost of owning and grazing gi goats(for farmer i) is given by, g for i = {1,2}, (1) where equation 1 is the cost function, and is the same for both farmers. (a) Given the above information, derive each farmer's profit function. (b) Use your answer to (a) to derive each farmer's best response function. (c) Is this a game of strategic complements or strategic substitutes? (d) Use the process of IEDS to determine the equilibrium number of goats that the farmers will graze. (e) Now suppose that each farmer did not act independently but cooperatively chose the number of goats that each of them could graze. How many goats will each graze on the commons? Is this quantity of goats sustainable as an IEDS equilibrium? Explain clearly







