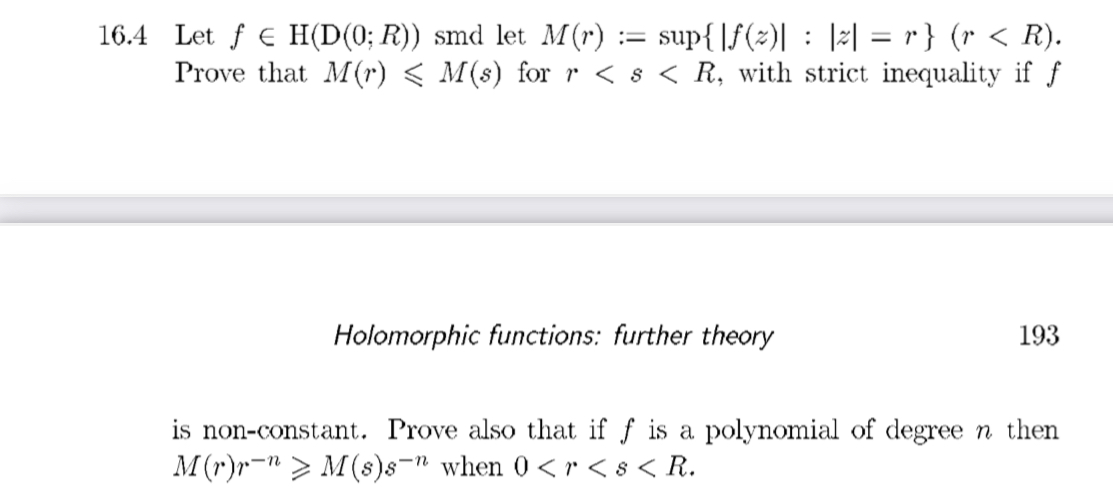
16.4 Let f H(D(0; R)) smd let M(r) := sup{|f(z)| = |2| = r} (r < R). Prove that M(r) < M(s) for r < s < R, with strict inequality if Holomorphic functions: further theory 193 is non-constant. Prove also that if f is a polynomial of degree n then M(r) M(s)sn when 0 < r < s < R.