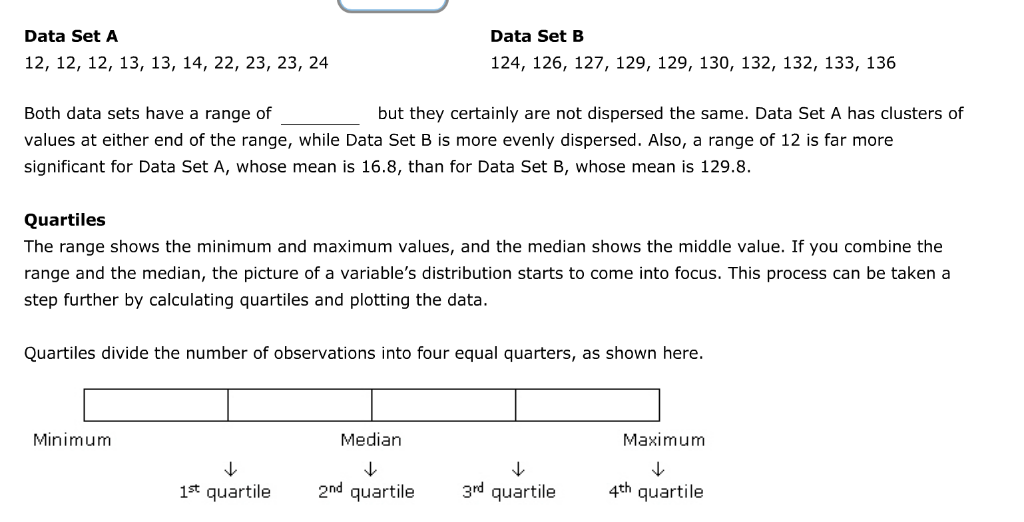
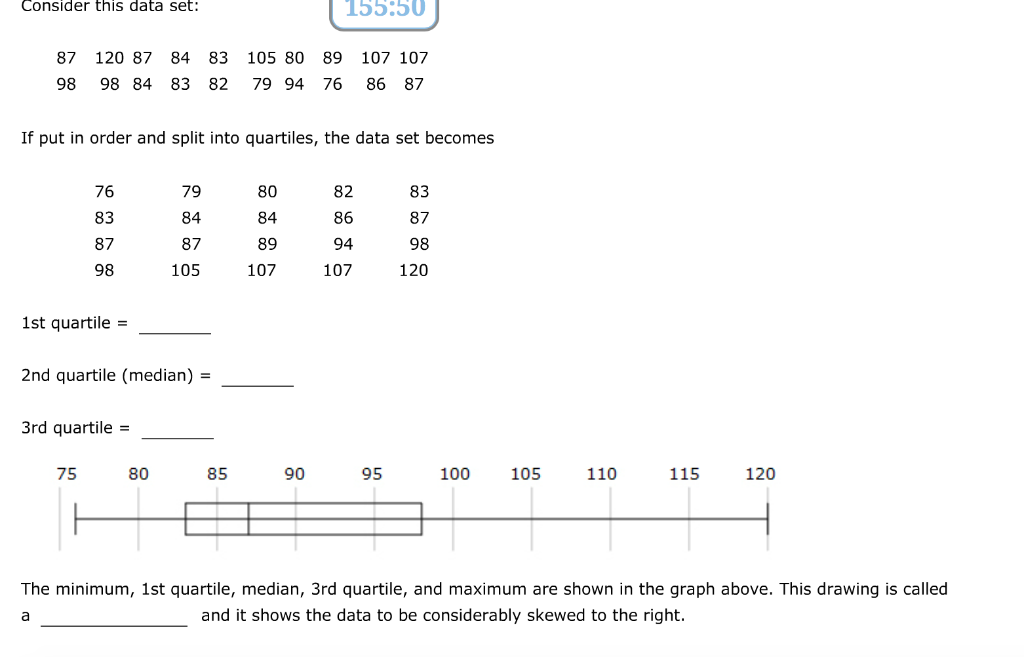
17. Measures of dispersion Aa Aa Suppose two stocks have the same mean expected return (10%). However, one stock always has a 10% return, while the other stock is very unpredictable. Which one would you want? Probably the first one, right? This section defines some basic measures of dispersion, also known as spread or variability, and how they help interpret data. Range The simplest measure of dispersion is range, which is defined as the span of values that a variable takes. You can find the range by determining the difference between the maximum and minimum values, as follows: Range = Maximum value-Minimum value The range is useful because it is easy to calculate and even easier to understand. Comparing the range and the mean indicates how significant the dispersion is. However, the range does not measure the dispersion of the majority of the observations; it focuses only on the highest and lowest values. Therefore, it is very sensitive to outliers. Consider the following two 10-item data sets: Data Set A Data Set B 12, 12, 12, 13, 13, 14, 22, 23, 23, 24 124, 126, 127, 129, 129, 130, 132, 132, 133, 136 Both data sets have a range of values at either end of the range, while Data Set B is more evenly dispersed. Also, a range of 12 is far more significant for Data Set A, whose mean is 16.8, than for Data Set B, whose mean is 129.8. but they certainly are not dispersed the same. Data Set A has clusters of Quartiles The range shows the minimum and maximum values, and the median shows the middle value. If you combine the range and the median, the picture of a variable's distribution starts to come into focus. This process can be taken a step further by calculating quartiles and plotting the data. Quartiles divide the number of observations into four equal quarters, as shown here. Minimum Median Maximum 1t quartile nd quartile 3d quartile 4th quartile 155.50 Consider this data set: 87 120 87 84 83 105 80 89 107 107 98 98 84 83 82 79 94 76 86 87 If put in order and split into quartiles, the data set becomes 83 87 98 120 82 86 94 107 80 84 89 107 79 84 87 105 76 83 87 98 1st quartile- 2nd quartile (median)- 3rd quartile - 120 115 110 105 100 95 85 90 80 75 The minimum, 1st quartile, median, 3rd quartile, and maximum are shown in the graph above. This drawing is called and it shows the data to be considerably skewed to the right 17. Measures of dispersion Aa Aa Suppose two stocks have the same mean expected return (10%). However, one stock always has a 10% return, while the other stock is very unpredictable. Which one would you want? Probably the first one, right? This section defines some basic measures of dispersion, also known as spread or variability, and how they help interpret data. Range The simplest measure of dispersion is range, which is defined as the span of values that a variable takes. You can find the range by determining the difference between the maximum and minimum values, as follows: Range = Maximum value-Minimum value The range is useful because it is easy to calculate and even easier to understand. Comparing the range and the mean indicates how significant the dispersion is. However, the range does not measure the dispersion of the majority of the observations; it focuses only on the highest and lowest values. Therefore, it is very sensitive to outliers. Consider the following two 10-item data sets: Data Set A Data Set B 12, 12, 12, 13, 13, 14, 22, 23, 23, 24 124, 126, 127, 129, 129, 130, 132, 132, 133, 136 Both data sets have a range of values at either end of the range, while Data Set B is more evenly dispersed. Also, a range of 12 is far more significant for Data Set A, whose mean is 16.8, than for Data Set B, whose mean is 129.8. but they certainly are not dispersed the same. Data Set A has clusters of Quartiles The range shows the minimum and maximum values, and the median shows the middle value. If you combine the range and the median, the picture of a variable's distribution starts to come into focus. This process can be taken a step further by calculating quartiles and plotting the data. Quartiles divide the number of observations into four equal quarters, as shown here. Minimum Median Maximum 1t quartile nd quartile 3d quartile 4th quartile 155.50 Consider this data set: 87 120 87 84 83 105 80 89 107 107 98 98 84 83 82 79 94 76 86 87 If put in order and split into quartiles, the data set becomes 83 87 98 120 82 86 94 107 80 84 89 107 79 84 87 105 76 83 87 98 1st quartile- 2nd quartile (median)- 3rd quartile - 120 115 110 105 100 95 85 90 80 75 The minimum, 1st quartile, median, 3rd quartile, and maximum are shown in the graph above. This drawing is called and it shows the data to be considerably skewed to the right