1.The makers of an exercise device advertise that using their machine a few minutes per day results in an average weight loss of 4 kilograms during the first month. A consumer group believes the average weight loss would be less than this. The consumer group recruited 36 volunteers to use the product in accordance with the manufacturer's recommendations. They found an average weight loss of 3.2 kilograms with a standard deviation of 1.8 kilograms. You are told that the calculated test statistic is ?2.67. Assume the conditions are satisfied.
a) Write the hypotheses to test the consumer group's claim.
b) Give the critical value if testing at the 1% level of significance.
c) Give your decision and briefly explain your reasoning.
d) Give your conclusion with respect to the consumer group's claim.
e) Explain a Type I error in the context of this question.
2.Say, Apple Inc. claims that 27% of all Apple device users own an iPad. If a random sample of 315 Apple device users is selected, what is the Z score if 37% of those sampled own an iPad? Assume the conditions are satisfied. Give your answer correctly rounded to two decimal places.
3.The production manager at a textile mill, believes that a new machine, producing cloth, is not working according to the company's specifications. The company requires that the cloth should have a mean breaking strength of 38 Kg. A sample of 91 pieces of cloth reveals a sample mean breaking strength of 39.2 Kg and a standard deviation of 0.5 Kg. If the manager wants to do hypothesis test to her belief, what value of the mean would be used in the null and the alternative hypotheses?
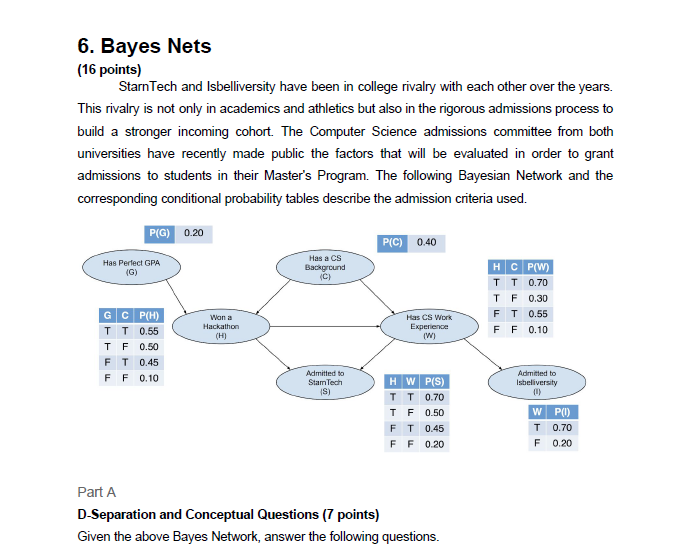
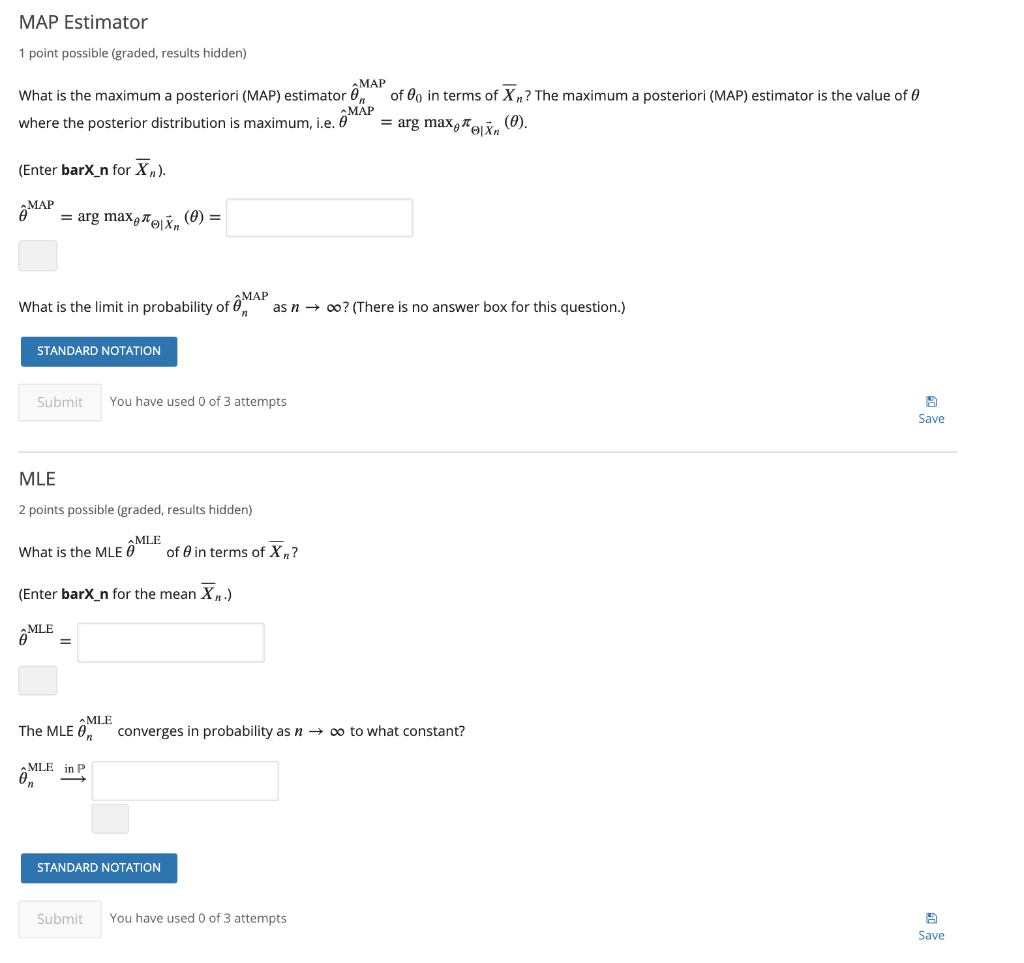
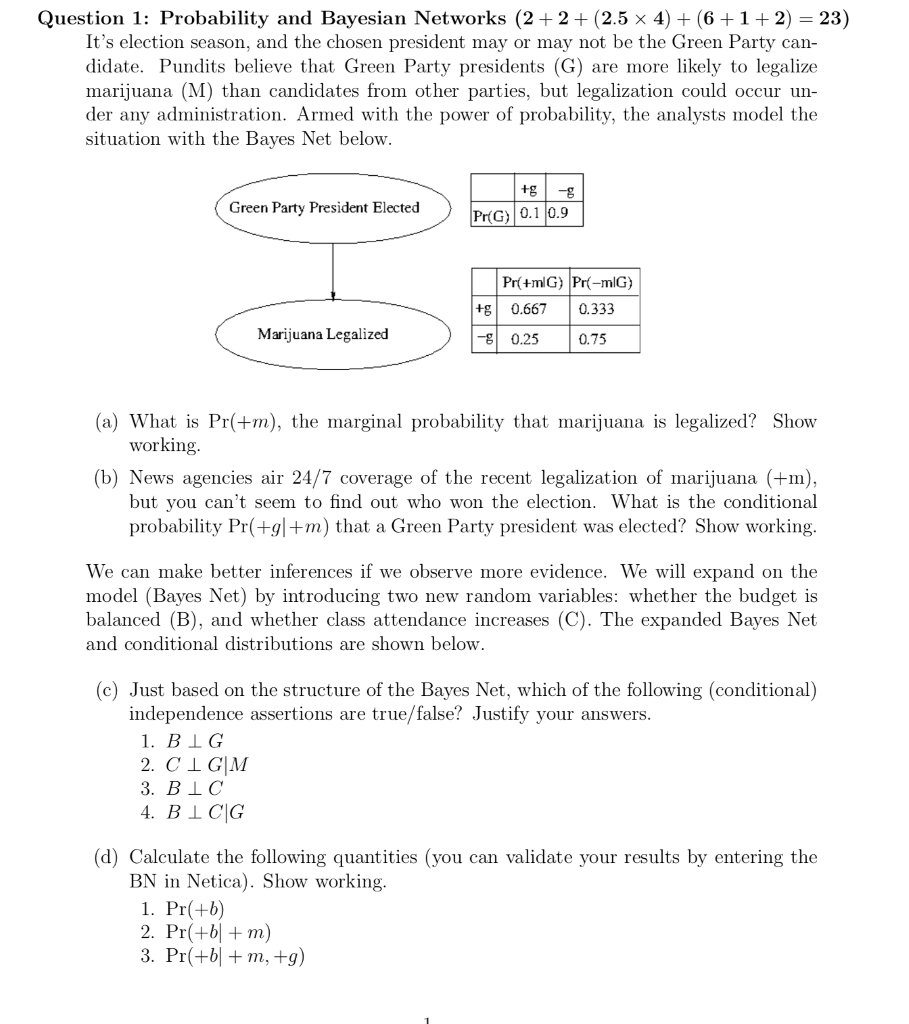
6. Bayes Nets (16 points) StarTech and Isbelliversity have been in college rivalry with each other over the years. This rivalry is not only in academics and athletics but also in the rigorous admissions process to build a stronger incoming cohort. The Computer Science admissions committee from both universities have recently made public the factors that will be evaluated in order to grant admissions to students in their Master's Program. The following Bayesian Network and the corresponding conditional probability tables describe the admission criteria used. P(G) 0.20 P(C) 0.40 Has a CS Has Perfect GPA Background H C P(W) (G) T T 0.70 T F 0.30 G C P(H) Has CS Work F T 0.55 Wan a Hackathon Experience F F 0.10 T T 0.55 CHI T F 0.50 F T 0.45 Admitted to Admitted to F F 0.10 StamTech H W P(S) Isbelliversity T T 0.70 T F 0.50 W P(1) F T 0.45 T 0.70 F F 0.20 F 0.20 Part A D-Separation and Conceptual Questions (7 points) Given the above Bayes Network, answer the following questions.7. Bookmark this page Setup: Suppose you observe an i.i.d. sample X1, ..., Xn of a Bernoulli random variable X with unknown parameter 60 = P (X = 1). We use the Bayesian approach to statistical inference and model the unknown parameter Go as a random variable @ (defined jointly with X). That is, let the conditional distribution of X given O be X|0 ~ Ber (0) and let * (0) = 30-1 (0 E [0, 1]) be the prior distribution of O. Let P denote the probabilities implied by the Bayesian model. Useful Facts: The Beta (a, /) distribution has pdf f ( 1 ) = 1-1(1 -1)0-1 B(a, p) where B(a, B) = 2-1(1 -18-1 di, and Mean a + B Variance (a+ ) (a+ p+ 1 ) Mode = argmax f a- 1 = atB-2 for a, B > 1. The mode is the value of the random variable at which the pdf attains its maximum.MAP Estimator 1 point possible (graded, results hidden) What is the maximum a posteriori (MAP) estimator 0, of Go in terms of X? The maximum a posteriori (MAP) estimator is the value of 0 where the posterior distribution is maximum, i.e. MAP = arg maxgojxn (). (Enter barX_n for X , ). MAP = arg max fox, (0) = What is the limit in probability of 0, as n - co? (There is no answer box for this question.) STANDARD NOTATION Submit You have used 0 of 3 attempts Save MLE 2 points possible (graded, results hidden) What is the MLE O AMLE of 0 in terms of X , ? (Enter barX_n for the mean X,.) MLE -MLE The MLE On converges in probability as n - co to what constant? AMLE in P STANDARD NOTATION Submit You have used 0 of 3 attempts H SaveQuestion 1: Probability and Bayesian Networks (2 + 2 + [2.5 x 4) + (6 + 1 + 2) = 23) It's election season, and the chosen president may or may not be the Green Party can- didate. Pundits believe that Green Party presidents (G) are more likely to legalize marijuana (M) than candidates from other parties, but legalization could occur un der any administration. Armed with the power of probability, the analysts model the situation with the Bayes Net below. Green Party Presidmi Elected (a) What is Pr(+m), the marginal probability that marijuana is legalized? Show working. m I-- E-- [b] News agencies air 24/? coverage of the recent legalization of marijuana (+m), but you can't seem to nd out who won the election. What is the conditional probability Pr[+g| +m) that a Green F'art},r president was elected? Show working. We can make better inferences if we observe more evidence. We will expand on the model (Bayes Not) by introducing two new random variables: whether the budget is balanced (B), and whether class attendance increases (C). The expanded Bayes Net and conditional distributions are shown below. (c) Just based on the structure of the Bayes Net, which of the following (conditional) independence assertions are true,\" false? Justify your answers. 1. Bit? scram 3. Bit? 4. arms (d) Calculate the following quantities {you can validate your results by entering the BN in Netica). Show working. 1. Pr(+b) 2. Pr(+b| + m) 3. Pr(+b| + m.+g)