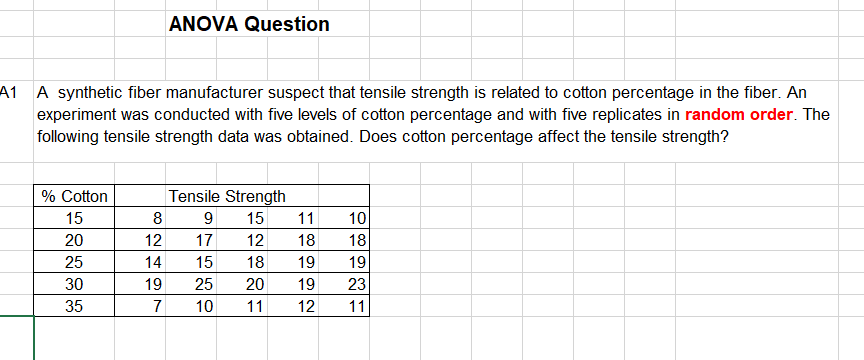
Question
1.What type of experimental design is employed in this problem? This is a randomized test design with various grinding machines from other suppliers assigned to
1.What type of experimental design is employed in this problem?
This is a randomized test design with various grinding machines from other suppliers assigned to surface roughness. Each design shows how the other grinding machines will provide the minor surface roughness.
2.Identify the treatments and the dependent variable (Response).
3.Use a multiple boxplot in order to compare responses between different levels.
a.Are there differences between the mean of the responses in at least two of the levels?
b.Do you think this is the result of within-group variation or between-group variation?
4.State clearly the hypothesis that we are testing in this problem.
a.Set up the null and alternative.
5.Run ANOVA on the data and generate the output with plots.
a.Comment on the degree of freedom values for each source of variation. How do you calculate them?
b.Do we reject the hypothesis that we are testing? Why or why not?
i.If you reject, can you tell which level(s) is probably the one(s) that has the different mean? (Hint: use the boxplots from part 1a)
ii.If you are suspicious of only one level, repeat the ANOVA without that level and see if you fail to reject the null in the test.
c.Use the histogram of residuals to comment on the normality of error term (residuals) in this ANOVA model.
Regression
1.Use a scatterplot in order to explain the relationship between X and Y
a.What do you expect for the sign of 1 and magnitude of 2?
2.After running the regression model in Minitab:
a.What is the estimated regression equation?
1.Interpret the estimate of 0 in the words of the problem. Do the same for 1. 0 = 1.49.
2.Conduct a test that the true slope of the model differs from 0.
3.Explain how to use the output of the regression for the test.
a.Relate the conclusion of your test to the scatterplot that you generated in (1)
b.Use the generated plots of the regression model (histogram of the residuals) in order to check the normality assumption of your model. While there is no need for a statistical test, you must compare the shape to a normal distribution pdf.
4.Generate the fitted line plot for this regression
Generate prediction interval for y* and confidence interval for () when * = ( average of given x's). Note that * is a point in units that we are interested in predicting the Minutes for it. Here, as an example, we use = 6 (average of column "unit") as x*. So in general you can pick any value for x*. For generating the intervals, we need to run the regression model again
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


