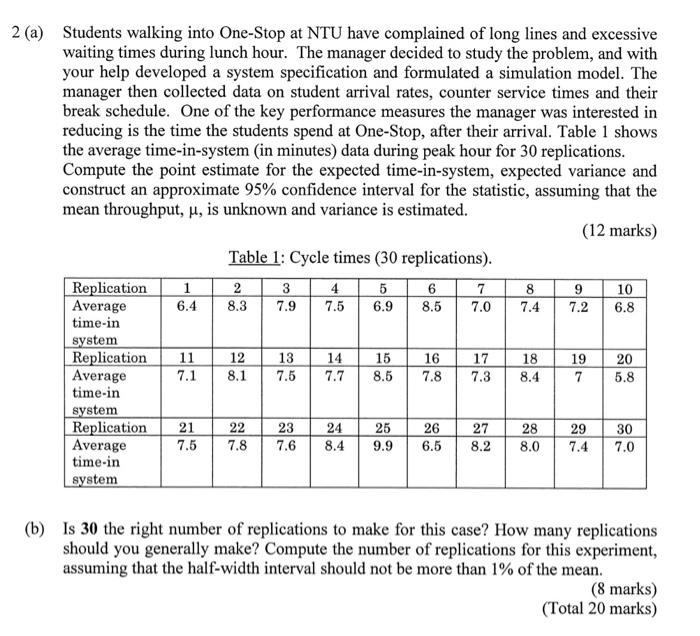
2 (a) Students walking into One-Stop at NTU have complained of long lines and excessive waiting times during lunch hour. The manager decided to study the problem, and with your help developed a system specification and formulated a simulation model. The manager then collected data on student arrival rates, counter service times and their break schedule. One of the key performance measures the manager was interested in reducing is the time the students spend at One-Stop, after their arrival. Table 1 shows the average time-in-system (in minutes) data during peak hour for 30 replications. Compute the point estimate for the expected time-in-system, expected variance and construct an approximate 95% confidence interval for the statistic, assuming that the mean throughput, p, is unknown and variance is estimated. (12 marks) Table 1: Cycle times (30 replications). Replication 12 3 6 7 9 10 Average 6.4 8.3 7.9 7.5 6.9 8.5 7.0 7.4 7.2 6.8 time-in system Replication 11 12 13 14 15 16 19 20 Average 7.1 8.1 7.5 7.7 8.5 7.8 7.3 8.4 7 5.8 time-in system Replication 21 22 23 26 27 28 29 30 Average 7.5 7.8 7.6 8.4 9.9 6.5 8.2 8.0 7.4 7.0 time-in system 8 17 18 . 24 25 (b) Is 30 the right number of replications to make for this case? How many replications should you generally make? Compute the number of replications for this experiment, assuming that the half-width interval should not be more than 1% of the mean. (8 marks) (Total 20 marks) 2 (a) Students walking into One-Stop at NTU have complained of long lines and excessive waiting times during lunch hour. The manager decided to study the problem, and with your help developed a system specification and formulated a simulation model. The manager then collected data on student arrival rates, counter service times and their break schedule. One of the key performance measures the manager was interested in reducing is the time the students spend at One-Stop, after their arrival. Table 1 shows the average time-in-system (in minutes) data during peak hour for 30 replications. Compute the point estimate for the expected time-in-system, expected variance and construct an approximate 95% confidence interval for the statistic, assuming that the mean throughput, p, is unknown and variance is estimated. (12 marks) Table 1: Cycle times (30 replications). Replication 12 3 6 7 9 10 Average 6.4 8.3 7.9 7.5 6.9 8.5 7.0 7.4 7.2 6.8 time-in system Replication 11 12 13 14 15 16 19 20 Average 7.1 8.1 7.5 7.7 8.5 7.8 7.3 8.4 7 5.8 time-in system Replication 21 22 23 26 27 28 29 30 Average 7.5 7.8 7.6 8.4 9.9 6.5 8.2 8.0 7.4 7.0 time-in system 8 17 18 . 24 25 (b) Is 30 the right number of replications to make for this case? How many replications should you generally make? Compute the number of replications for this experiment, assuming that the half-width interval should not be more than 1% of the mean. (8 marks) (Total 20 marks)