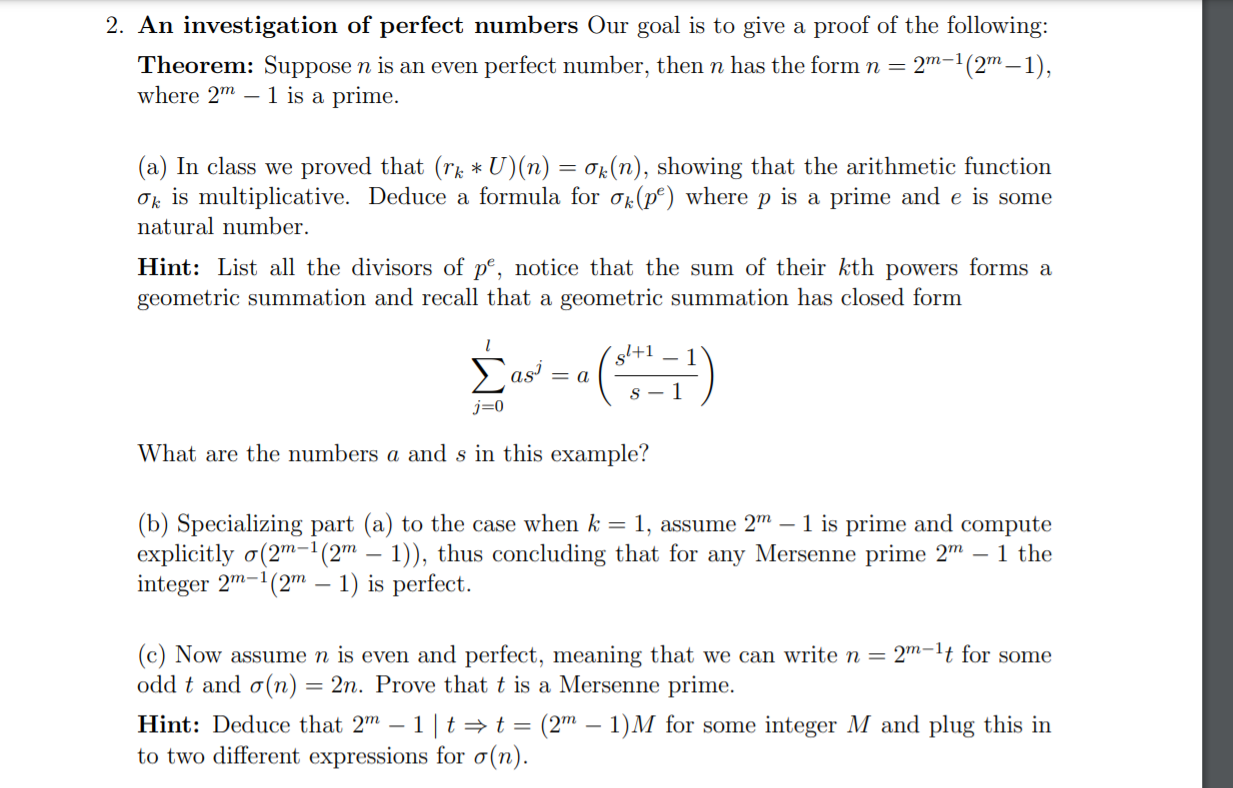
2. An investigation of perfect numbers Our goal is to give a proof of the following: Theorem: Suppose n is an even perfect number, then n has the form n = 2m-1(2M 1), where 2m 1 is a prime. (a) In class we proved that (rk * U)(n) = 0k(n), showing that the arithmetic function Ok is multiplicative. Deduce a formula for ok (p) where p is a prime and e is some natural number. Hint: List all the divisors of p, notice that the sum of their kth powers forms a geometric summation and recall that a geometric summation has closed form 1 $1+1 = a j=0 What are the numbers a and s in this example? (b) Specializing part (a) to the case when k = 1, assume 2 1 is prime and compute explicitly o(2-1(2m 1)), thus concluding that for any Mersenne prime 2m 1 the integer 2m-1(2m 1) is perfect. (c) Now assume n is even and perfect, meaning that we can write n = 2m-1t for some odd t and o(n) = 2n. Prove that t is a Mersenne prime. Hint: Deduce that 2" 1| tt = (2" 1)M for some integer M and plug this in to two different expressions for o(n). 2. An investigation of perfect numbers Our goal is to give a proof of the following: Theorem: Suppose n is an even perfect number, then n has the form n = 2m-1(2M 1), where 2m 1 is a prime. (a) In class we proved that (rk * U)(n) = 0k(n), showing that the arithmetic function Ok is multiplicative. Deduce a formula for ok (p) where p is a prime and e is some natural number. Hint: List all the divisors of p, notice that the sum of their kth powers forms a geometric summation and recall that a geometric summation has closed form 1 $1+1 = a j=0 What are the numbers a and s in this example? (b) Specializing part (a) to the case when k = 1, assume 2 1 is prime and compute explicitly o(2-1(2m 1)), thus concluding that for any Mersenne prime 2m 1 the integer 2m-1(2m 1) is perfect. (c) Now assume n is even and perfect, meaning that we can write n = 2m-1t for some odd t and o(n) = 2n. Prove that t is a Mersenne prime. Hint: Deduce that 2" 1| tt = (2" 1)M for some integer M and plug this in to two different expressions for o(n)