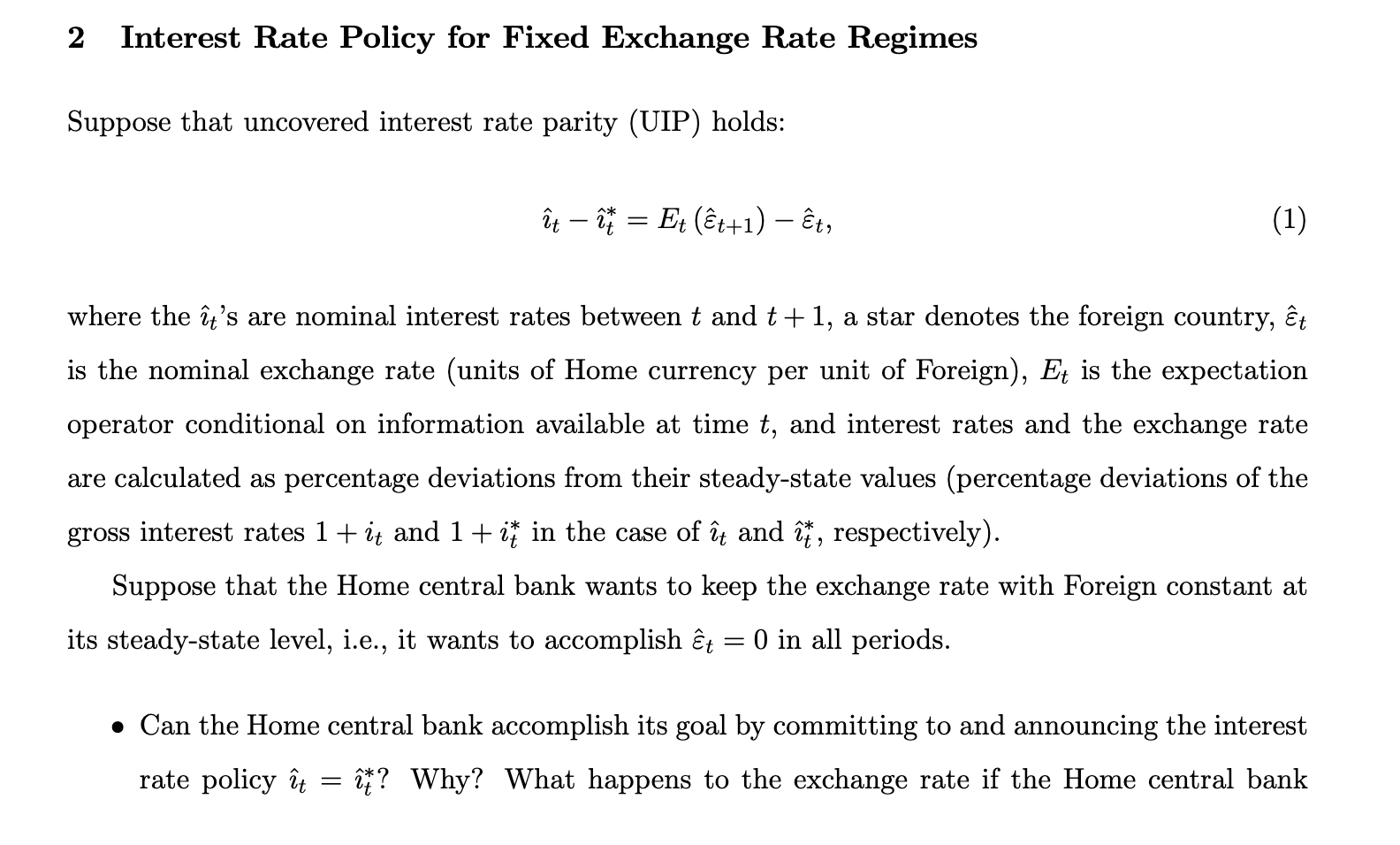
2 Interest Rate Policy for Fixed Exchange Rate Regimes Suppose that uncovered interest rate parity (UIP) holds: ^t^t=Et(^t+1)^t, where the ^t 's are nominal interest rates between t and t+1, a star denotes the foreign country, ^t is the nominal exchange rate (units of Home currency per unit of Foreign), Et is the expectation operator conditional on information available at time t, and interest rates and the exchange rate are calculated as percentage deviations from their steady-state values (percentage deviations of the gross interest rates 1+it and 1+it in the case of ^t and ^t, respectively). Suppose that the Home central bank wants to keep the exchange rate with Foreign constant at its steady-state level, i.e., it wants to accomplish ^t=0 in all periods. - Can the Home central bank accomplish its goal by committing to and announcing the interest rate policy ^t=^t ? Why? What happens to the exchange rate if the Home central bank commits to and announces the policy ^t=^t ? Suppose the Home central bank commits to and announces the interest rate policy: ^t=^t+^twith>0 This means that the Home central bank commits to shadowing the policy of the Foreign central bank and to increasing the interest rate t^t if the currency weakens ( ^t rises) or lowering the interest rate ^t if the currency strengthens ( ^t falls). The central bank announces this commitment and is completely credible. Furthermore, assume that the Home central bank and government are fully and credibly committed to the fact that they would never let the currency become worthless. (This means that the Home central bank and government would never accept a situation in which ^t=. Drastic actions would be taken such that ^t remains finite.) - Use the UIP condition (1), the policy rule (2), the fact that these two equations must hold in each period ( t,t+1,t+2, and so on), and the fact that we assumed policy commitments to be fully credible to prove that the policy rule (2) will deliver ^t=0 in all periods. (In developing this proof, you will have to use the Law of Iterated Expectations: Under rational expectations, the expectation today of the expectation tomorrow of something the day after tomorrow is equal to the expectation today of that something. Formally, Et[Et+1(xt+2)]=Et(xt+2) for any variable x. Note that this applies also to longer horizons: Et[Et+1[Et+2[Et+3[Et+T(xt+T+1)]]]]=Et[Et+T(xt+T+1)]=Et(xt+T+1).) - What happens to ^t versus ^t if the Home central bank has been successful with its policy? - If the policy commitment has been successful, does the Home central bank actually need to exercise its threat of adjusting ^t in response to exchange rate movements? 2 Interest Rate Policy for Fixed Exchange Rate Regimes Suppose that uncovered interest rate parity (UIP) holds: ^t^t=Et(^t+1)^t, where the ^t 's are nominal interest rates between t and t+1, a star denotes the foreign country, ^t is the nominal exchange rate (units of Home currency per unit of Foreign), Et is the expectation operator conditional on information available at time t, and interest rates and the exchange rate are calculated as percentage deviations from their steady-state values (percentage deviations of the gross interest rates 1+it and 1+it in the case of ^t and ^t, respectively). Suppose that the Home central bank wants to keep the exchange rate with Foreign constant at its steady-state level, i.e., it wants to accomplish ^t=0 in all periods. - Can the Home central bank accomplish its goal by committing to and announcing the interest rate policy ^t=^t ? Why? What happens to the exchange rate if the Home central bank commits to and announces the policy ^t=^t ? Suppose the Home central bank commits to and announces the interest rate policy: ^t=^t+^twith>0 This means that the Home central bank commits to shadowing the policy of the Foreign central bank and to increasing the interest rate t^t if the currency weakens ( ^t rises) or lowering the interest rate ^t if the currency strengthens ( ^t falls). The central bank announces this commitment and is completely credible. Furthermore, assume that the Home central bank and government are fully and credibly committed to the fact that they would never let the currency become worthless. (This means that the Home central bank and government would never accept a situation in which ^t=. Drastic actions would be taken such that ^t remains finite.) - Use the UIP condition (1), the policy rule (2), the fact that these two equations must hold in each period ( t,t+1,t+2, and so on), and the fact that we assumed policy commitments to be fully credible to prove that the policy rule (2) will deliver ^t=0 in all periods. (In developing this proof, you will have to use the Law of Iterated Expectations: Under rational expectations, the expectation today of the expectation tomorrow of something the day after tomorrow is equal to the expectation today of that something. Formally, Et[Et+1(xt+2)]=Et(xt+2) for any variable x. Note that this applies also to longer horizons: Et[Et+1[Et+2[Et+3[Et+T(xt+T+1)]]]]=Et[Et+T(xt+T+1)]=Et(xt+T+1).) - What happens to ^t versus ^t if the Home central bank has been successful with its policy? - If the policy commitment has been successful, does the Home central bank actually need to exercise its threat of adjusting ^t in response to exchange rate movements