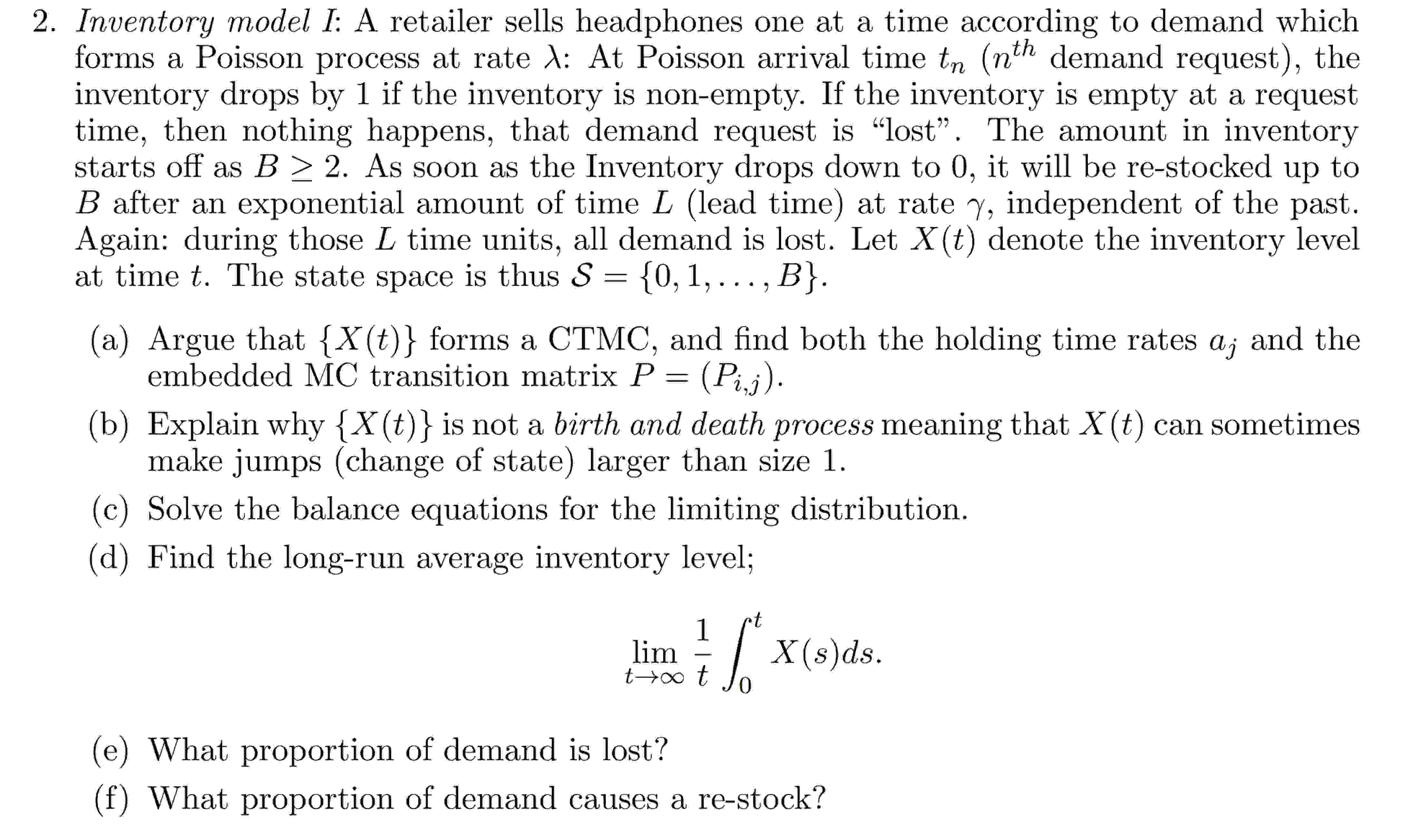
2. Inventory model I: A retailer sells headphones one at a time according to demand which forms a Poisson process at rate : At Poisson arrival time tn (nth demand request), the inventory drops by 1 if the inventory is non-empty. If the inventory is empty at a request time, then nothing happens, that demand request is "lost". The amount in inventory starts off as B > 2. As soon as the Inventory drops down to 0, it will be re-stocked up to B after an exponential amount of time L (lead time) at rate y, independent of the past. Again: during those L time units, all demand is lost. Let X(t) denote the inventory level at time t. The state space is thus S = {0, 1, {0, 1, ..., B}. j (a) Argue that {X(t)} forms a CTMC, and find both the holding time rates a; and the embedded MC transition matrix P = (Pi,j). (b) Explain why {X(t)} is not a birth and death process meaning that X (t) can sometimes make jumps (change of state) larger than size 1. (c) Solve the balance equations for the limiting distribution. (d) Find the long-run average inventory level; t 1 lim t t L X(s)ds. 0 (e) What proportion of demand is lost? (f) What proportion of demand causes a re-stock?