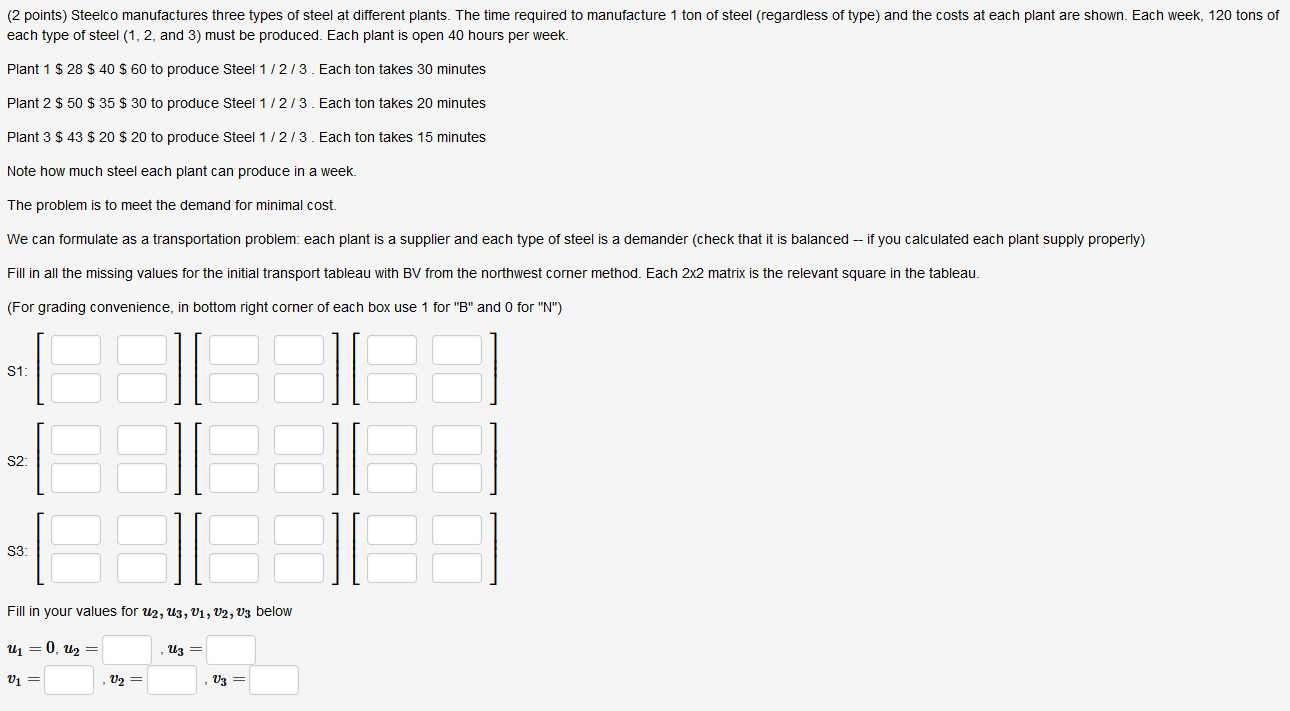
(2 points) Steelco manufactures three types of steel at different plants. The time required to manufacture 1 ton of steel (regardless of type) and the costs at each plant are shown. Each week, 120 tons of each type of steel (1, 2, and 3) must be produced. Each plant is open 40 hours per week. Plant 1 $ 28 $ 40 $ 60 to produce Steel 1/2/3. Each ton takes 30 minutes Plant 2 $ 50 $ 35 $ 30 to produce Steel 1/2/3. Each ton takes 20 minutes Plant 3 $ 43 $ 20 $ 20 to produce Steel 1/2/3. Each ton takes 15 minutes Note how much steel each plant can produce in a week. The problem is to meet the demand for minimal cost. We can formulate as a transportation problem: each plant is a supplier and each type of steel is a demander (check that it is balanced -- if you calculated each plant supply properly) Fill in all the missing values for the initial transport tableau with BV from the northwest corner method. Each 2x2 matrix is the relevant square in the tableau. (For grading convenience, in bottom right corner of each box use 1 for "B" and 0 for "N") S1: S2 S3 Fill in your values for U2, U3, 01, 02, 03 below U=0, uz = U3 = Vj V2 = U3 = (2 points) Steelco manufactures three types of steel at different plants. The time required to manufacture 1 ton of steel (regardless of type) and the costs at each plant are shown. Each week, 120 tons of each type of steel (1, 2, and 3) must be produced. Each plant is open 40 hours per week. Plant 1 $ 28 $ 40 $ 60 to produce Steel 1/2/3. Each ton takes 30 minutes Plant 2 $ 50 $ 35 $ 30 to produce Steel 1/2/3. Each ton takes 20 minutes Plant 3 $ 43 $ 20 $ 20 to produce Steel 1/2/3. Each ton takes 15 minutes Note how much steel each plant can produce in a week. The problem is to meet the demand for minimal cost. We can formulate as a transportation problem: each plant is a supplier and each type of steel is a demander (check that it is balanced -- if you calculated each plant supply properly) Fill in all the missing values for the initial transport tableau with BV from the northwest corner method. Each 2x2 matrix is the relevant square in the tableau. (For grading convenience, in bottom right corner of each box use 1 for "B" and 0 for "N") S1: S2 S3 Fill in your values for U2, U3, 01, 02, 03 below U=0, uz = U3 = Vj V2 = U3 =