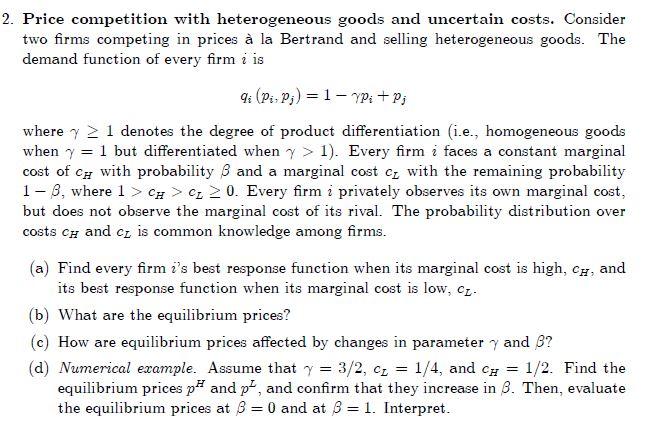
2. Price competition with heterogeneous goods and uncertain costs. Consider two firms competing in prices la Bertrand and selling heterogeneous goods. The demand function of every firm i is 9: (PPj) = 1 - YP:+P; where y > 1 denotes the degree of product differentiation (i.e., homogeneous goods when y = 1 but differentiated when y > 1). Every firm i faces a constant marginal cost of CH with probability 3 and a marginal cost oz with the remaining probability 1-B, where 1 > C > cz > 0. Every firm i privately observes its own marginal cost, but does not observe the marginal cost of its rival. The probability distribution over costs Ch and cu is common knowledge among firms. (a) Find every firm i's best response function when its marginal cost is high, Ch, and its best response function when its marginal cost is low, cz. (b) What are the equilibrium prices? (c) How are equilibrium prices affected by changes in parameter y and B? (d) Numerical example. Assume that y = 3/2, C2 = 1/4, and cu = 1/2. Find the equilibrium prices pH and p-, and confirm that they increase in 8. Then, evaluate the equilibrium prices at 8 = 0 and at 3 = 1. Interpret. 2. Price competition with heterogeneous goods and uncertain costs. Consider two firms competing in prices la Bertrand and selling heterogeneous goods. The demand function of every firm i is 9: (PPj) = 1 - YP:+P; where y > 1 denotes the degree of product differentiation (i.e., homogeneous goods when y = 1 but differentiated when y > 1). Every firm i faces a constant marginal cost of CH with probability 3 and a marginal cost oz with the remaining probability 1-B, where 1 > C > cz > 0. Every firm i privately observes its own marginal cost, but does not observe the marginal cost of its rival. The probability distribution over costs Ch and cu is common knowledge among firms. (a) Find every firm i's best response function when its marginal cost is high, Ch, and its best response function when its marginal cost is low, cz. (b) What are the equilibrium prices? (c) How are equilibrium prices affected by changes in parameter y and B? (d) Numerical example. Assume that y = 3/2, C2 = 1/4, and cu = 1/2. Find the equilibrium prices pH and p-, and confirm that they increase in 8. Then, evaluate the equilibrium prices at 8 = 0 and at 3 = 1. Interpret