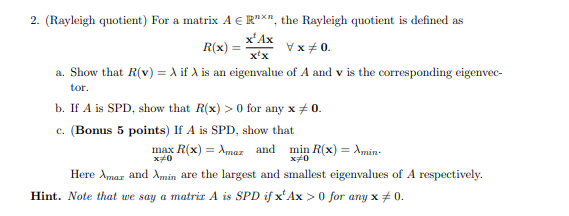
2. (Rayleigh quotient) For a matrix A Rx, the Rayleigh quotient is defined as R(x) = x'Ax xtx Vx 0. a. Show that R(v) = A if A is an eigenvalue of A and v is the corresponding eigenvec- tor. b. If A is SPD, show that R(x) > 0 for any x + 0. c. (Bonus 5 points) If A is SPD, show that max R(x) Amaz and x=0 min R(x) = Amin. x+0 Here Amar and Amin are the largest and smallest eigenvalues of A respectively. Hint. Note that we say a matrix A is SPD if x'Ax > 0 for any x +0.