Answered step by step

Verified Expert Solution

Link Copied!

Question

1 Approved Answer

Posted on Mar 25, 2024

2. Suppose that T is a contraction over the metric space (S, d) with modulus (0, 1). Prove that for any z, z' S

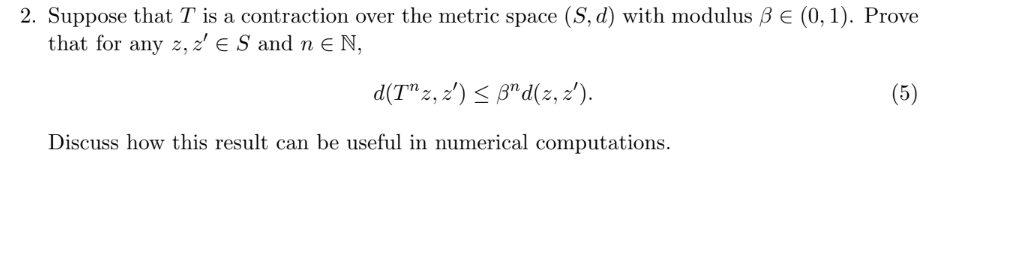
2. Suppose that T is a contraction over the metric space (S, d) with modulus (0, 1). Prove that for any z, z' S and n = N, d(Tz, z)nd(z, z'). Discuss how this result can be useful in numerical computations. (5)

Step by Step Solution

There are 3 Steps involved in it

Step: 1

Get Instant Access to Expert-Tailored Solutions

See step-by-step solutions with expert insights and AI powered tools for academic success

Step: 2

Step: 3

Ace Your Homework with AI

Get the answers you need in no time with our AI-driven, step-by-step assistance

Get Started

Recommended Textbook for

Linear Algebra

Authors: Jim Hefferon

1st Edition

978-0982406212, 0982406215

More Books

Students also viewed these Economics questions

Question

If net operating income is $385,000, the annual debt service is $267,000, What is the before tax cash flow from operations

Answered: 1 week ago

Question

Describe, and estimate the costs of, procedures to (a) insert a new item into an existing heap; (b) delete the topmost item from a non-empty heap; (c) starting from an array holding N items in...

Answered: 1 week ago

Question

Let A, B be sets. Define: (a) the Cartesian product (A B) (b) the set of relations R between A and B (c) the identity relation A on the set A [3 marks] Suppose S, T are relations between A and B, and...

Answered: 1 week ago

Question

★★★★★

Suppose that a countrys annual growth rates were 5, 3, 4, 1, 2, 2, 3, 4, 6, and 3 in yearly sequence over a 10-year period. What was the countrys trend rate of growth over this period? Which set of...

Answered: 1 week ago

Question

★★★★★

Consider the transition matrix (a) If Compute x(1), x(2), x(3), and x(4) to three decimal places. (b) Show that T is regular and find its steady state vector? 0 0.2 0.01 7-10 0.3 0.3 0.5 0.7

Answered: 1 week ago

Question

★★★★★

PQR Ltd. issued 5,000, 12% debentures of `100 each on April 1, 2016 to be matured on April 1, 2021. The market price of the debenture is `80. Compute the cost of existing debentures assuming 35% tax...

Answered: 1 week ago

Question

★★★★★

There are 10 blue and 16 red chips in a bowl. If we select five chips at random, what is the probability that more red chips are drawn than blue ones?

Answered: 1 week ago

Question

★★★★★

Assume that you are purchasing an investment and have decided to invest in a company in the digital phone business. You have narrowed the choice to Digital Plus Corp. and Red Zone, Inc. and have...

Answered: 1 week ago

Question

★★★★★

Question completion Status: QUESTION 16 Department Ahad 5000 wats in work in process that were 60%.completed as to labor overhead the beginning of the period; 4,000 units of direct materials were...

Answered: 1 week ago

Question

★★★★★

Complete the following tasks using the templates provided in FNSACC421_AT2_TMP_v1 and the information and data provided in Appendix A for Golden Steer, including their procedural guidelines. Enter...

Answered: 1 week ago

Question

★★★★★

All to be done in C++ no user input is required. Just need to create vectors and create functions to manipulate them as described. Search 2D Vector - Part 2 Write a C++ program that uses a function...

Answered: 1 week ago

Question

★★★★★

In 2021, Santa Fe Corporation had profits of $500,000 on sales of $10,000,000. At the beginning of 2021 Santa Fe's book equity was $2,500,000, and at the end of 2021 Santa Fe's total assets were...

Answered: 1 week ago

Question

★★★★★

What validations has Cvent put in place to ensure venues are receiving qualified leads? Cvent compares venues' sleeping room information to the planner's RFP sleeping room requirements before the RFP...

Answered: 1 week ago

Question

★★★★★

Changes to Australia's superannuation legislation now enable eligible employees to choose the superannuation fund they wish their super contributions to be paid into. Employers are legally obliged to...

Answered: 1 week ago

Question

★★★★★

= {x|ln 3.2 x 20} and two events A Problem 1. (6 points) Given a sample space S x 6} and B {x|3 x 7}, describe the following events. a) AUB. b) A - B. c) AU (BNS). = {x|2

Answered: 1 week ago

Question

★★★★★

Case Study You are a business analyst who has just joined the commission system replacement project at PrivateWealth. The project has been running for six months, and half of the planned analysis...

Answered: 1 week ago

Question

★★★★★

4) Z Corporation i... 12 Corporation, located in the United States, has an accounts receivable of 750 million yen in VHC year from a bank in Tokyo. The current spot rate is Yen 116/$1.00 and the one...

Answered: 1 week ago

Question

★★★★★

How much more interest will be earned if $5000 is invested for 6 years at 7% compounded continuously, instead of at 7% compounded quarterly?

Answered: 1 week ago

Question

★★★★★

Produce an automorphism of P2 other than the identity map, and other than a shift map p(x) p(x - k).

Answered: 1 week ago

Question

★★★★★

Show that the only eigenvalue of a nilpotent matrix is zero.

Answered: 1 week ago

Question

★★★★★

Doing the computations by hand, find the determinant of the matrix below. 6 2

Answered: 1 week ago

Question

★★★★★

In defending their experimental research with animals, psychologists have noted that animals biology and behavior can tell us much about our own. animals are fascinating creatures and worthy of...

Answered: 1 week ago

Question

★★★★★

What have you learned about sleep that you could apply to yourself?

Answered: 1 week ago

Question

★★★★★

If most information in the brain is processed outside of our awareness, how can we ever really know ourselves?

Answered: 1 week ago

Previous Question Next Question