2. Westfarmers Limited (WES) is an Australian company with diverse holdings, includ- ing retail, industry, etc.. It is Australia's largest private employer. Currently i.e., t=0), WES shares are trading at $39.21. You wish to purchase a European put on WES shares, with strike price $40.00, expiring after two time steps. Consider a two-step binomial model, with variable returns: R(0,0) = 1.03, R(1, 1) = 1.02 and R(1,0) = 1.04. Using CRR notation, assume u = 1.13 and d=1/u.

Please answer part (f)

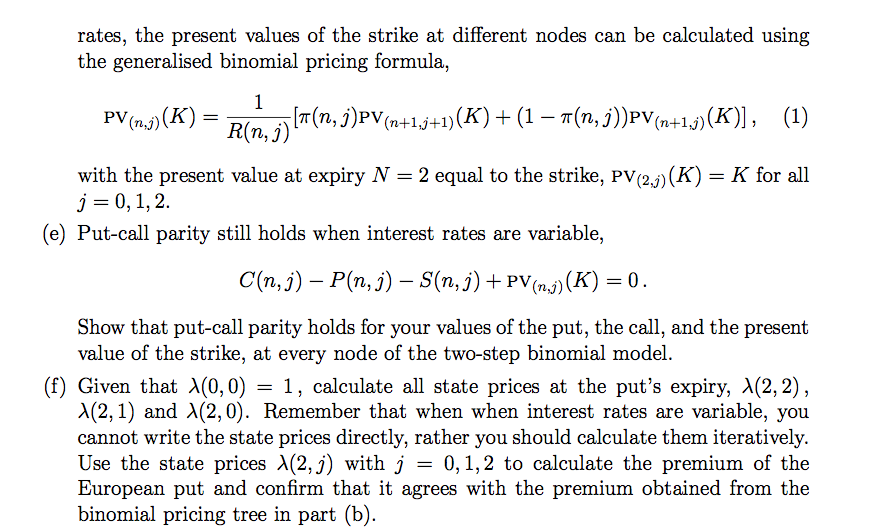
rates, the present values of the strike at different nodes can be calculated using the generalised binomial pricing formula, 1 PV (1,5)(K) = Blo, ()(n,j)PV(n+1.j+1)(K) + (1 7(n, j))PV(n+1,5)(K)], (1) with the present value at expiry N = 2 equal to the strike, PV(2,1)(K) = K for all j=0,1,2. (e) Put-call parity still holds when interest rates are variable, C(n,j) - P(n,j) - Sin,j) + PV(n.)(K) = 0. Show that put-call parity holds for your values of the put, the call, and the present value of the strike, at every node of the two-step binomial model. (f) Given that (0,0) = 1, calculate all state prices at the put's expiry, 1(2, 2), 1(2, 1) and 1(2,0). Remember that when when interest rates are variable, you cannot write the state prices directly, rather you should calculate them iteratively. Use the state prices (2,j) with j = 0,1,2 to calculate the premium of the European put and confirm that it agrees with the premium obtained from the binomial pricing tree in part (b). rates, the present values of the strike at different nodes can be calculated using the generalised binomial pricing formula, 1 PV (1,5)(K) = Blo, ()(n,j)PV(n+1.j+1)(K) + (1 7(n, j))PV(n+1,5)(K)], (1) with the present value at expiry N = 2 equal to the strike, PV(2,1)(K) = K for all j=0,1,2. (e) Put-call parity still holds when interest rates are variable, C(n,j) - P(n,j) - Sin,j) + PV(n.)(K) = 0. Show that put-call parity holds for your values of the put, the call, and the present value of the strike, at every node of the two-step binomial model. (f) Given that (0,0) = 1, calculate all state prices at the put's expiry, 1(2, 2), 1(2, 1) and 1(2,0). Remember that when when interest rates are variable, you cannot write the state prices directly, rather you should calculate them iteratively. Use the state prices (2,j) with j = 0,1,2 to calculate the premium of the European put and confirm that it agrees with the premium obtained from the binomial pricing tree in part (b)