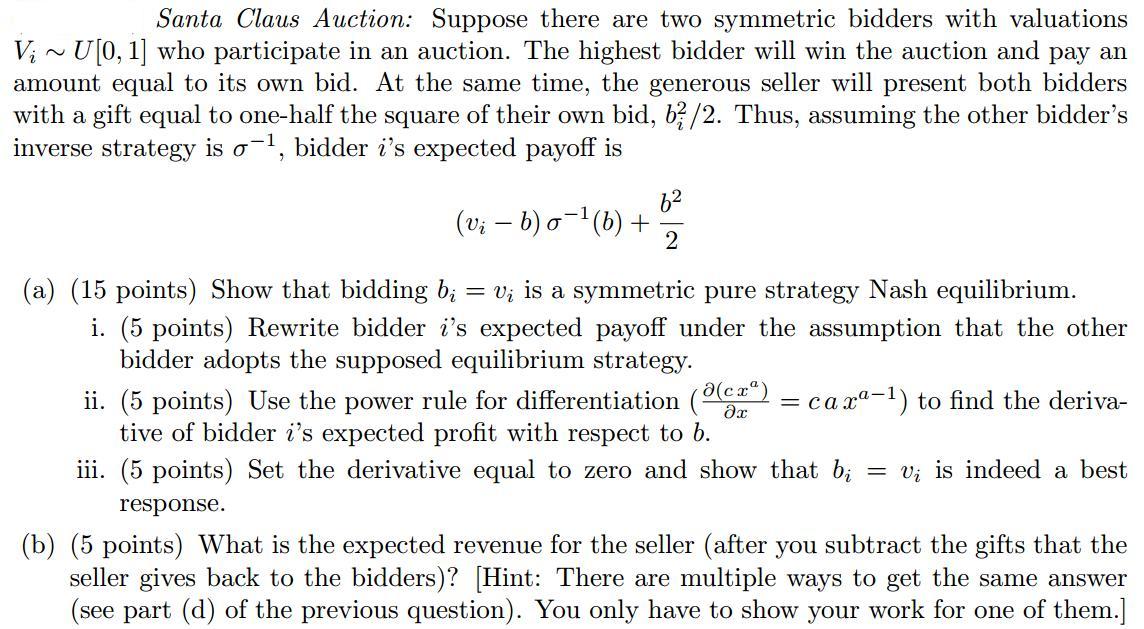
•   

Santa Claus Auction: Suppose there are two symmetric bidders with valuations Vi~ U[0, 1] who participate in an auction. The highest bidder will win the auction and pay an amount equal to its own bid. At the same time, the generous seller will present both bidders with a gift equal to one-half the square of their own bid, b2/2. Thus, assuming the other bidder's inverse strategy is o-, bidder i's expected payoff is 6 (vi- b) o (b) + 2 (a) (15 points) Show that bidding bi = v is a symmetric pure strategy Nash equilibrium. i. (5 points) Rewrite bidder i's expected payoff under the assumption that the other bidder adopts the supposed equilibrium strategy. ii. (5 points) Use the power rule for differentiation ((c) = car-) to find the deriva- tive of bidder i's expected profit with respect to b. iii. (5 points) Set the derivative equal to zero and show that b = v is indeed a best response. (b) (5 points) What is the expected revenue for the seller (after you subtract the gifts that the seller gives back to the bidders)? [Hint: There are multiple ways to get the same answer (see part (d) of the previous question). You only have to show your work for one of them.]