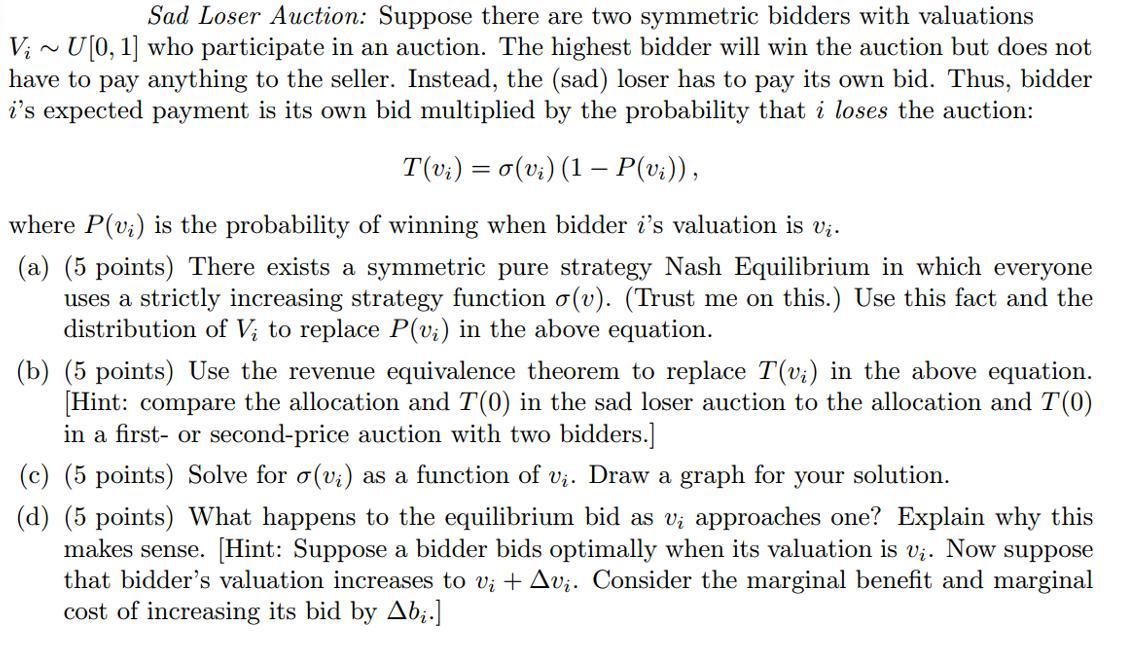
Sad Loser Auction: Suppose there are two symmetric bidders with valuations Vi~ U[0, 1] who participate in an auction. The highest bidder will win the auction but does not have to pay anything to the seller. Instead, the (sad) loser has to pay its own bid. Thus, bidder i's expected payment is its own bid multiplied by the probability that i loses the auction: T(vi) = o(vi) (1 - P(vi)), where P(vi) is the probability of winning when bidder i's valuation is v. (a) (5 points) There exists a symmetric pure strategy Nash Equilibrium in which everyone uses a strictly increasing strategy function o(v). (Trust me on this.) Use this fact and the distribution of V; to replace P(vi) in the above equation. (b) (5 points) Use the revenue equivalence theorem to replace T(vi) in the above equation. [Hint: compare the allocation and T(0) in the sad loser auction to the allocation and T(0) in a first- or second-price auction with two bidders.] (c) (5 points) Solve for o(vi) as a function of v. Draw a graph for your solution. (d) (5 points) What happens to the equilibrium bid as v; approaches one? Explain why this makes sense. [Hint: Suppose a bidder bids optimally when its valuation is v. Now suppose that bidder's valuation increases to vi + Avi. Consider the marginal benefit and marginal cost of increasing its bid by Ab.]