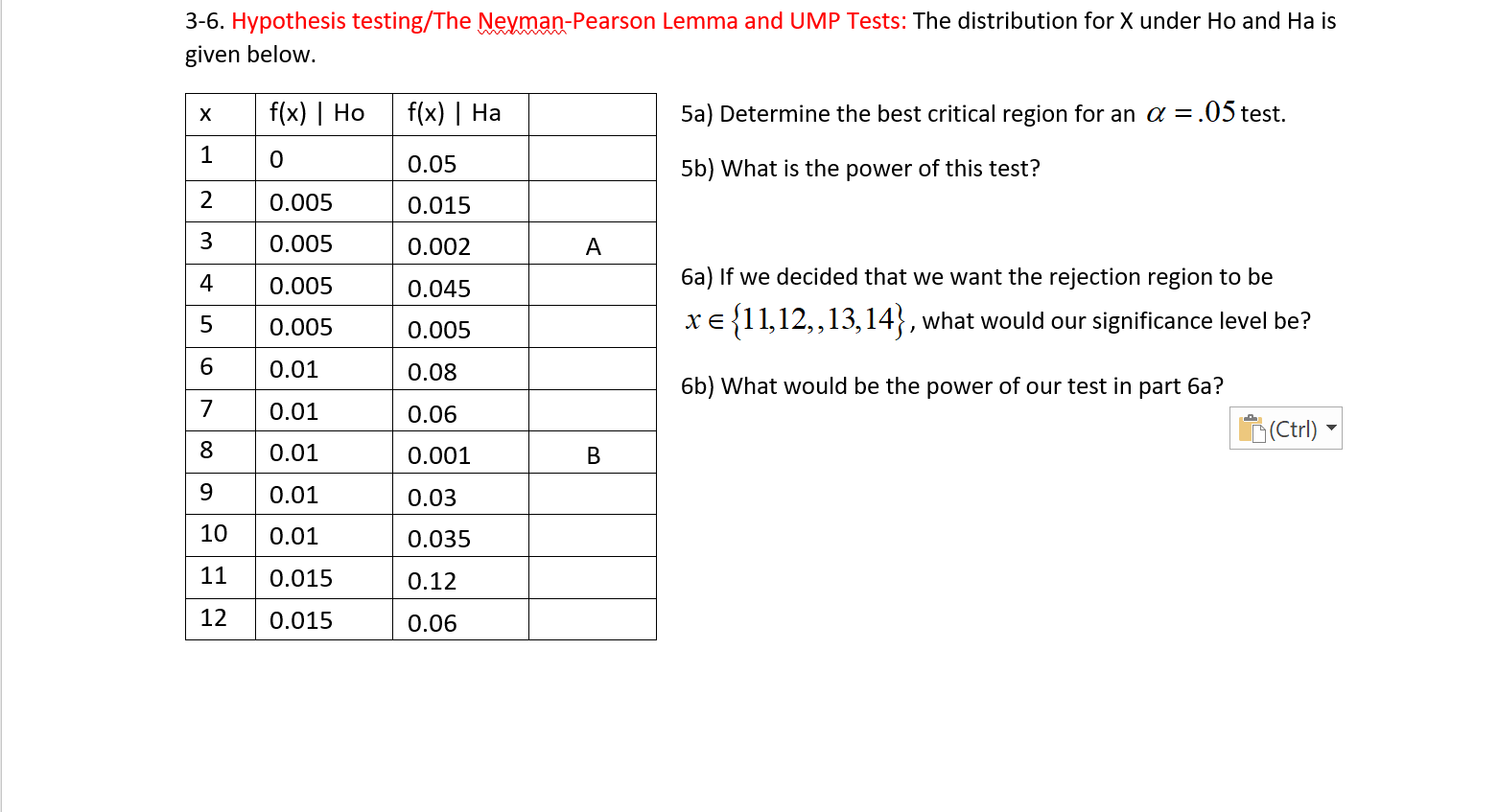
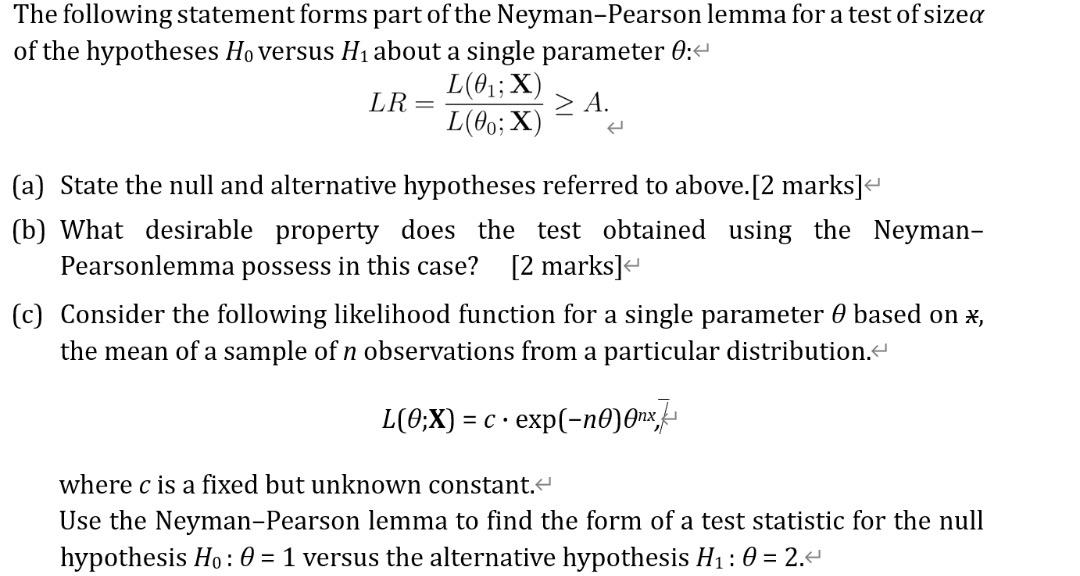
Question
2.1 A cellular call is made to your cell phone at random within a ten-minute interval. You were busy on a call for 2 minutes
2.1 A cellular call is made to your cell phone at random within a ten-minute interval. You were busy on a call for 2 minutes into this ten-minute period. There is a probability that the call arrived when you were not busy on your cell. Identify the random variable and define the distribution of the random variable?
2.2 Complete the below statement.
Identify the distribution of the random variable with the following moment-generating function. ?(?)=e6?+9?2
2.4 A soft drink giant, the Coca-Cola Company produces soft drinks in bulk. The amount of beverages sold to the retail chain stores (such as Pick 'n Pay, Checkers and Spar etc.) in one day can be modelled by a gamma or exponential distribution with ?? = 5 (measurements in litres).
2.4.1 Find the probability that the company will sell more than 5 kilolitres on a given day.
2.4.2 Interpret the answer found in (2.4.1).
2.5 There is relationship between the distribution function of a Beta-distributed random
variable and sums of binomial probabilities. Define this relationship.
2.6 The weekly repair cost ?? for a manufacturing machine has a probability density function given
by
?(?)= 4(1??)3,0?
0,elsewhere
with measurements in hundreds of rands. What type of distribution does Y follow?


Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


