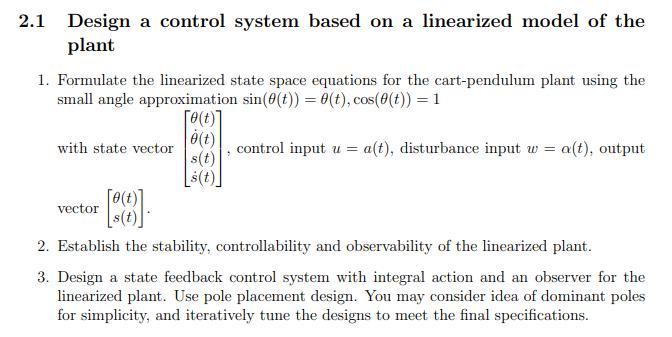
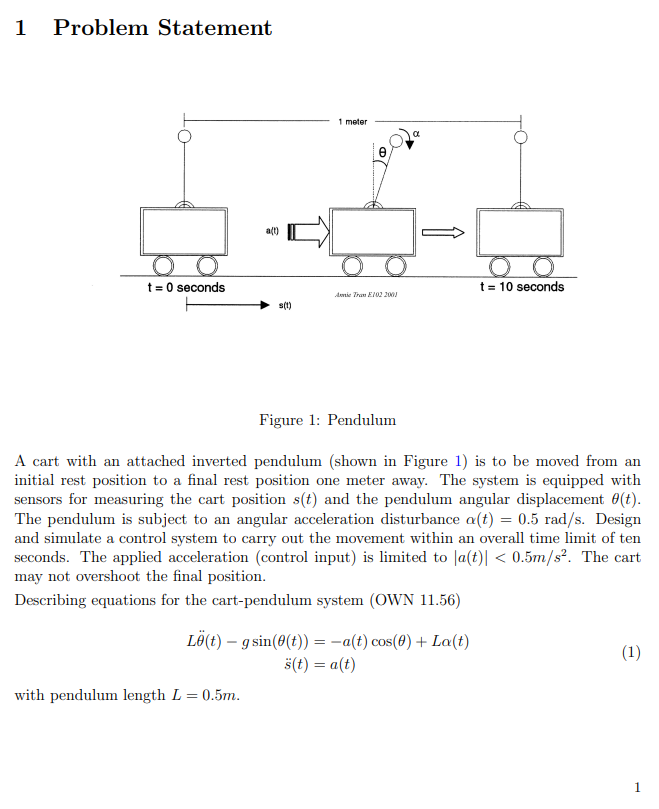
2.1 Design a control system based on a linearized model of the plant 1. Formulate the linearized state space equations for the cart-pendulum plant using the small angle approximation sin(0(t)) = 0(t), cos(0(t)) = 1 with state vector [0(t)] (t) s(t) vector control input u = a(t), disturbance input w = a(t), output [88]. 2. Establish the stability, controllability and observability of the linearized plant. 3. Design a state feedback control system with integral action and an observer for the linearized plant. Use pole placement design. You may consider idea of dominant poles for simplicity, and iteratively tune the designs to meet the final specifications. Problem Statement t = 0 seconds a(t) s(t) with pendulum length L = 0.5m. 1 meter Anne Tran E102 2001 Figure 1: Pendulum A cart with an attached inverted pendulum (shown in Figure 1) is to be moved from an initial rest position to a final rest position one meter away. The system is equipped with sensors for measuring the cart position s(t) and the pendulum angular displacement (t). The pendulum is subject to an angular acceleration disturbance a(t) = 0.5 rad/s. Design and simulate a control system to carry out the movement within an overall time limit of ten seconds. The applied acceleration (control input) is limited to la(t)| < 0.5m/s. The cart may not overshoot the final position. Describing equations for the cart-pendulum system (OWN 11.56) L(t) - g sin(0 (t)) = a(t) cos(9) + La(t) s(t) = a(t) O t = 10 seconds (1) 1