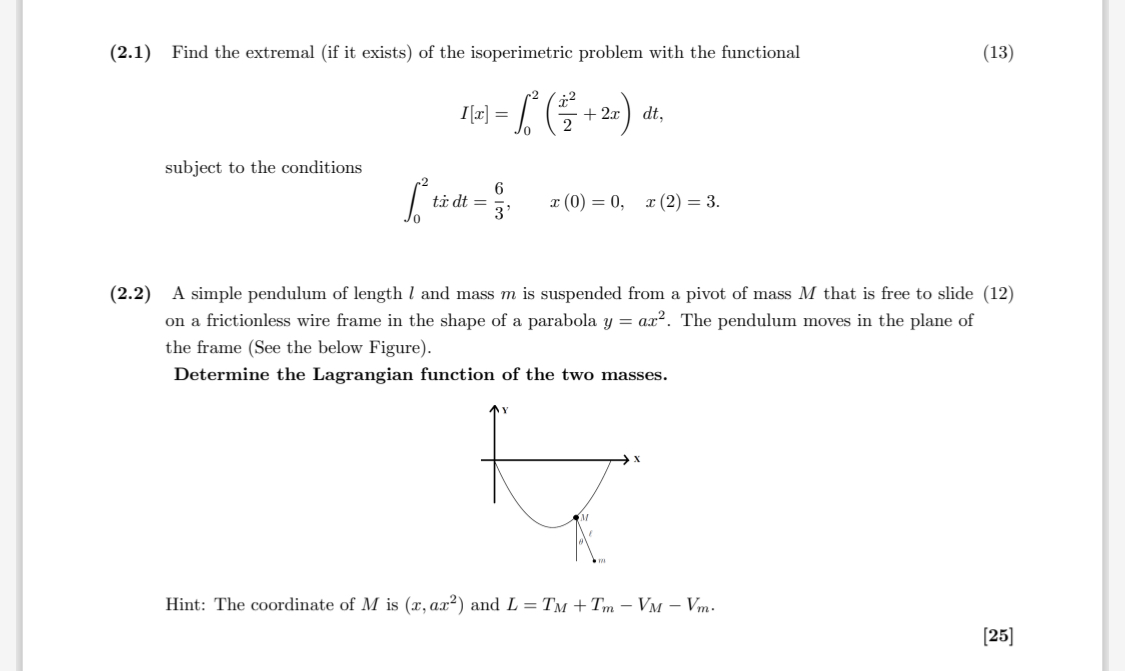
(2.1) Find the extremal (if it exists) of the isoperimetric problem with the functional [x] = (+2r) dt, subject to the conditions tx dt = 6353 x(0) = 0, x (2) = 3. (13) (2.2) A simple pendulum of length l and mass m is suspended from a pivot of mass M that is free to slide (12) on a frictionless wire frame in the shape of a parabola y = ax. The pendulum moves in the plane of the frame (See the below Figure). Determine the Lagrangian function of the two masses. Hint: The coordinate of M is (x, ax) and L = TM +Tm - VM - Vm. [25]