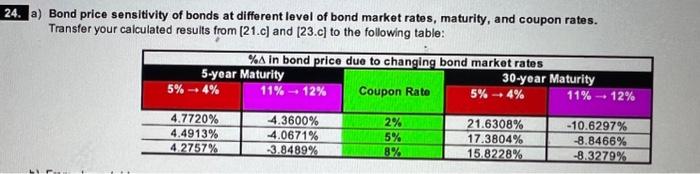
28
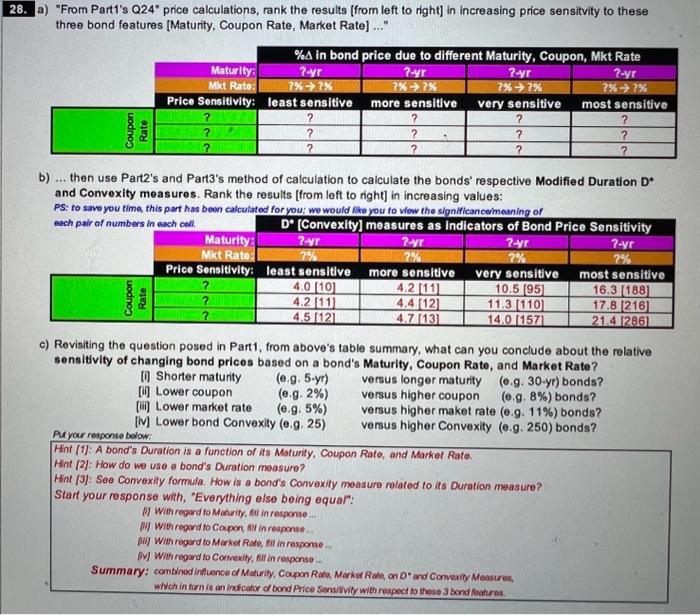
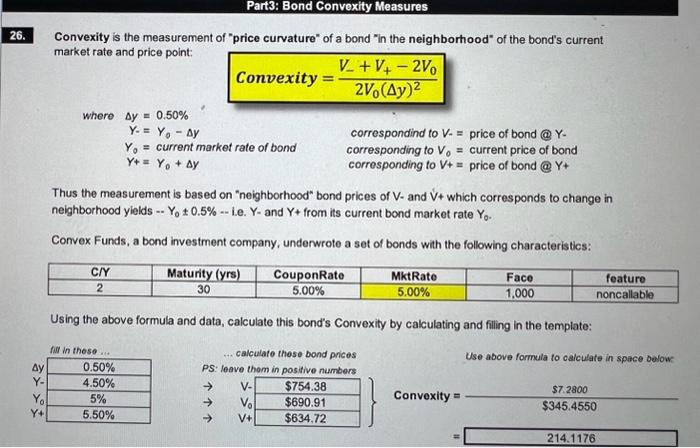
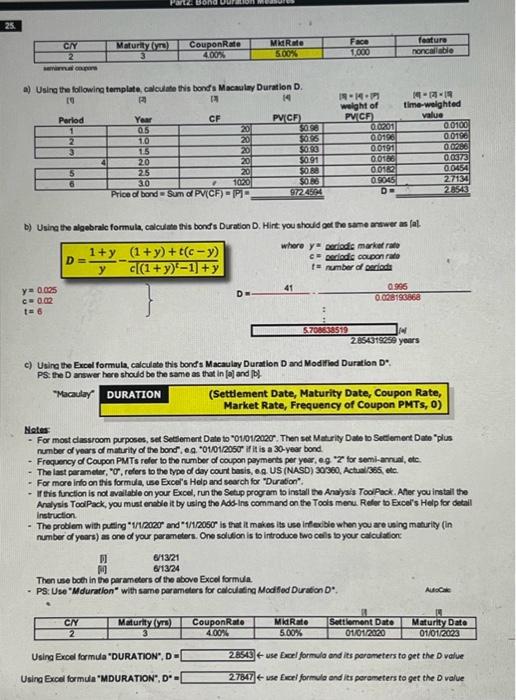
Part3: Bond Convexity Measures Convexity is the measurement of "price curvature" of a bond "in the neighborhood" of the bond's current market rate and price point: Convexity=2V0(y)2V+V+2V0 whereyYY0Y+=0.50%=Y0y=currentmarketrateofbond=Y0+ycorrespondindtoV=priceofbond@YcorrespondingtoV0=currentpriceofbondcorrespondingtoV+=priceofbond@Y+ correspondind to V - = price of bond @ Y - corresponding to V0= current price of bond corresponding to V+=price of bond @ Y+ Thus the measurement is based on "neighborhood" bond prices of V - and V+ which corresponds to change in neighborhood yields -- Y00.5% - i.e. Y - and Y+ from its current bond market rate Y0. Convex Funds, a bond investment company, underwrote a set of bonds with the following characteristics: Using the above formula and data, calculate this bond's Convexity by calculating and filling in the template: fill in thase. calculate these bond prices PS: leave thom in positive numbers. Use above formula to calculate in space bolow Convexity=$345.4550$7.2800 a) Usina the followina temalate calculate this bonds Macaulay Duration D. b) Using the algebrale formula, calculate this bonds Duration D. Hirt you should get the same arsarer as lal. c) Using the Excel formula, calculote this bonds Macaulay Duration D and Modifled Duration D*. PS: the D answer here should be the same as that in [a] and BD. Metes: number of yoars of maturily of the bond, e.q. 0101/2050 if it is a 30-year bond. - Frequency d Coupon PMTs reler io the number of coupon payments per yes, eg " 2 for semi-amul, ete. - For more into on tivis formula, use Excel's Help and search for "Duration". - If this function is not avallatle on your Excel, run the Setup prog am to install the Anaysis Tool Pock. Aher you install the Analysis Tooi Pack, you must enable it by using the Ads-ins command on the Tools menu. Refer be Excel's Help for detail instruction. - The problem with peting "1/12000" and "1/1/2060" is that it makes its use irfexible when you are using mahrity (in number of years) as one of your perameters. One sciltion is to introduce two cells byour calculation: i1 613/21 Then use bot in the parancters of the above Excel formula. - PS: Use "Mduration" with same perameters for calloulatina Modfod Duration D*. Nocik Using Excel formula "DURATION", D = \& use Bacl formula and its porameters to get the D value Usina Excel formula "MDURATION", D*" t use Exal formulo and its porameters to get the 0 value a) Bond price sensitivity of bonds at different level of bond market rates, maturity, and coupon rates. Transfer your calculated results from [21.c] and [23.c] to the following table: a) "From Part1's Q24" price calculations, rank the results [from left to right] in increasing price sensitvity to these three bond features [Maturity, Coupon Rate, Market Rate] ..." b) ... then use Part2's and Part3's method of calculation to calculate the bonds' respective Modified Duration D* and Convexity measures. Rank the results [from left to right] in increasing values: PS: to savo you time, this part has boon calculated for you: wo would ike vou fo viow the sianificancwimanina of c) Revisiting the question posed in Part1, from above's table summary, what can you conclude about the relative sensitivity of changing bond prices based on a bond's Maturity, Coupon Rate, and Market Rate? [i] Shorter maturity [ii] Lower coupon [iii] Lower market rate (e.g. 5-yr) versus longer maturity (e.g. 30-yr) bonds? (e.g. 2%) versus higher coupon (e.g. 8% ) bonds? (.9.5%) versus higher maket rate (e.g. 11\%) bonds? [iv] Lower bond Convexity (e.g. 25) versus higher Convexity (e.g. 250) bonds? Pu your responso below: Hint (1): A bond's Duration is a function of its Matunity, Coupon Rate, and Market Rate. Hint (2): How do we use a bond's Duration moasure? Hint (3): See Convexity formula. How is a bond's Convexity measure related to its Duration measure? Start your response with, "Everything else being equal": (1) With regard to Maturity, fil in responso. (ii) With regard to Coupon fill in response. (iii) With rogard to Market Rate, fill in response ... [V] With rogerd to Comexity, fill in response Summary: comblinodinfuance of Matirity, Capon Rato, Marker Rath, on D* and Convexily Measures, wheh in turn it an indicator al bond Price Sonsitivity with respoct to these 3 bond fleaturos. Part3: Bond Convexity Measures Convexity is the measurement of "price curvature" of a bond "in the neighborhood" of the bond's current market rate and price point: Convexity=2V0(y)2V+V+2V0 whereyYY0Y+=0.50%=Y0y=currentmarketrateofbond=Y0+ycorrespondindtoV=priceofbond@YcorrespondingtoV0=currentpriceofbondcorrespondingtoV+=priceofbond@Y+ correspondind to V - = price of bond @ Y - corresponding to V0= current price of bond corresponding to V+=price of bond @ Y+ Thus the measurement is based on "neighborhood" bond prices of V - and V+ which corresponds to change in neighborhood yields -- Y00.5% - i.e. Y - and Y+ from its current bond market rate Y0. Convex Funds, a bond investment company, underwrote a set of bonds with the following characteristics: Using the above formula and data, calculate this bond's Convexity by calculating and filling in the template: fill in thase. calculate these bond prices PS: leave thom in positive numbers. Use above formula to calculate in space bolow Convexity=$345.4550$7.2800 a) Usina the followina temalate calculate this bonds Macaulay Duration D. b) Using the algebrale formula, calculate this bonds Duration D. Hirt you should get the same arsarer as lal. c) Using the Excel formula, calculote this bonds Macaulay Duration D and Modifled Duration D*. PS: the D answer here should be the same as that in [a] and BD. Metes: number of yoars of maturily of the bond, e.q. 0101/2050 if it is a 30-year bond. - Frequency d Coupon PMTs reler io the number of coupon payments per yes, eg " 2 for semi-amul, ete. - For more into on tivis formula, use Excel's Help and search for "Duration". - If this function is not avallatle on your Excel, run the Setup prog am to install the Anaysis Tool Pock. Aher you install the Analysis Tooi Pack, you must enable it by using the Ads-ins command on the Tools menu. Refer be Excel's Help for detail instruction. - The problem with peting "1/12000" and "1/1/2060" is that it makes its use irfexible when you are using mahrity (in number of years) as one of your perameters. One sciltion is to introduce two cells byour calculation: i1 613/21 Then use bot in the parancters of the above Excel formula. - PS: Use "Mduration" with same perameters for calloulatina Modfod Duration D*. Nocik Using Excel formula "DURATION", D = \& use Bacl formula and its porameters to get the D value Usina Excel formula "MDURATION", D*" t use Exal formulo and its porameters to get the 0 value a) Bond price sensitivity of bonds at different level of bond market rates, maturity, and coupon rates. Transfer your calculated results from [21.c] and [23.c] to the following table: a) "From Part1's Q24" price calculations, rank the results [from left to right] in increasing price sensitvity to these three bond features [Maturity, Coupon Rate, Market Rate] ..." b) ... then use Part2's and Part3's method of calculation to calculate the bonds' respective Modified Duration D* and Convexity measures. Rank the results [from left to right] in increasing values: PS: to savo you time, this part has boon calculated for you: wo would ike vou fo viow the sianificancwimanina of c) Revisiting the question posed in Part1, from above's table summary, what can you conclude about the relative sensitivity of changing bond prices based on a bond's Maturity, Coupon Rate, and Market Rate? [i] Shorter maturity [ii] Lower coupon [iii] Lower market rate (e.g. 5-yr) versus longer maturity (e.g. 30-yr) bonds? (e.g. 2%) versus higher coupon (e.g. 8% ) bonds? (.9.5%) versus higher maket rate (e.g. 11\%) bonds? [iv] Lower bond Convexity (e.g. 25) versus higher Convexity (e.g. 250) bonds? Pu your responso below: Hint (1): A bond's Duration is a function of its Matunity, Coupon Rate, and Market Rate. Hint (2): How do we use a bond's Duration moasure? Hint (3): See Convexity formula. How is a bond's Convexity measure related to its Duration measure? Start your response with, "Everything else being equal": (1) With regard to Maturity, fil in responso. (ii) With regard to Coupon fill in response. (iii) With rogard to Market Rate, fill in response ... [V] With rogerd to Comexity, fill in response Summary: comblinodinfuance of Matirity, Capon Rato, Marker Rath, on D* and Convexily Measures, wheh in turn it an indicator al bond Price Sonsitivity with respoct to these 3 bond fleaturos