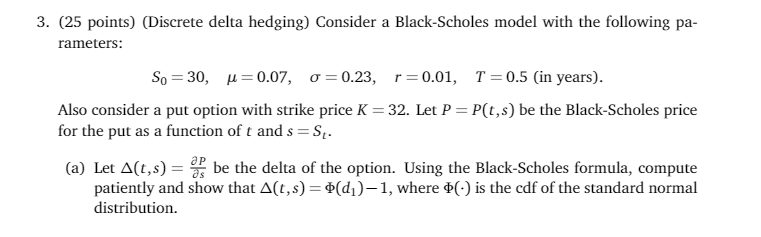
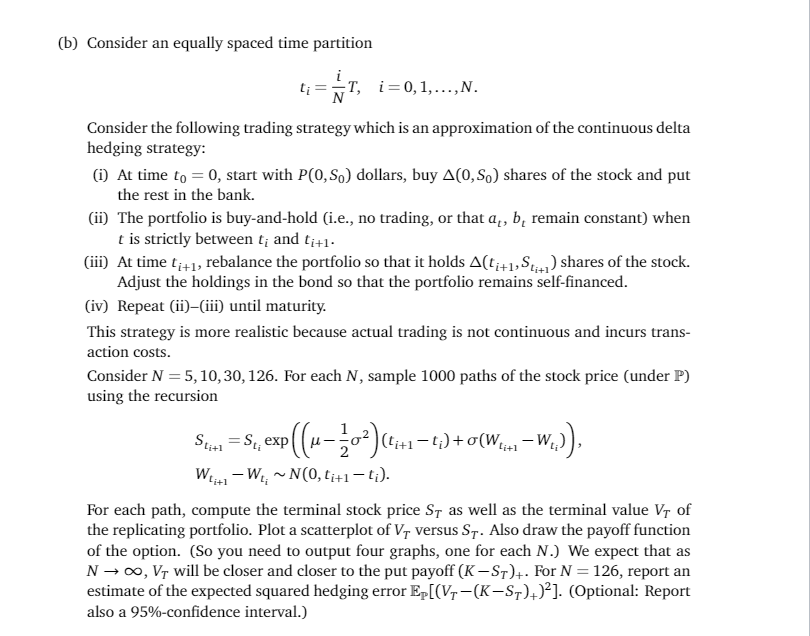
3. (25 points) (Discrete delta hedging) Consider a Black-Scholes model with the following pa- rameters: So = 30, u=0.07, o=0.23, r=0.01, T=0.5 (in years). Also consider a put option with strike price K = 32. Let P=P(t,s) be the Black-Scholes price for the put as a function of t and s=St. (a) Let At,s) = be the delta of the option. Using the Black-Scholes formula, compute patiently and show that Alt,s) = (d)-1, where $() is the cdf of the standard normal distribution. (b) Consider an equally spaced time partition it, i=0,1,...,N. Consider the following trading strategy which is an approximation of the continuous delta hedging strategy: (i) At time to = 0, start with P(0,So) dollars, buy A(0,So) shares of the stock and put the rest in the bank. (ii) The portfolio is buy-and-hold (i.e., no trading, or that a, b, remain constant) when t is strictly between t and ti+1. (iii) At time ti+1, rebalance the portfolio so that it holds Ati+1, Stith) shares of the stock. Adjust the holdings in the bond so that the portfolio remains self-financed. (iv) Repeat (ii)-(iii) until maturity. This strategy is more realistic because actual trading is not continuous and incurs trans- action costs. Consider N =5, 10, 30, 126. For each N, sample 1000 paths of the stock price (under P) using the recursion Scion =S, exp((--02) lit1 4)+0(W..-W.)) Wi+1- WN(0, ti+1 -1;). For each path, compute the terminal stock price St as well as the terminal value V1 of the replicating portfolio. Plot a scatterplot of V, versus St. Also draw the payoff function of the option. (So you need to output four graphs, one for each N.) We expect that as NO, V will be closer and closer to the put payoff (K-ST)+. For N = 126, report an estimate of the expected squared hedging error Ep[(V;-(K-ST)+)?]. (Optional: Report also a 95%-confidence interval.) 3. (25 points) (Discrete delta hedging) Consider a Black-Scholes model with the following pa- rameters: So = 30, u=0.07, o=0.23, r=0.01, T=0.5 (in years). Also consider a put option with strike price K = 32. Let P=P(t,s) be the Black-Scholes price for the put as a function of t and s=St. (a) Let At,s) = be the delta of the option. Using the Black-Scholes formula, compute patiently and show that Alt,s) = (d)-1, where $() is the cdf of the standard normal distribution. (b) Consider an equally spaced time partition it, i=0,1,...,N. Consider the following trading strategy which is an approximation of the continuous delta hedging strategy: (i) At time to = 0, start with P(0,So) dollars, buy A(0,So) shares of the stock and put the rest in the bank. (ii) The portfolio is buy-and-hold (i.e., no trading, or that a, b, remain constant) when t is strictly between t and ti+1. (iii) At time ti+1, rebalance the portfolio so that it holds Ati+1, Stith) shares of the stock. Adjust the holdings in the bond so that the portfolio remains self-financed. (iv) Repeat (ii)-(iii) until maturity. This strategy is more realistic because actual trading is not continuous and incurs trans- action costs. Consider N =5, 10, 30, 126. For each N, sample 1000 paths of the stock price (under P) using the recursion Scion =S, exp((--02) lit1 4)+0(W..-W.)) Wi+1- WN(0, ti+1 -1;). For each path, compute the terminal stock price St as well as the terminal value V1 of the replicating portfolio. Plot a scatterplot of V, versus St. Also draw the payoff function of the option. (So you need to output four graphs, one for each N.) We expect that as NO, V will be closer and closer to the put payoff (K-ST)+. For N = 126, report an estimate of the expected squared hedging error Ep[(V;-(K-ST)+)?]. (Optional: Report also a 95%-confidence interval.)








