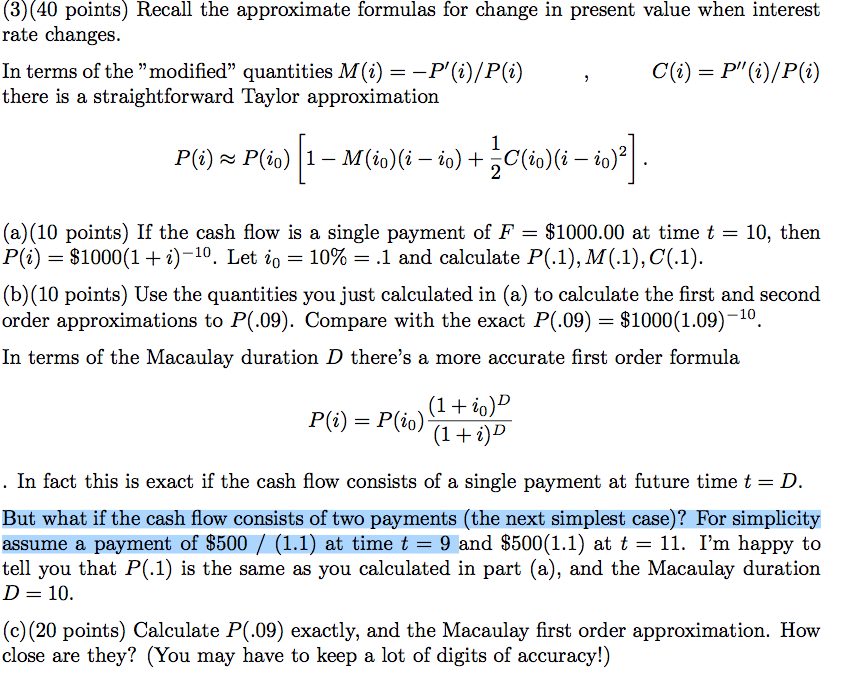
(3) (40 points) Recall the approximate formulas for change in present value when interest rate changes. In terms of the "modified" quantities M(i)- -P'(i)/P(i),C(i) - P(i)/P(i) there is a straightforward Taylor approximation (a)(10 points) If the cash flow is a single payment of F $1000.00 at timet 10, then Pli) = $1000(1 + i)-10. Let io = 10% = .1 and calculate P(1), M(1), C(1) (b)(10 points) Use the quantities you just calculated in (a) to calculate the first and second order approximations to P(.09). Compare with the exact P(.09) $1000 (1.09)-10. In terms of the Macaulay duration D there's a more accurate first order formula . In fact this is exact if the cash flow consists of a single payment at future time t- D But what if the cash flow consists of two payments (the next simplest case)? For simplicity assume a payment of S500/(1.1) at timet 9 and 8500 (1.1) at t 11. I'm happy to tell you that P(.1) is the same as you calculated in part (a), and the Macaulay duration D 10 (c) (20 points) Calculate P(.09) exactly, and the Macaulay first order approximation. How close are they? (You may have to keep a lot of digits of accuracy!) (3) (40 points) Recall the approximate formulas for change in present value when interest rate changes. In terms of the "modified" quantities M(i)- -P'(i)/P(i),C(i) - P(i)/P(i) there is a straightforward Taylor approximation (a)(10 points) If the cash flow is a single payment of F $1000.00 at timet 10, then Pli) = $1000(1 + i)-10. Let io = 10% = .1 and calculate P(1), M(1), C(1) (b)(10 points) Use the quantities you just calculated in (a) to calculate the first and second order approximations to P(.09). Compare with the exact P(.09) $1000 (1.09)-10. In terms of the Macaulay duration D there's a more accurate first order formula . In fact this is exact if the cash flow consists of a single payment at future time t- D But what if the cash flow consists of two payments (the next simplest case)? For simplicity assume a payment of S500/(1.1) at timet 9 and 8500 (1.1) at t 11. I'm happy to tell you that P(.1) is the same as you calculated in part (a), and the Macaulay duration D 10 (c) (20 points) Calculate P(.09) exactly, and the Macaulay first order approximation. How close are they? (You may have to keep a lot of digits of accuracy!)