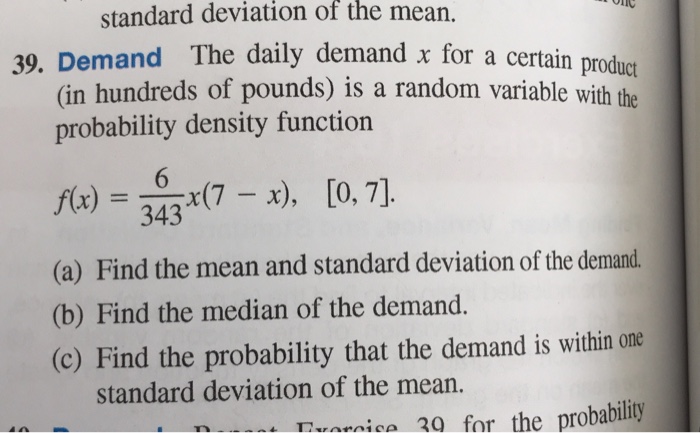
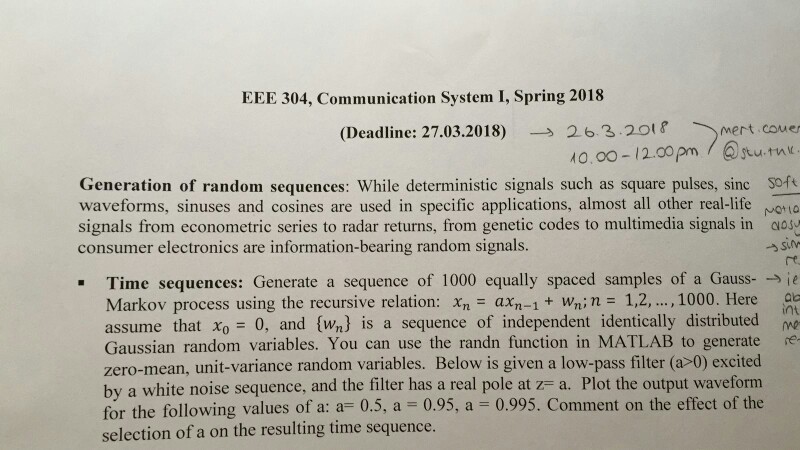
Question
3. Buses arrive to a bus stop according to an exponential distribution with rate ?= 4 busses/hour. If you arrived at 8:00 am to the
3. Buses arrive to a bus stop according to an exponential distribution with rate
?= 4 busses/hour. If you arrived at 8:00 am to the bus stop,
a) what is the expected time of the next bus?
b) Assume you asked one of the people waiting for the bus about the arrival
time of the last bus and he told you that the last bus left at 7:40 am. What
is the expected time of the next bus?
4. Break downs occur on an old car with rate ?= 5 break-downs/month. The owner
of the car is planning to have a trip on his car for 4 days.
a) What is the probability that he will return home safely on his car.
b) If the car broke down the second day of the trip and the car was fixed, what is
the probability that he doesn't return home safely on his car.
5. Suppose that the amount of time one spends in a bank is exponentially distributed with
mean 10 minutes. What is the probability that a customer will spend more than 15
minutes in the bank? What is the probability that a customer will spend more than 15
minutes in the bank given that he is still in the bank after 10 minutes?
6. Suppose the lifespan in hundreds of hours, T, of a light bulb of a home lamp is
exponentially distributed with lambda = 0.2. compute the probability that the light bulb
will last more than 700 hours Also, the probability that the light bulb will last more than
900 hours
7. Let X = amount of time (in minutes) a postal clerk spends with his/her customer. The
time is known to have an exponential distribution with the average amount of time equal
to 4 minutes.
a) Find the probability that a clerk spends four to five minutes with a randomly selected
customer.
b) Half of all customers are finished within how long? (Find median)
c) Which is larger, the mean or the median?
8. On the average, a certain computer part lasts 10 years. The length of time the computer
part lasts is exponentially distributed.
a) What is the probability that a computer part lasts more than 7 years?
b) On the average, how long would 5 computer parts last if they are used one after
another?
c) Eighty percent of computer parts last at most how long?
d) What is the probability that a computer part lasts between 9 and 11 years?
9. Suppose that the length of a phone call, in minutes, is an exponential random variable
with decay parameter = 1/12 . If another person arrives at a public telephone just before
you, find the probability that you will have to wait more than 5 minutes. Let X = the
length of a phone call, in minutes. What is median mean and standard deviation of X?
1. Calculate the Poisson distribution whose ? (Average Rate of Success)) is 3 & X (Poisson
Random Variable) is 6.
2. Customers arrive at a checkout counter according to a Poisson distribution at an average
of 7 per hour. During a given hour, what are the probabilities that
a) No more than 3 customers arrive?
b) At least 2 customers arrive?
c) Exactly 5 customers arrive?
3. Manufacturer of television set knows that on an average 5% of their product is defective.
They sells television sets in consignment of 100 and guarantees that not more than 2 set
will be defective. What is the probability that the TV set will fail to meet the guaranteed
quality?
4. It is known from the past experience that in a certain plant there are on the average of 4
industrial accidents per month. Find the probability that in a given year will be less that 3
accidents.
5. Suppose that the change of an individual coal miner being killed in a mining accident
during a year is 1.1499. Use the Poisson distribution to calculate the probability that in
the mine employing 350 miners- there will be at least one accident in a year.
6. The number of road construction projects that take place at any one time in a certain city
follows a Poisson distribution with a mean of 3. Find the probability that exactly five road
construction projects are currently taking place in this city. (0.100819)
7. The number of road construction projects that take place at any one time in a certain city
follows a Poisson distribution with a mean of 7. Find the probability that more than four
road construction projects are currently taking place in the city. (0.827008)


Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


