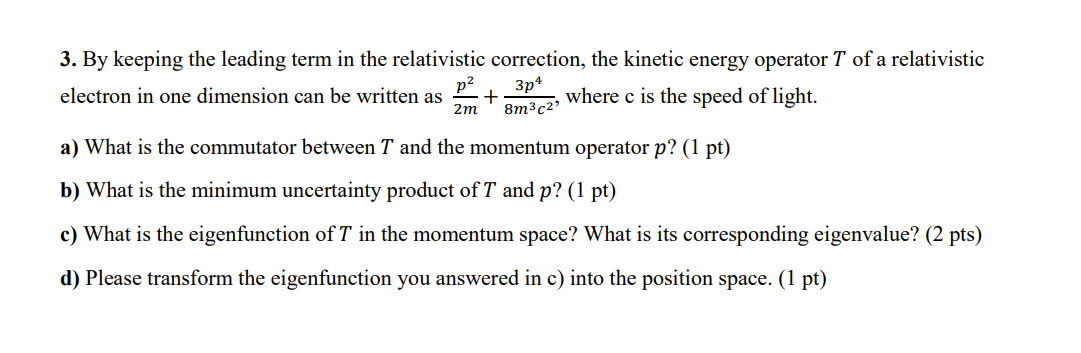
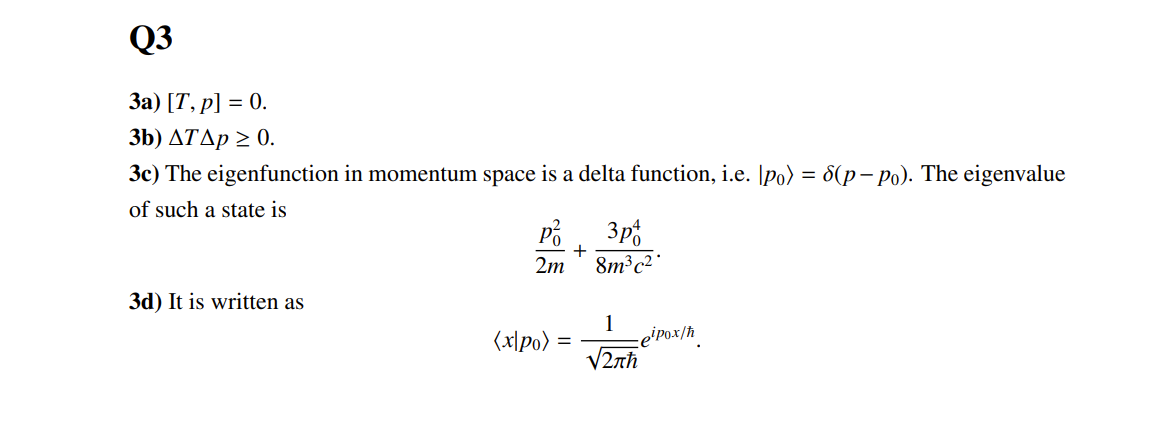
3. By keeping the leading term in the relativistic correction, the kinetic energy operator T of a relativistic electron in one dimension can be written as p 3p4 + 2m 8m3c2 where c is the speed of light. a) What is the commutator between T and the momentum operator p? (1 pt) b) What is the minimum uncertainty product of T and p? (1 pt) c) What is the eigenfunction of T in the momentum space? What is its corresponding eigenvalue? (2 pts) d) Please transform the eigenfunction you answered in c) into the position space. (1 pt) Q3 3a) [T, p] = 0. 3b) ATAP 0. 3c) The eigenfunction in momentum space is a delta function, i.e. [po) = 8(p-po). The eigenvalue of such a state is P + 2m 8m3c2* 3d) It is written as 1 (x|po) = eipox/ 2h