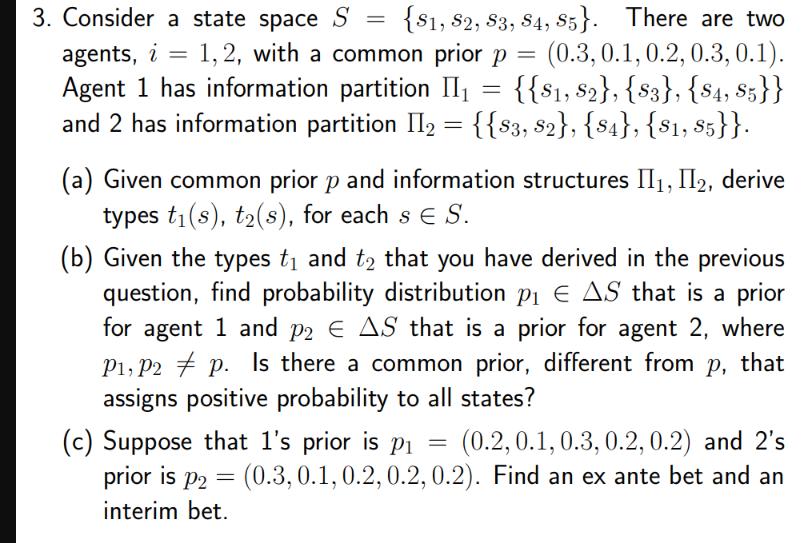
3. Consider a state space S {$1, S2, S3, S4, S5}. There are two agents, i = 1,2, with a common prior p = (0.3, 0.1,0.2, 0.3, 0.1). Agent 1 has information partition II = {{$, $2}, {$3}, {$4, $5}} and 2 has information partition II2 = {{$3, $2}, {$4}, {81, 85}}. = (a) Given common prior p and information structures II, I2, derive types t(s), t(s), for each s S. (b) Given the types t and to that you have derived in the previous question, find probability distribution p AS that is a prior for agent 1 and P2 EAS that is a prior for agent 2, where P1, P2P. Is there a common prior, different from P, that assigns positive probability to all states? (c) Suppose that 1's prior is p = (0.2, 0.1, 0.3, 0.2,0.2) and 2's prior is p2 (0.3, 0.1, 0.2, 0.2, 0.2). Find an ex ante bet and an interim bet. =