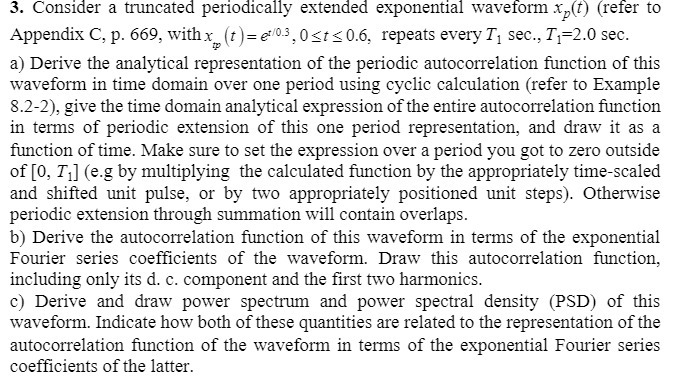
3. Consider a truncated periodically extended exponential waveform xp(t) (refer to Appendix C, p. 669, with x (t)= 0.3, 0t0.6, repeats every T sec., T=2.0 sec. a) Derive the analytical representation of the periodic autocorrelation function of this waveform in time domain over one period using cyclic calculation (refer to Example 8.2-2), give the time domain analytical expression of the entire autocorrelation function in terms of periodic extension of this one period representation, and draw it as a function of time. Make sure to set the expression over a period you got to zero outside of [0, T] (e.g by multiplying the calculated function by the appropriately time-scaled and shifted unit pulse, or by two appropriately positioned unit steps). Otherwise periodic extension through summation will contain overlaps. b) Derive the autocorrelation function of this waveform in terms of the exponential Fourier series coefficients of the waveform. Draw this autocorrelation function, including only its d. c. component and the first two harmonics. c) Derive and draw power spectrum and power spectral density (PSD) of this waveform. Indicate how both of these quantities are related to the representation of the autocorrelation function of the waveform in terms of the exponential Fourier series coefficients of the latter.