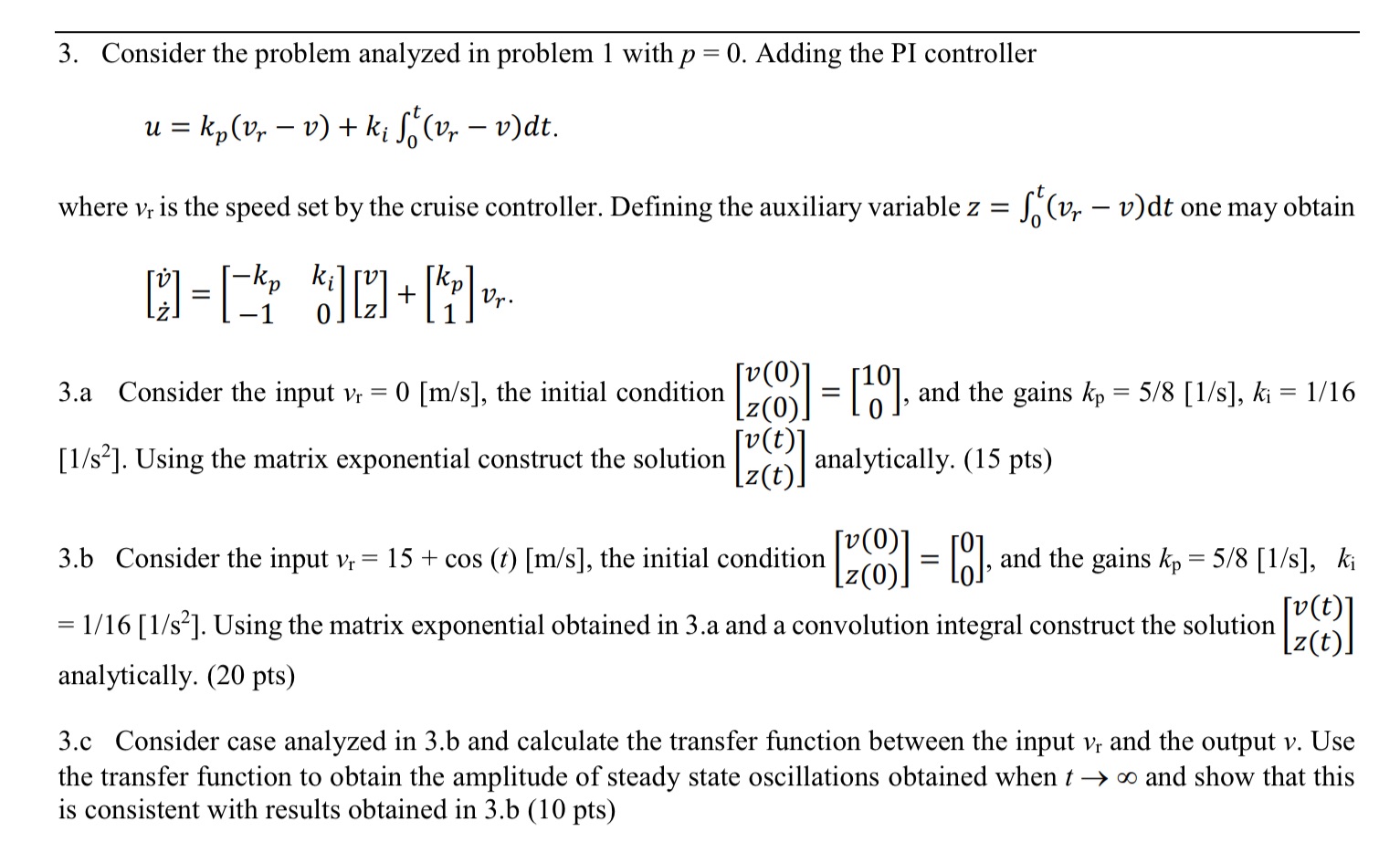
3. Consider the problem analyzed in problem 1 with p = 0. Adding the PI controller - u = kp (vr v) + ki (vr v)dt. - where vr is the speed set by the cruise controller. Defining the auxiliary variable z = (vv)dt one may obtain [1] = -kp + Vr. 3.a Consider the input vr = 0 [m/s], the initial condition [1/2]. Using the matrix exponential construct the solution [128] = [19] analytically. (15 pts) and the gains kp = 5/8 [1/s], ki = 1/16 3.b Consider the input vr = 15+ cos (t) [m/s], the initial condition = and the gains kp = 5/8 [1/s], ki [2] = 1/16 [1/s]. Using the matrix exponential obtained in 3.a and a convolution integral construct the solution analytically. (20 pts) 3.c Consider case analyzed in 3.b and calculate the transfer function between the input vr and the output v. Use the transfer function to obtain the amplitude of steady state oscillations obtained when t and show that this is consistent with results obtained in 3.b (10 pts)