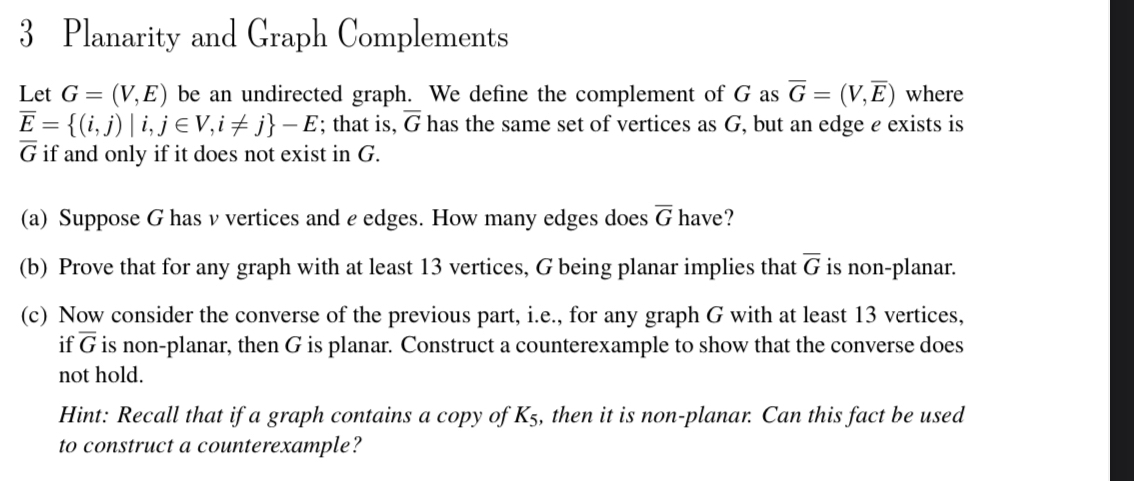
$3$ Planarity and Graph Complements

Let $G=(V,E)$ be an undirected graph. We define the complement of $G$ as $\frac{?}{\text{b}\text{a}\text{r}}(G)=(V\frac{,}{b}ar(E))$ where $\frac{?}{\text{b}\text{a}\text{r}}(E)=\{(i,j)|i,$jinV,$ij\}-E$; that is$,\frac{?}{\text{b}\text{a}\text{r}}(G)$ has the same set of vertices as $G,$ but an edge $e$ exists is $\frac{?}{\text{b}\text{a}\text{r}}(G)$ if and only if it does not exist in $G.$

$($a$)$ Suppose $G$ has $v$ vertices and $e$ edges. How many edges does $\frac{?}{\text{b}\text{a}\text{r}}(G)$ have?

$($b$)$ Prove that for any graph with at least $13$ vertices, $G$ being planar implies that $\frac{?}{\text{b}\text{a}\text{r}}(G)$ is non$-$planar.

$($c$)$ Now consider the converse of the previous part, i$.$e$.,$ for any graph $G$ with at least $13$ vertices, if $\frac{?}{\text{b}\text{a}\text{r}}(G)$ is non$-$planar, then $G$ is planar. Construct a counterexample to show that the converse does not hold.

Hint: Recall that if a graph contains a copy of $K_5,$ then it is non$-$planar. Can this fact be used to construct a counterexample?