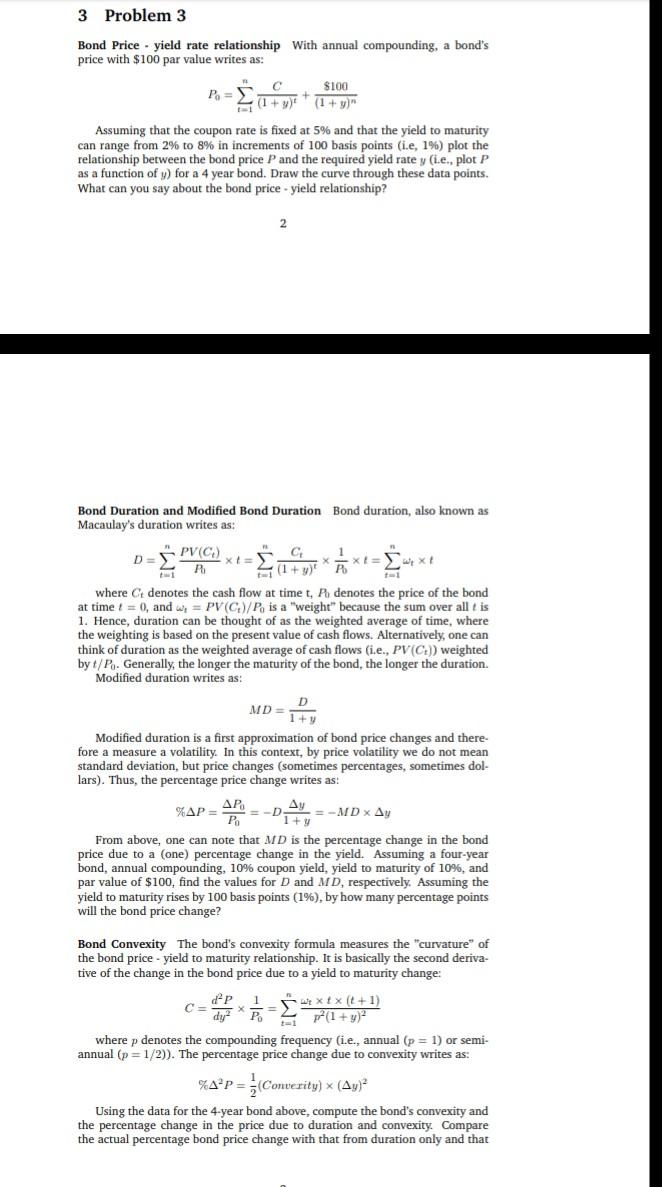
3 Problem 3 Bond Price - yield rate relationship With annual compounding, a bond's price with $100 par value writes as: $100 P = (1+y)(1+y)" Assuming that the coupon rate is fixed at 5% and that the yield to maturity can range from 2% to 8% in increments of 100 basis points (ie, 1%) plot the relationship between the bond price and the required yield rate y (i.e., plot P as a function of y) for a 4 year bond. Draw the curve through these data points. What can you say about the bond price - yield relationship? 2 Bond Duration and Modified Bond Duration Bond duration, also known as Macaulay's duration writes as: PV(C) C 1 D x XE X (1+y) R where Ct denotes the cash flow at time t, P denotes the price of the bond at time I = 0, and we = PV(C)/P, is a "weight" because the sum over allt is 1. Hence, duration can be thought of as the weighted average of time, where the weighting is based on the present value of cash flows. Alternatively, one can think of duration as the weighted average of cash flows (i.e., PV(C:)) weighted by t/P. Generally, the longer the maturity of the bond, the longer the duration Modified duration writes as: MD= Modified duration is a first approximation of bond price changes and there- fore a measure a volatility. In this context, by price volatility we do not mean standard deviation, but price changes (sometimes percentages, sometimes dol- lars). Thus, the percentage price change writes as: = =-D Ay =-MD x Ay 1 + From above, one can note that MD is the percentage change in the bond price due to a (one) percentage change in the yield. Assuming a four-year bond, annual compounding, 10% coupon yield, yield to maturity of 10%, and par value of $100, find the values for D and MD, respectively. Assuming the yield to maturity rises by 100 basis points (1%), by how many percentage points will the bond price change? %AP = Bond Convexity The bond's convexity formula measures the "curvature of the bond price - yield to maturity relationship. It is basically the second deriva- tive of the change in the bond price due to a yield to maturity change: AP 1 CE uxtx (2+1) dy? Po (1 + y)2 where p denotes the compounding frequency (i.e., annual (p = 1) or semi- annual (p=1/2)). The percentage price change due to convexity writes as: %A?p= }(Converity) x (Ay) Using the data for the 4-year bond above, compute the bond's convexity and the percentage change in the price due to duration and convexity. Compare the actual percentage bond price change with that from duration only and that 3 Problem 3 Bond Price - yield rate relationship With annual compounding, a bond's price with $100 par value writes as: $100 P = (1+y)(1+y)" Assuming that the coupon rate is fixed at 5% and that the yield to maturity can range from 2% to 8% in increments of 100 basis points (ie, 1%) plot the relationship between the bond price and the required yield rate y (i.e., plot P as a function of y) for a 4 year bond. Draw the curve through these data points. What can you say about the bond price - yield relationship? 2 Bond Duration and Modified Bond Duration Bond duration, also known as Macaulay's duration writes as: PV(C) C 1 D x XE X (1+y) R where Ct denotes the cash flow at time t, P denotes the price of the bond at time I = 0, and we = PV(C)/P, is a "weight" because the sum over allt is 1. Hence, duration can be thought of as the weighted average of time, where the weighting is based on the present value of cash flows. Alternatively, one can think of duration as the weighted average of cash flows (i.e., PV(C:)) weighted by t/P. Generally, the longer the maturity of the bond, the longer the duration Modified duration writes as: MD= Modified duration is a first approximation of bond price changes and there- fore a measure a volatility. In this context, by price volatility we do not mean standard deviation, but price changes (sometimes percentages, sometimes dol- lars). Thus, the percentage price change writes as: = =-D Ay =-MD x Ay 1 + From above, one can note that MD is the percentage change in the bond price due to a (one) percentage change in the yield. Assuming a four-year bond, annual compounding, 10% coupon yield, yield to maturity of 10%, and par value of $100, find the values for D and MD, respectively. Assuming the yield to maturity rises by 100 basis points (1%), by how many percentage points will the bond price change? %AP = Bond Convexity The bond's convexity formula measures the "curvature of the bond price - yield to maturity relationship. It is basically the second deriva- tive of the change in the bond price due to a yield to maturity change: AP 1 CE uxtx (2+1) dy? Po (1 + y)2 where p denotes the compounding frequency (i.e., annual (p = 1) or semi- annual (p=1/2)). The percentage price change due to convexity writes as: %A?p= }(Converity) x (Ay) Using the data for the 4-year bond above, compute the bond's convexity and the percentage change in the price due to duration and convexity. Compare the actual percentage bond price change with that from duration only and that