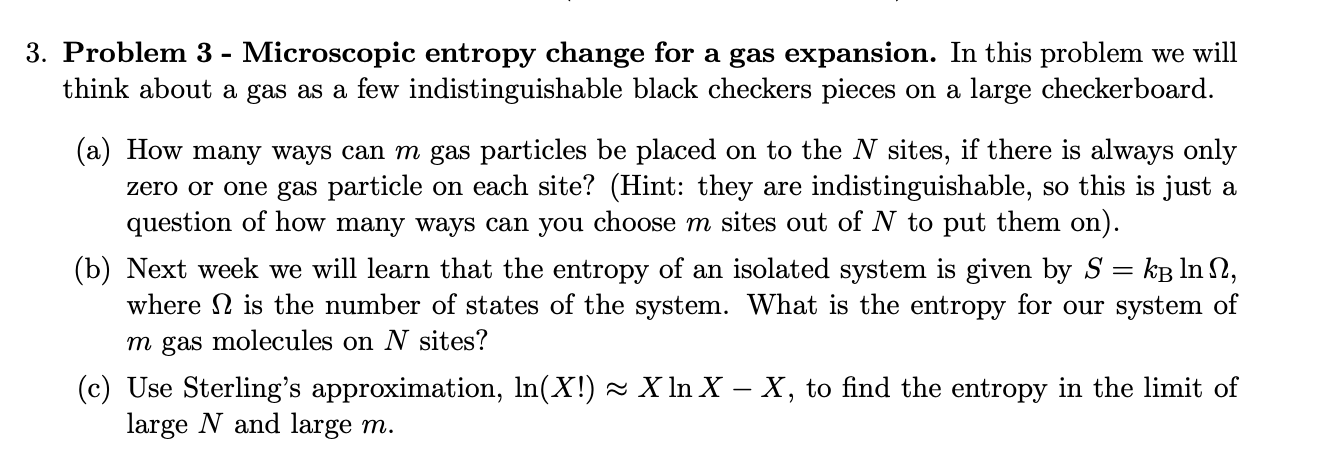
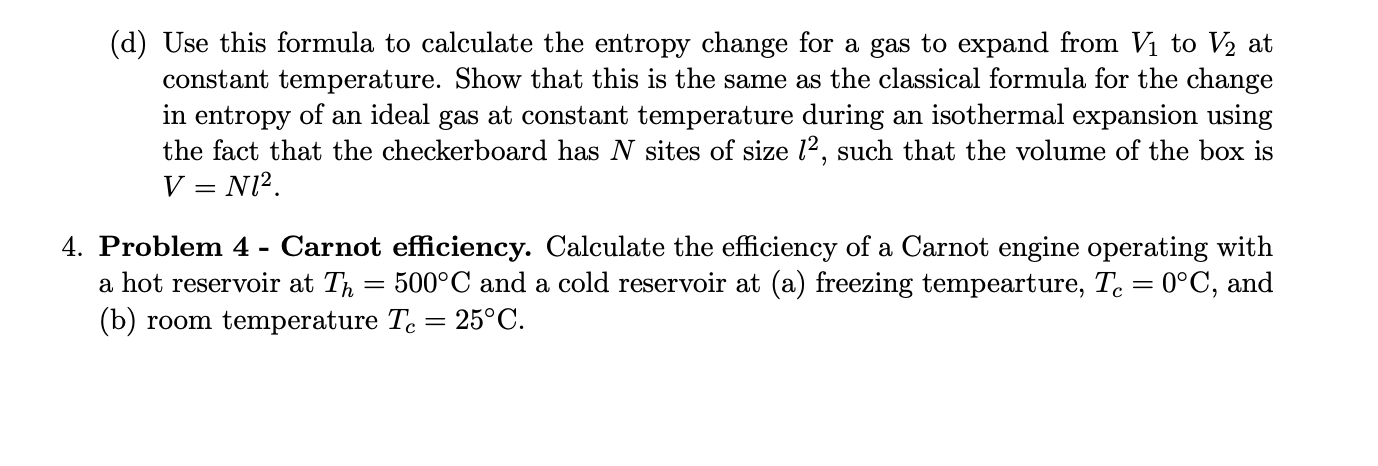
3. Problem 3 - Microscopic entropy change for a gas expansion. In this problem we will think about a gas as a few indistinguishable black checkers pieces on a large checkerboard. (a) How many ways can m gas particles be placed on to the N sites, if there is always only zero or one gas particle on each site? (Hint: they are indistinguishable, so this is just a question of how many ways can you choose m sites out of N to put them on). = (b) Next week we will learn that the entropy of an isolated system is given by S = kB lnn, where is the number of states of the system. What is the entropy for our system of m gas molecules on N sites? (c) Use Sterling's approximation, ln(X!) X ln X - X, to find the entropy in the limit of large N and large m. (d) Use this formula to calculate the entropy change for a gas to expand from V to V2 at constant temperature. Show that this is the same as the classical formula for the change in entropy of an ideal gas at constant temperature during an isothermal expansion using the fact that the checkerboard has N sites of size 12, such that the volume of the box is V = N1. 4. Problem 4 - Carnot efficiency. Calculate the efficiency of a Carnot engine operating with a hot reservoir at T = 500C and a cold reservoir at (a) freezing tempearture, Tc = 0C, and (b) room temperature Tc = 25C.