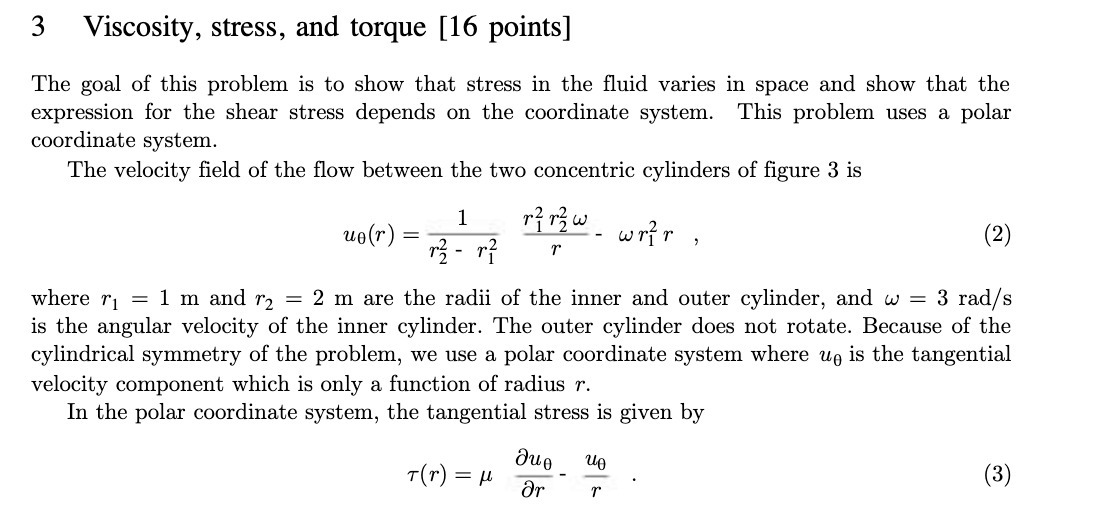
3 Viscosity, stress, and torque [16 points] The goal of this problem is to show that stress in the fluid varies in space and show that the expression for the shear stress depends on the coordinate system. This problem uses a polar coordinate system. The velocity field of the flow between the two concentric cylinders of figure 3 is ue(r): wrir, where r = 1 m and r = 2 m are the radii of the inner and outer cylinder, and w = 3 rad/s is the angular velocity of the inner cylinder. The outer cylinder does not rotate. Because of the cylindrical symmetry of the problem, we use a polar coordinate system where ue is the tangential velocity component which is only a function of radius r. In the polar coordinate system, the tangential stress is given by = 1 r - r r r r w T(r) = ue r uo r (3)