3-1.
3-2
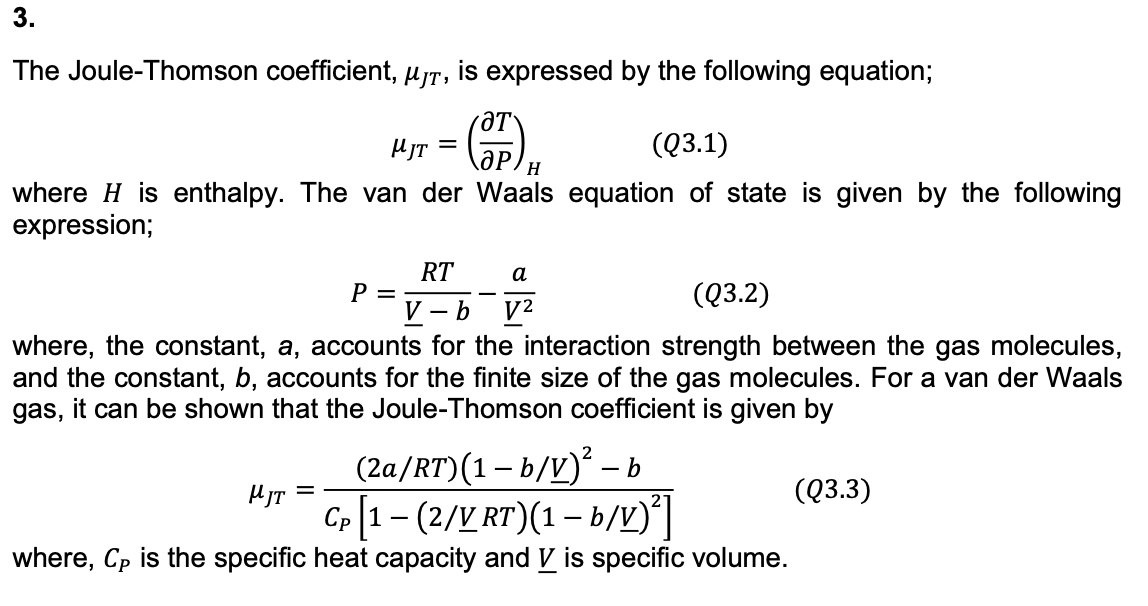
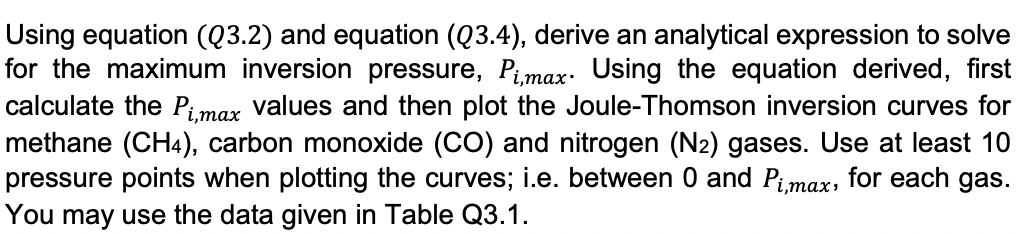
3. The Joule-Thomson coefficient, JT, is expressed by the following equation; JT=(PT)H(Q3.1) where H is enthalpy. The van der Waals equation of state is given by the following expression; P=VbRTV2a where, the constant, a, accounts for the interaction strength between the gas molecules, and the constant, b, accounts for the finite size of the gas molecules. For a van der Waals gas, it can be shown that the Joule-Thomson coefficient is given by JT=CP[1(2/VRT)(1b/V)2](2a/RT)(1b/V)2b where, CP is the specific heat capacity and V is specific volume. Using equation (Q3.2) and equation (Q3.4), derive an analytical expression to solve for the maximum inversion pressure, Pi,max. Using the equation derived, first calculate the Pi,max values and then plot the Joule-Thomson inversion curves for methane (CH4), carbon monoxide (CO) and nitrogen (N2) gases. Use at least 10 pressure points when plotting the curves; i.e. between 0 and Pi,max, for each gas. You may use the data given in Table Q3.1. According to the principle of Corresponding States, all fluids, when compared at the same reduced temperature and reduced pressure, have approximately the same compressibility factor and all deviate from ideal gas behavior to about the same degree. By plotting the Joule-Thomson inversion curves you have computed in part b) in terms of reduced variables, check if the principle of corresponding states holds for the inversion temperature, Ti. You may use the data given in Table Q3.1. 3. The Joule-Thomson coefficient, JT, is expressed by the following equation; JT=(PT)H(Q3.1) where H is enthalpy. The van der Waals equation of state is given by the following expression; P=VbRTV2a where, the constant, a, accounts for the interaction strength between the gas molecules, and the constant, b, accounts for the finite size of the gas molecules. For a van der Waals gas, it can be shown that the Joule-Thomson coefficient is given by JT=CP[1(2/VRT)(1b/V)2](2a/RT)(1b/V)2b where, CP is the specific heat capacity and V is specific volume. Using equation (Q3.2) and equation (Q3.4), derive an analytical expression to solve for the maximum inversion pressure, Pi,max. Using the equation derived, first calculate the Pi,max values and then plot the Joule-Thomson inversion curves for methane (CH4), carbon monoxide (CO) and nitrogen (N2) gases. Use at least 10 pressure points when plotting the curves; i.e. between 0 and Pi,max, for each gas. You may use the data given in Table Q3.1. According to the principle of Corresponding States, all fluids, when compared at the same reduced temperature and reduced pressure, have approximately the same compressibility factor and all deviate from ideal gas behavior to about the same degree. By plotting the Joule-Thomson inversion curves you have computed in part b) in terms of reduced variables, check if the principle of corresponding states holds for the inversion temperature, Ti. You may use the data given in Table Q3.1









