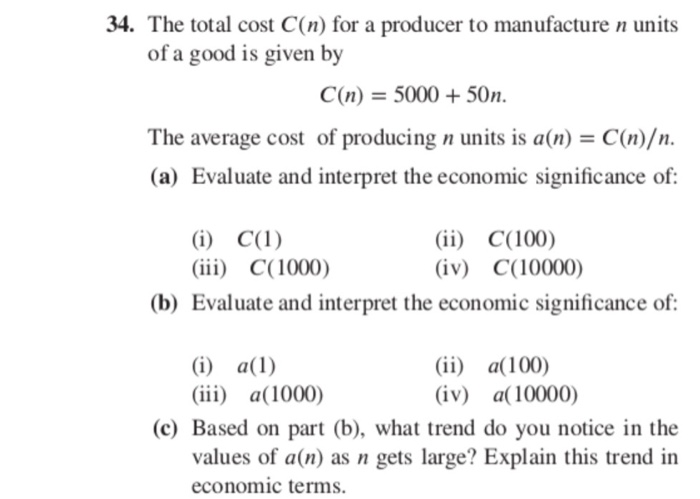
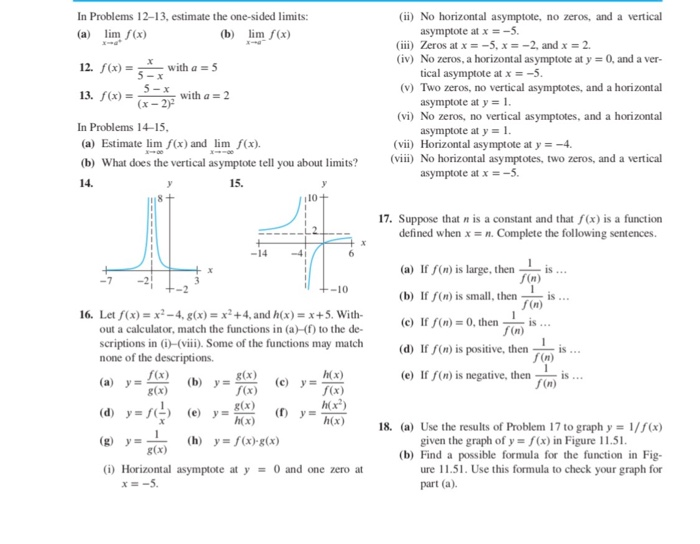
34. The total cost C(n) for a producer to manufacture n units of a good is given by C(n) = 5000 + 50n. The average cost of producing n units is a(n) = C(n). (a) Evaluate and interpret the economic significance of: (i) C(1) (ii) C(100) (iii) C(1000) (iv) C(10000) (b) Evaluate and interpret the economic significance of: (i) (1) (ii) a(100) (iii) (1000) (iv) a(10000) (c) Based on part (b), what trend do you notice in the values of a(n) as n gets large? Explain this trend in economic terms. 11.6 COMPARING POWER, EXPONENTIAL, AND LOG FUNCTIONS 471 In Problems 45-47, find a possible formula for the rational functions. 45. This function has zeros at x = 2 and x = 3. It has a ver- tical asymptote at x = 5. It has a horizontal asymptote of y = -3 46. The graph of y = g(x) has two vertical asymptotes: one at x = -2 and one at x = 3. It has a horizontal asymptote of y = 0. The graph of g crosses the x-axis once, at x = 5. In Problems 12-13, estimate the one-sided limits: (a) lim (x) (b) lim f(x) 12. f(x) = 5* with a = 5 5-x 13. f(x) = with a = 2 (x - 2) In Problems 14-15 (a) Estimate lim f(x) and lim f(x). (b) What does the vertical asymptote tell you about limits? 15. (ii) No horizontal asymptote, no zeros, and a vertical asymptote at x = -5. (iii) Zeros at x = -5, x = -2, and x = 2. (iv) No zeros, a horizontal asymptote at y = 0, and a ver- tical asymptote at x = -5. () Two zeros, no vertical asymptotes, and a horizontal asymptote at y = 1. (vi) No zeros, no vertical asymptotes, and a horizontal asymptote at y = 1. (vii) Horizontal asymptote at y = -4. (viii) No horizontal asymptotes, two zeros, and a vertical asymptote at x = -5. 14. 17. Suppose that n is a constant and that f(x) is a function defined when x = n. Complete the following sentences. -10 is (a) If f(n) is large, then (b) If f(n) is small, then (e) If f(n) = 0, then f(n) (d) If f(n) is positive, then (e) If f(n) is negative, then ---12-13 is is 16. Let f(x) = x2 -4,8(x) = x2 +4, and h(x) = x+5. With- out a calculator, match the functions in ( af) to the de- scriptions in (1)-(viii). Some of the functions may match none of the descriptions (a) y = f(x) (b) y = 8(x) h(x) y = g(x) f(x) f(x) (d) y=1" (e ya 8(x) h(x) ( y = h(x) h(x) (B) = 1 (h) y = f(x)-g(x) g(x) (i) Horizontal asymptote at y = 0 and one zero at XE=5. 18. (a) Use the results of Problem 17 to graph y = 1/f(x) given the graph of y = f(x)in Figure 11.51. (b) Find a possible formula for the function in Fig- ure 11.51. Use this formula to check your graph for part (a)