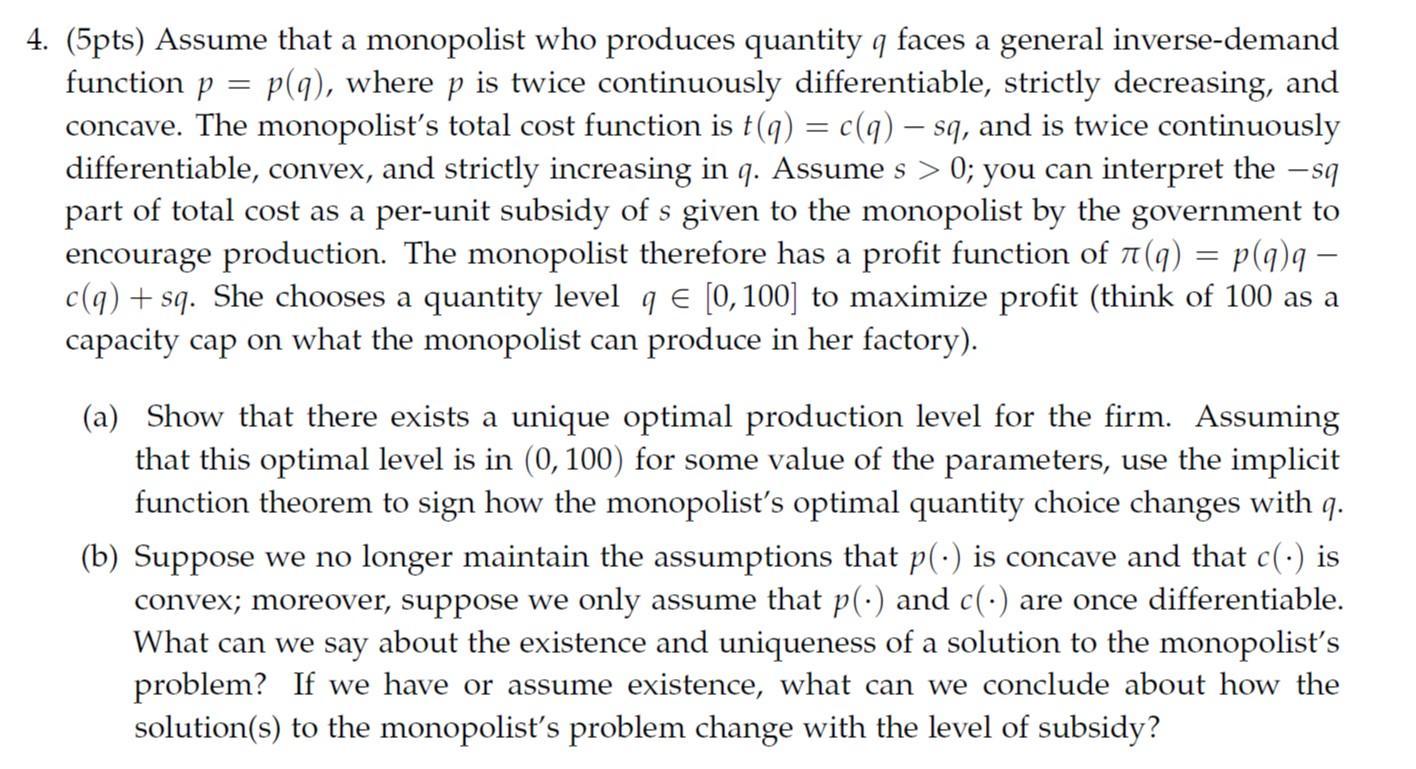
4. (5pts) Assume that a monopolist who produces quantity q faces a general inverse-demand function p = p(q), where p is twice continuously differentiable, strictly decreasing, and concave. The monopolist's total cost function is t(q) = c(q) sq, and is twice continuously differentiable, convex, and strictly increasing in q. Assume s> 0; you can interpret the -sq part of total cost as a per-unit subsidy of s given to the monopolist by the government to encourage production. The monopolist therefore has a profit function of 7(q) = p(q)q c(q) + sq. She chooses a quantity level q [0,100] to maximize profit (think of 100 as a capacity cap on what the monopolist can produce in her factory). (a) Show that there exists a unique optimal production level for the firm. Assuming that this optimal level is in (0, 100) for some value of the parameters, us the implicit function theorem to sign how the monopolist's optimal quantity choice changes with q. (b) Suppose we no longer maintain the assumptions that p(.) is concave and that c(.) is convex; moreover, suppose we only assume that p(.) and c(.) are once differentiable. What can we say about the existence and uniqueness of a solution to the monopolist's problem? If we have or assume existence, what can we conclude about how the solution(s) to the monopolist's problem change with the level of subsidy?