Question: 4. A twice differentiable function, f(x), has a domain of [-6, 6] (4) -6 < x < -2 x = -2 -2 < x

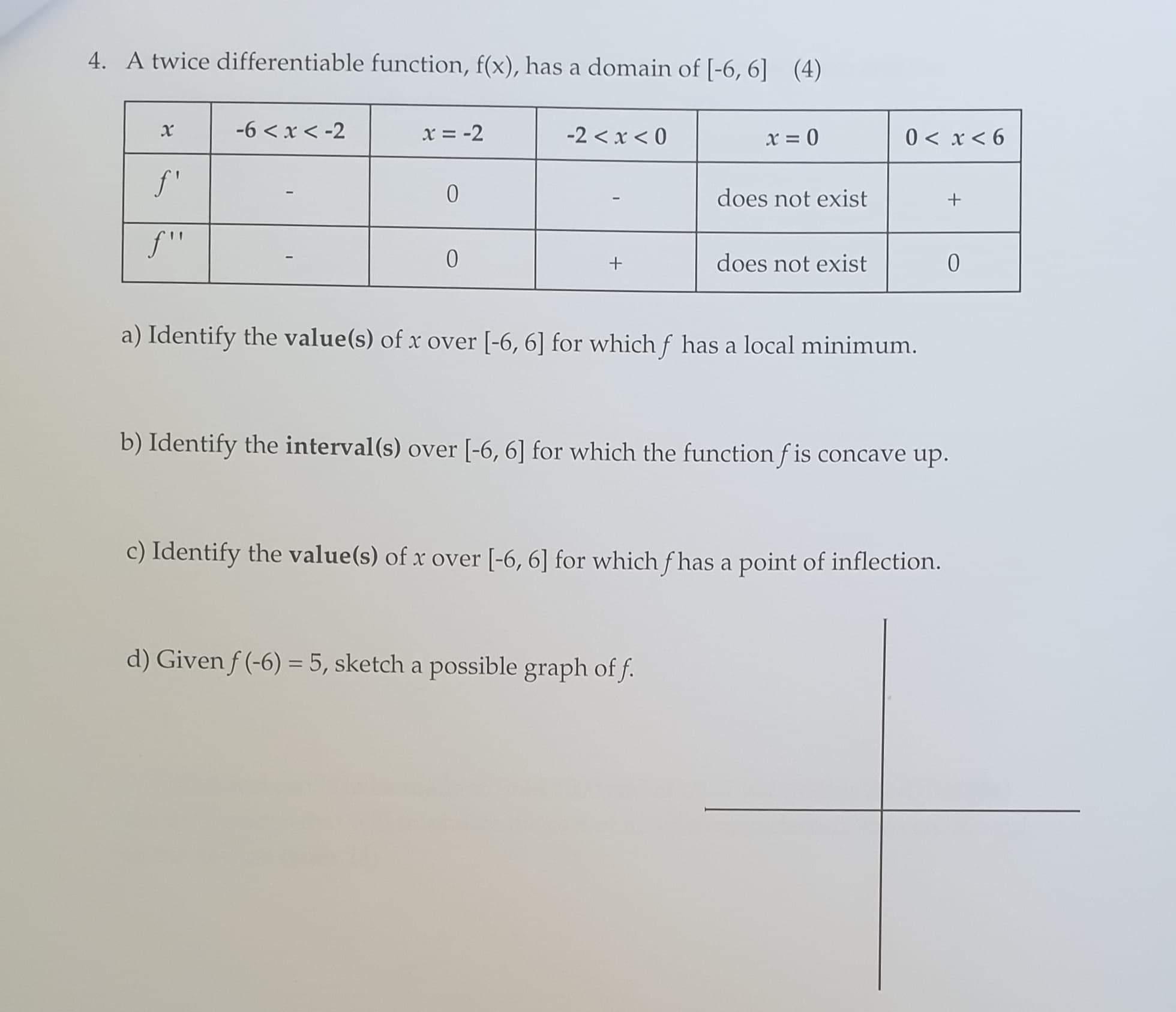
4. A twice differentiable function, f(x), has a domain of [-6, 6]

4. A twice differentiable function, f(x), has a domain of [-6, 6] (4) -6 < x < -2 x = -2 -2 < x < 0 x = 0 0 < x < 6 f' does not exist + does not exist a) Identify the value(s) of x over [-6, 6] for which f has a local minimum. b) Identify the interval(s) over [-6, 6] for which the function f is concave up. c) Identify the value(s) of x over [-6, 6] for which f has a point of inflection. d) Given f (-6) = 5, sketch a possible graph of f.

Step by Step Solution

★★★★★

3.43 Rating (153 Votes )

There are 3 Steps involved in it

1 Expert Approved Answer

Step: 1 Unlock blur-text-image

Question Has Been Solved by an Expert!

Get step-by-step solutions from verified subject matter experts

Step: 2 Unlock

Step: 3 Unlock

Students Have Also Explored These Related Mathematics Questions!

A differentiable function f on a convex, open set S in n is convex if and only if for every x0; x S. f is strictly convex if and only if for every x x0 S. (32)

A differentiable function f on an open interval S is (strictly) convex if and only if f is (strictly) increasing. Combined with exercises 4.35 and 4.36, this means that convex and concave functions...

What is the shear capacity of the RC beam described below considering the steel reinforcement and using the formula: VRsyAw 2fyd cot 8/s The shear reinforcement in the beam is provided by sets of...

seventh pages Chapter 3 Curve Sketching How much metal would be required to make a 400-mL soup can? What is the least amount of cardboard needed to build a box that holds 3000 cm3 of cereal? The...

AP Style Problem 1. The table below shows some points on a function f that is both continuous and differentiable on the closed interval [0, 20] 5 15 20 10 f(x) 10 22 40 22 10 A) f(x) is increasing in...

please solve all the questions in the images! Thank you! 1. Find the particular solution to y ' = 3sin(x) given the general solution is y = C - 3cos(x) and the initial condition y(TT) = 1. (5 points)...

Reference: Calculus and Its Applications, 12th, by Marvin L. Bittinger and David Ellenbogen Book Elementary Applied Calculus I need help with questions These are practice questions Links to Book...

1. Find the domain of the function f ( x )= x 2 x +2 1) ______ (A) D=( ,2 ) ( 2, ) (B) D=( ,2 ) (2, ) (C) D= ( 2, ) (D) D=(2, ) (E) none of the above 1 2. According to the Center for Disease Control...

Section 2.6: (page 120) Numbers 4, 5, 7-9 all, 16, 17 Section 2.7: (page 133) Numbers 3-6 all, 9-12 all Section 2.8: (page 140) Numbers 10-12 all textbrokercom X 4." Final Exam X 6 1 Canvas LMS X e...

answer the question clearly You are building a flight-control system for which a convincing safety case must be made. Would you assign the tasks of safety requirements engineering, test case...

Why are results or outcomes requested with CIT? What could be done with these data?

Evaluate the following integral: 2015 -2015 [x] dx

I. Executive Summary. Provide a brief overview of the key findings and recommendations from your portfolio analysis and research. II . Portfolio Analysis. Research and analyze a portfolio of at least...

2. The first who talked about medical ethics was Select one: a. Thomas Alaquinas b. Hippocrates c Al-Razi d. Ishag Bin Ali Al-Rohawi

Which of the following double displacement reactions will occur - complete the equations that do: Mg+2, SO42 + 2 Na+, 2 OH- - Ni+2, CI- + 2 Na+, 2 Br Ag+, NO3+Na+1, 1-1 -> - Ca+2, Cl- AI+3,3 NO3- -...

One interesting legal fact that deepens this discussion is that several states, including California, New York, and Colorado, have laws protecting employees from being fired for lawful off-duty...

Q1 (3 points) The graph below shows the position of a particle on the number line over a time period of 10 minutes (time is measured on the horizontal axis, while position is measured on the vertical...

A Question 14 (1 point) Retake question Let g(x) be twice differentiable function. Which of the following statements about the function f(a) = g(a) + 22 is TRUE? Olf g'(a) 3 on [-1, 4), then f(-1) is...

Paraphrasing ool X MATH_1241_A02_202315:A5 X 6 MATH1241Assignment4(1).pdf X a PSC|202.FLEX--2023 Winter X + C G File [Users/zawadarwad/Downloads/MATH1241Assignment4%20(1).pdf MATH1241Assignment4...

DUE: 3/6 WEEKLY REVIEW #1 X g(x) g'(x) -2 12 -5 -1 10 -2 0 7 0 1 4 -4 2 2 -1 graph of h 3 -2 -3 Let f be the function defined by f(x) = sin(nx) + In(2 - x). Let g be a strictly decreasing twice...

4. (5 points) Concert tickets went on sale at noon (t = 0) and were sold out within 9 hours. The number of people waiting in line to purchase tickets at time t is modeled by a twice- differentiable...

Suppose that f is a twice differentiable function (everywhere). The so-called arclength kernel of f is the function h defined by h(ac) = V1+ f' (x)2 and is used to help compute the length of a curve...