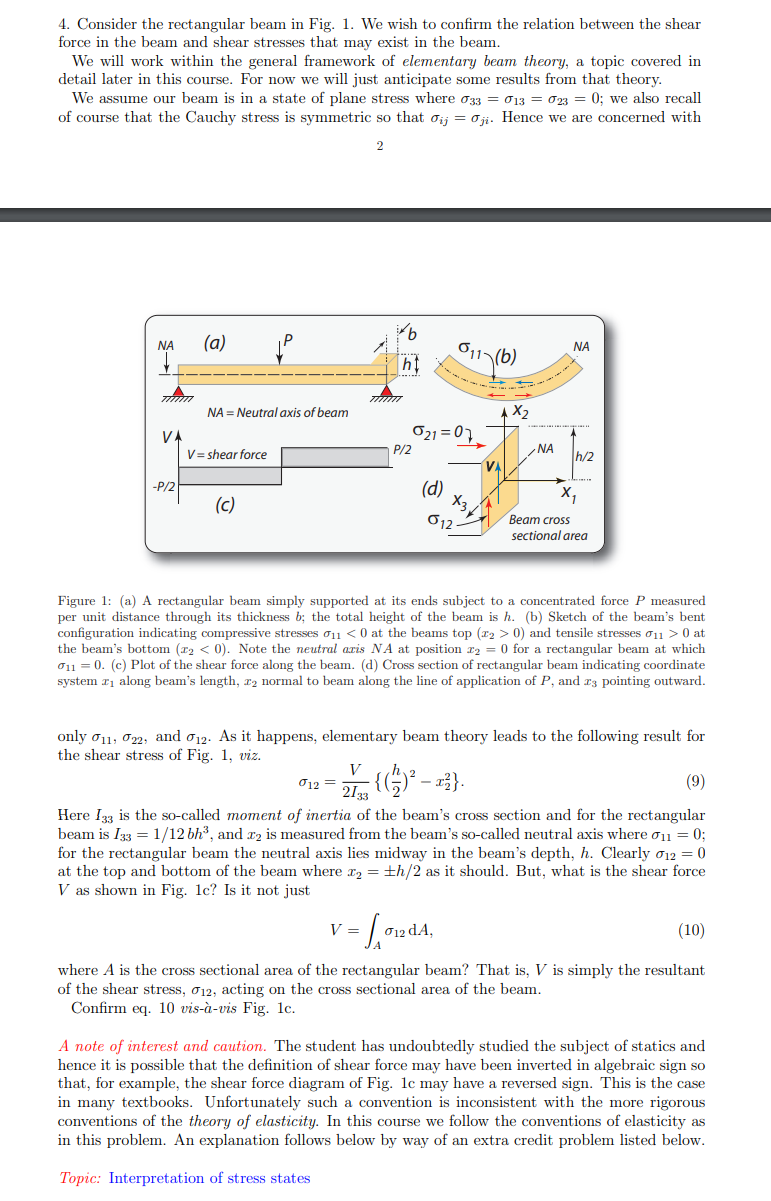
4. Consider the rectangular beam in Fig. 1. We wish to confirm the relation between the shear force in the beam and shear stresses that may exist in the beam. We will work within the general framework of elementary beam theory, a topic covered in detail later in this course. For now we will just anticipate some results from that theory. We assume our beam is in a state of plane stress where 33 = 13 = 023 = 0; we also recall of course that the Cauchy stress is symmetric so that = ji. Hence we are concerned with 2 (a) NA = Neutral axis of beam V = shear force -P/2 (c) h NA 011(b) 021-07 NA P/2 (d) X3 012 Beam cross sectional area Figure 1: (a) A rectangular beam simply supported at its ends subject to a concentrated force P measured per unit distance through its thickness b; the total height of the beam is h. (b) Sketch of the beam's bent configuration indicating compressive stresses 11 0) and tensile stresses 11 > 0 at the beam's bottom (x2 < 0). Note the neutral aris NA at position 2 = 0 for a rectangular beam at which 110. (c) Plot of the shear force along the beam. (d) Cross section of rectangular beam indicating coordinate system 21 along beam's length, x2 normal to beam along the line of application of P, and 23 pointing outward. only 11, 22, and 12. As it happens, elementary beam theory leads to the following result for the shear stress of Fig. 1, viz. V 012= (-4) (9) Here I33 is the so-called moment of inertia of the beam's cross section and for the rectangular beam is 133 = 1/12 bh, and x2 is measured from the beam's so-called neutral axis where 11 = 0; for the rectangular beam the neutral axis lies midway in the beam's depth, h. Clearly 12 = 0 at the top and bottom of the beam where x2 = h/2 as it should. But, what is the shear force V as shown in Fig. 1c? Is it not just 012 dA, (10) where A is the cross sectional area of the rectangular beam? That is, V is simply the resultant of the shear stress, 12, acting on the cross sectional area of the beam. Confirm eq. 10 vis--vis Fig. 1c. A note of interest and caution. The student has undoubtedly studied the subject of statics and hence it is possible that the definition of shear force may have been inverted in algebraic sign so that, for example, the shear force diagram of Fig. 1c may have a reversed sign. This is the case in many textbooks. Unfortunately such a convention is inconsistent with the more rigorous conventions of the theory of elasticity. In this course we follow the conventions of elasticity as in this problem. An explanation follows below by way of an extra credit problem listed below. Topic: Interpretation of stress states