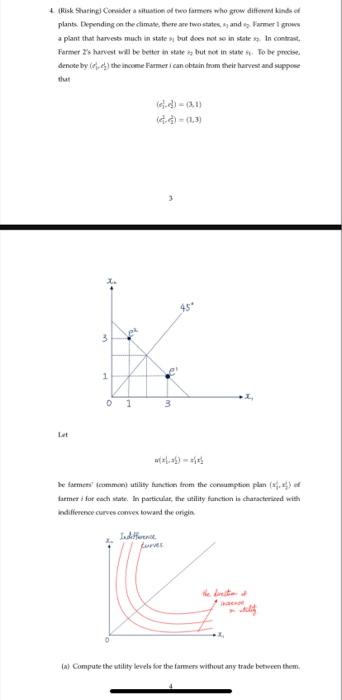
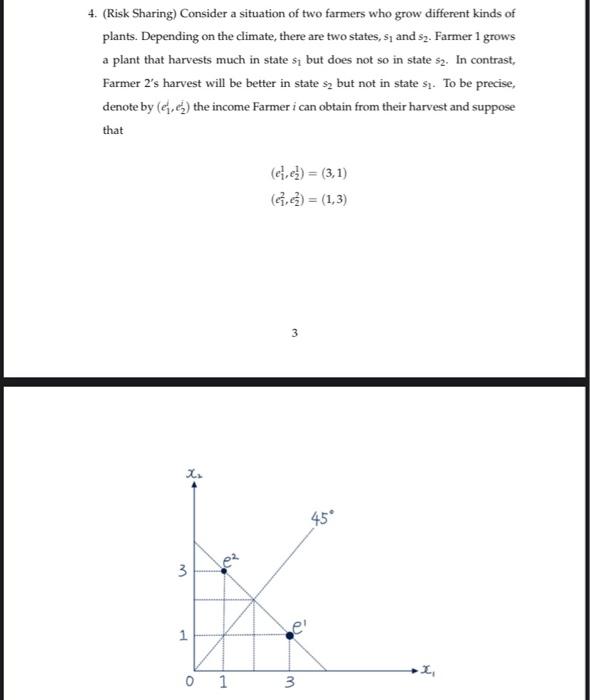
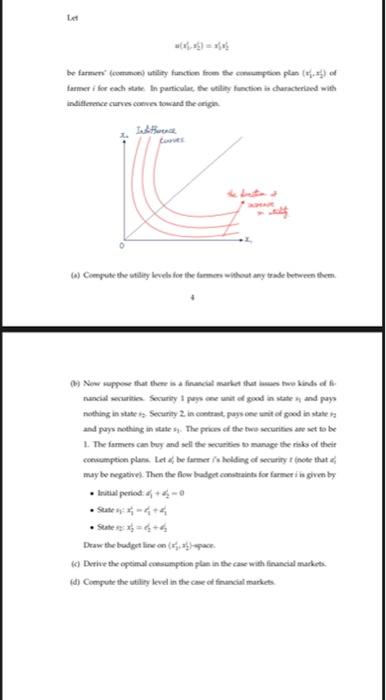
4 fok Sharing Considera situation of two farmers who grow different kinds of plants. Depending on the climate, there are two states and Farmer grow a plant that harvest much in state but does not so in states. In contrast, Farmer's harvest will be better in state bute in se To be precise denete by the income Farmer i can obtain from their harvest and suppose thu )-1) 45 3 1 1 3 he formen community hunction from the consumption plan farmer for each state. In particular, the utility function is characterized with Indifference curves contextoward the origin Dr. Curves tiet . (a) Compute the utility levels for the farmers without any trade between them Game for each state in particular the function characterized with indicare coward the origin Luts a) Compute the utility for the farm without any trade the b) Now suppose that there is a financial market that is the of mandal Security persone unit of goods and y othing in state Security 2. in c.paysement of goods and pays nothing in the of the weet 1. The farmers can buy and see the securities to manage the modele consumption plateforholding of security way he tel. Then the castigum Daw the tradition Derive the optimal comptan plan in the case with financial market (d) Compute the stayed in the case ofercial market 1. (Medium of Exchange and Specialization) Consider the example of 3 producers, Ann the Apple orchard, Bob the Banana grower, and Chris the Chocolatier. To be more specific, assume that each pro ducer has 12 hours/day of labor that they can devote to produce goods. For the technology, assume that Ann can produce 1 unit of apples from 2 hours/day of labor and can pro- duce 1 unit of bananas from 4 hours/day. Bob can produce 1 unit of bananas from 2 hours/day of labor and can pro- duce 1 unit of chocolate from 4 hours/day. . Chris can produce 1 unit of chocolate from 2 hours/day of labor and can produce 1 unit of apples from 4 hours/day. In terms of their preference, let's assume . Ann wants to consume apples and bananas. Bob wants to consume bananas and chocolate . Chris wants to consume chocolate and apples. Also assume that each producer consumes their desired goods the same amount, that is, for example, Ann prefer consuming 3 units of apples and 3 units of ba- 1 nanas to 2 units of apples and 2 unit of bananas consuming 2 units of apples and 2 units of bananas tol unit of apples and I unit of bananas, and consuming I unit of apples and 1 unit of bananas to any other combinations of goods. Therefore, cach producer wants to consume two types of commodities while he/she has a commodity to which he/she is good at producing. The following problem asks you how money as a medium of exchange helps the producers to engage in their specialization and how the economy achieves an efficient outcome (a) First assume that their is no money and their is no centralized marketplace, in which case only barter trade between two of the producers is possible. Explain why in this case no barter trade occurs between the producers. (b) If there is no trade between the producers, they have to produce goods for themselves for consumption. How much amount of goods does each pro- ducer consume? (Due to the symmetry of the producers, it is enough to answer how many units of apples and bananas Ann would produce and consume) (C) Now let's assume that Ann has a unit of money and that money is surely accepted as a method of payment. What would happen to this economy? (Hint: If trades are possible, then it is more efficient for Ann to focus on pro- ducing apples, for Bob on producing Bananas, and for Chris on producing chocolate. After each producer focuses on their own specialization, how the trades occur between the producers?) 2. (Present-Value Pricing of a Financial Asset) Bob is working for a finance com- pany and creating a new financial commodity. The commodity pays off $50 in 1 year from today, $50 in 2 years from today, $200 in 3 years from today, and $200 in 4 years from today. Bob already knows that the market interest rate is i = 10% and wants to compute the price of the asset. Draw the time lines of the commodity's payoffs and derive the formula to compute the price of the asset 2 3. (Application of Demand-Supply Analysis of Bonds) In the aftermath of the global economic crisis that started to take hold in 2008, U.S. government budget deficits increased dramatically, yet interest rates on U.S. Treasury debt fell sharply and stayed low for quite a while. To understand this phenomenon, answer the following questions: (a) Using the demand and supply analysis in the U.S. Treasury bond markets, explain the effect of an increase in government budget deficits. (b) During the global economic crisis, investors tended to avoid trading risky assets such as stocks or company-issued bonds. This change in investor sen- timents caused decreases in liquidity of securities other than the Treasury bonds; in other words, the Treasury bonds became more liquid relative to other assets. One reasonable explanation of the phenomenon cited above is the effect of this liquidity change outweighed the effect of government bud- get deficits. Explain the effect of liquidity on Treasury bonds using demand and supply analysis. 4. (Risk Sharing) Consider a situation of two farmers who grow different kinds of plants. Depending on the climate, there are two states, s; and sz. Farmer 1 grows a plant that harvests much in state s but does not so in state s2. In contrast, Farmer 2's harvest will be better in states, but not in states. To be precise, denote by (4.c) the income Farmer i can obtain from their harvest and suppose that (cle) = (3,1) e, ) = (1,3) 450 3 1 e 0 1 3 Lt be farmers commodity function from the common plan (2) farmer for each sme In particulat de utility function is characterised with indideme curvescentowand the eigin LA w Compute the utility levels for the formers without any trade between them New suppe that there is a financial markets as we kinds of ancial securities Security per cent el goed in state and pays nothing in states Security 2. in contrast, pays one unit of goed in states and pays nothing in states. The prices of the bwa securities are set to be 1. The farmets can buy and sell the securities to manage the risks of their consumption plans et de formes bolding of security (note that may be negative. Then the flow budget constraints for farmer in given by Initial periodo . Sute-4 Daw the budget line on a (c) Derive the optimal consumption plan in the case with financial markets. 6) Compute the thing level in the case of financial markets 4 fok Sharing Considera situation of two farmers who grow different kinds of plants. Depending on the climate, there are two states and Farmer grow a plant that harvest much in state but does not so in states. In contrast, Farmer's harvest will be better in state bute in se To be precise denete by the income Farmer i can obtain from their harvest and suppose thu )-1) 45 3 1 1 3 he formen community hunction from the consumption plan farmer for each state. In particular, the utility function is characterized with Indifference curves contextoward the origin Dr. Curves tiet . (a) Compute the utility levels for the farmers without any trade between them Game for each state in particular the function characterized with indicare coward the origin Luts a) Compute the utility for the farm without any trade the b) Now suppose that there is a financial market that is the of mandal Security persone unit of goods and y othing in state Security 2. in c.paysement of goods and pays nothing in the of the weet 1. The farmers can buy and see the securities to manage the modele consumption plateforholding of security way he tel. Then the castigum Daw the tradition Derive the optimal comptan plan in the case with financial market (d) Compute the stayed in the case ofercial market 1. (Medium of Exchange and Specialization) Consider the example of 3 producers, Ann the Apple orchard, Bob the Banana grower, and Chris the Chocolatier. To be more specific, assume that each pro ducer has 12 hours/day of labor that they can devote to produce goods. For the technology, assume that Ann can produce 1 unit of apples from 2 hours/day of labor and can pro- duce 1 unit of bananas from 4 hours/day. Bob can produce 1 unit of bananas from 2 hours/day of labor and can pro- duce 1 unit of chocolate from 4 hours/day. . Chris can produce 1 unit of chocolate from 2 hours/day of labor and can produce 1 unit of apples from 4 hours/day. In terms of their preference, let's assume . Ann wants to consume apples and bananas. Bob wants to consume bananas and chocolate . Chris wants to consume chocolate and apples. Also assume that each producer consumes their desired goods the same amount, that is, for example, Ann prefer consuming 3 units of apples and 3 units of ba- 1 nanas to 2 units of apples and 2 unit of bananas consuming 2 units of apples and 2 units of bananas tol unit of apples and I unit of bananas, and consuming I unit of apples and 1 unit of bananas to any other combinations of goods. Therefore, cach producer wants to consume two types of commodities while he/she has a commodity to which he/she is good at producing. The following problem asks you how money as a medium of exchange helps the producers to engage in their specialization and how the economy achieves an efficient outcome (a) First assume that their is no money and their is no centralized marketplace, in which case only barter trade between two of the producers is possible. Explain why in this case no barter trade occurs between the producers. (b) If there is no trade between the producers, they have to produce goods for themselves for consumption. How much amount of goods does each pro- ducer consume? (Due to the symmetry of the producers, it is enough to answer how many units of apples and bananas Ann would produce and consume) (C) Now let's assume that Ann has a unit of money and that money is surely accepted as a method of payment. What would happen to this economy? (Hint: If trades are possible, then it is more efficient for Ann to focus on pro- ducing apples, for Bob on producing Bananas, and for Chris on producing chocolate. After each producer focuses on their own specialization, how the trades occur between the producers?) 2. (Present-Value Pricing of a Financial Asset) Bob is working for a finance com- pany and creating a new financial commodity. The commodity pays off $50 in 1 year from today, $50 in 2 years from today, $200 in 3 years from today, and $200 in 4 years from today. Bob already knows that the market interest rate is i = 10% and wants to compute the price of the asset. Draw the time lines of the commodity's payoffs and derive the formula to compute the price of the asset 2 3. (Application of Demand-Supply Analysis of Bonds) In the aftermath of the global economic crisis that started to take hold in 2008, U.S. government budget deficits increased dramatically, yet interest rates on U.S. Treasury debt fell sharply and stayed low for quite a while. To understand this phenomenon, answer the following questions: (a) Using the demand and supply analysis in the U.S. Treasury bond markets, explain the effect of an increase in government budget deficits. (b) During the global economic crisis, investors tended to avoid trading risky assets such as stocks or company-issued bonds. This change in investor sen- timents caused decreases in liquidity of securities other than the Treasury bonds; in other words, the Treasury bonds became more liquid relative to other assets. One reasonable explanation of the phenomenon cited above is the effect of this liquidity change outweighed the effect of government bud- get deficits. Explain the effect of liquidity on Treasury bonds using demand and supply analysis. 4. (Risk Sharing) Consider a situation of two farmers who grow different kinds of plants. Depending on the climate, there are two states, s; and sz. Farmer 1 grows a plant that harvests much in state s but does not so in state s2. In contrast, Farmer 2's harvest will be better in states, but not in states. To be precise, denote by (4.c) the income Farmer i can obtain from their harvest and suppose that (cle) = (3,1) e, ) = (1,3) 450 3 1 e 0 1 3 Lt be farmers commodity function from the common plan (2) farmer for each sme In particulat de utility function is characterised with indideme curvescentowand the eigin LA w Compute the utility levels for the formers without any trade between them New suppe that there is a financial markets as we kinds of ancial securities Security per cent el goed in state and pays nothing in states Security 2. in contrast, pays one unit of goed in states and pays nothing in states. The prices of the bwa securities are set to be 1. The farmets can buy and sell the securities to manage the risks of their consumption plans et de formes bolding of security (note that may be negative. Then the flow budget constraints for farmer in given by Initial periodo . Sute-4 Daw the budget line on a (c) Derive the optimal consumption plan in the case with financial markets. 6) Compute the thing level in the case of financial markets