Question
4. In many interesting applications, we need to know what it means by two curves are orthogonal (perpendicular) to each other. (a) Let's begin with
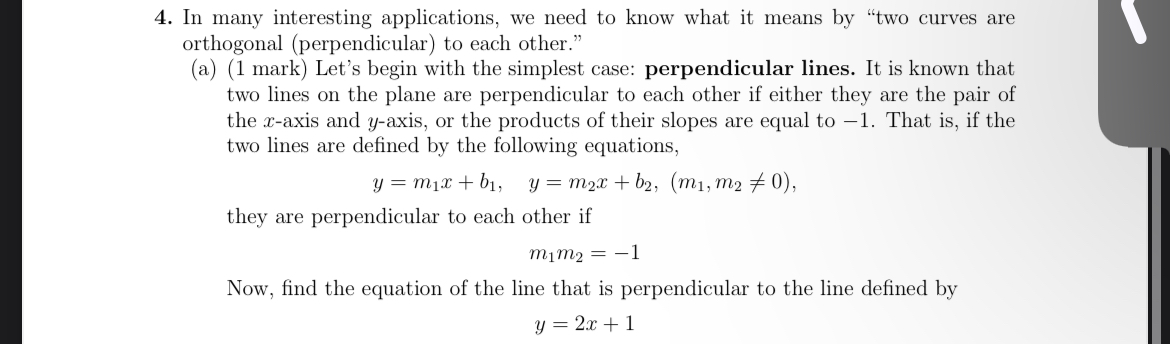
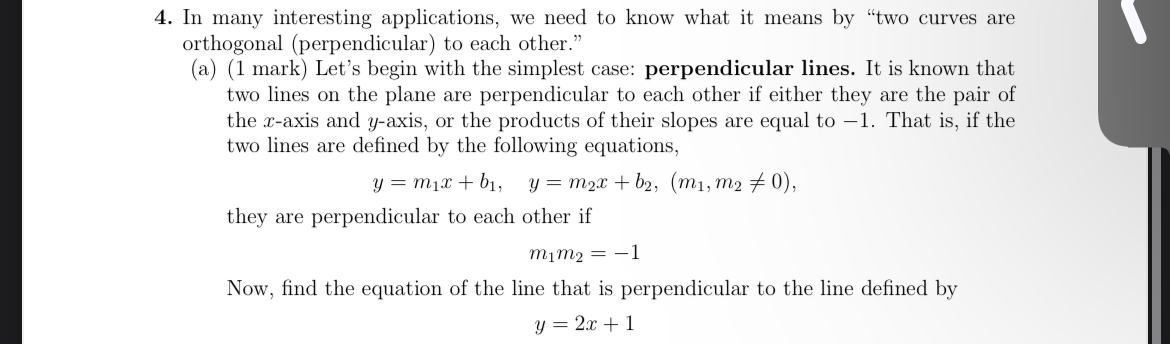
4. In many interesting applications, we need to know what it means by "two curves are orthogonal (perpendicular) to each other." (a) Let's begin with the simplest case: perpendicular lines. It is known that two lines on the plane are perpendicular to each other if either they are the pair of the x-axis and y-axis, or the products of their slopes are equal to ?1. That is, if the two lines are defined by the following equations,
y=m1x+b1, y=m2x+b2, (m1,m2 ?=0), they are perpendicular to each other if m1m2 = ?1
Now, find the equation of the line that is perpendicular to the line defined by y = 2x + 1
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


