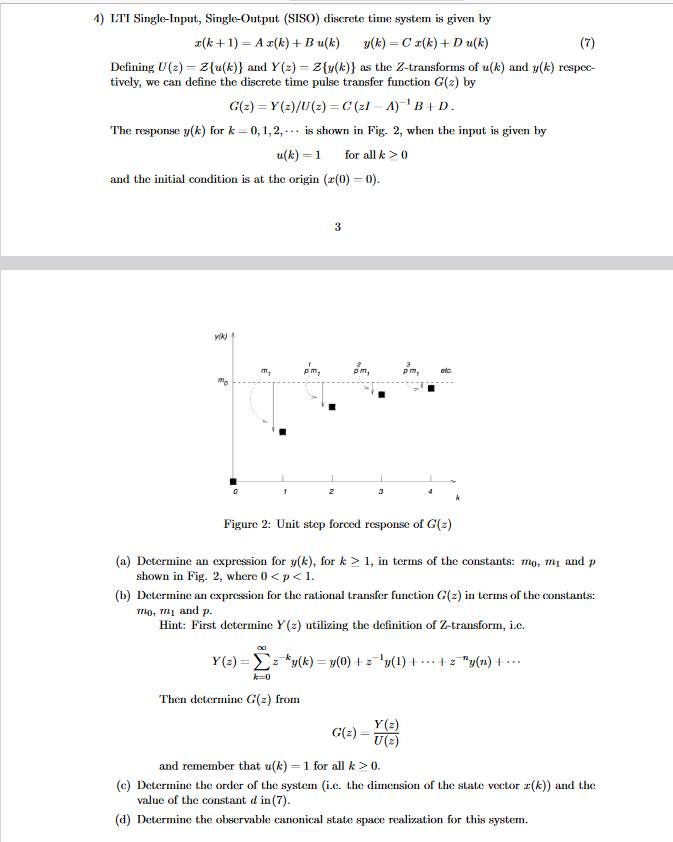
4) LTI Single-Input, Single-Output (SISO) discrete time system is given by x(k+1) = A x(k) + Bu(k) y(k)=Cx(k) + Du(k) (7) Defining U(z) - Z{u(k)} and Y(z)-Z{y(k)} as the Z-transforms of u(k) and y(k) respec- tively, we can define the discrete time pulse transfer function G(z) by G(z)=Y (2)/U(z)=C(zI-A) B+D. The response y(k) for k0, 1, 2, is shown in Fig. 2, when the input is given by u(k)=1 for all k > 0 and the initial condition is at the origin (z(0) = 0). v(K) mo m 1 pm, 3 2 pm, Then determine G(z) from Figure 2: Unit step forced response of G(z) (a) Determine an expression for y(k), for k> 1, in terms of the constants: mo, m and p shown in Fig. 2, where 0 < p < 1. pm, (b) Determine an expression for the rational transfer function G(z) in terms of the constants: mo, my and p. Hint: First determine Y(z) utilizing the definition of Z-transform, i.e. 00 Y(z)=zky(k)= y(0) +zy(1) ++ z"y(n) + ... G(z) = Y(z) U () and remember that u(k)=1 for all k > 0. (c) Determine the order of the system (i.c. the dimension of the state vector z(k)) and the value of the constant d in (7). (d) Determine the observable canonical state space realization for this system.