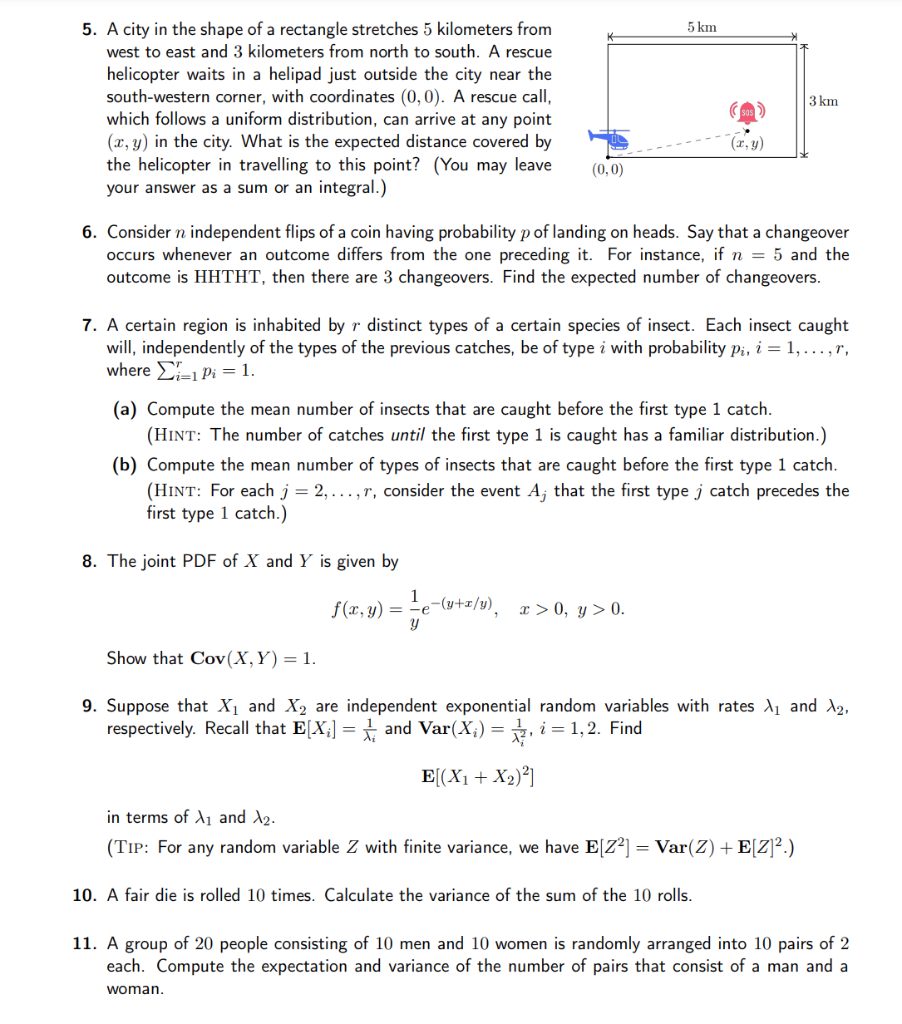
5. A city in the shape of a rectangle stretches 5 kilometers from west to east and 3 kilometers from north to south. A rescue helicopter waits in a helipad just outside the city near the south-western corner, with coordinates (0,0). A rescue call, which follows a uniform distribution, can arrive at any point (x, y) in the city. What is the expected distance covered by the helicopter in travelling to this point? (You may leave your answer as a sum or an integral.) 5 km (x, y) (0,0) 3 km 6. Consider n independent flips of a coin having probability p of landing on heads. Say that a changeover occurs whenever an outcome differs from the one preceding it. For instance, if n = 5 and the outcome is HHTHT, then there are 3 changeovers. Find the expected number of changeovers. 7. A certain region is inhabited by r distinct types of a certain species of insect. Each insect caught will, independently of the types of the previous catches, be of type i with probability pi, i = 1,...,r, where Pi 1. (a) Compute the mean number of insects that are caught before the first type 1 catch. (HINT: The number of catches until the first type 1 is caught has a familiar distribution.) (b) Compute the mean number of types of insects that are caught before the first type 1 catch. (HINT: For each j = 2,...,r, consider the event A; that the first type j catch precedes the first type 1 catch.) 8. The joint PDF of X and Y is given by 1 f(x, y) = -e-(y+x/y) x > 0, y >0. Y Show that Cov(X, Y) = 1. 9. Suppose that X and X2 are independent exponential random variables with rates 1 and 2, respectively. Recall that E[X;] = and Var(X) = 1, i = 1,2. Find in terms of A1 and A2. E[(X1+ X2)] (TIP: For any random variable Z with finite variance, we have E[Z2] = Var(Z) +E[Z].) 10. A fair die is rolled 10 times. Calculate the variance of the sum of the 10 rolls. 11. A group of 20 people consisting of 10 men and 10 women is randomly arranged into 10 pairs of 2 each. Compute the expectation and variance of the number of pairs that consist of a man and a woman.