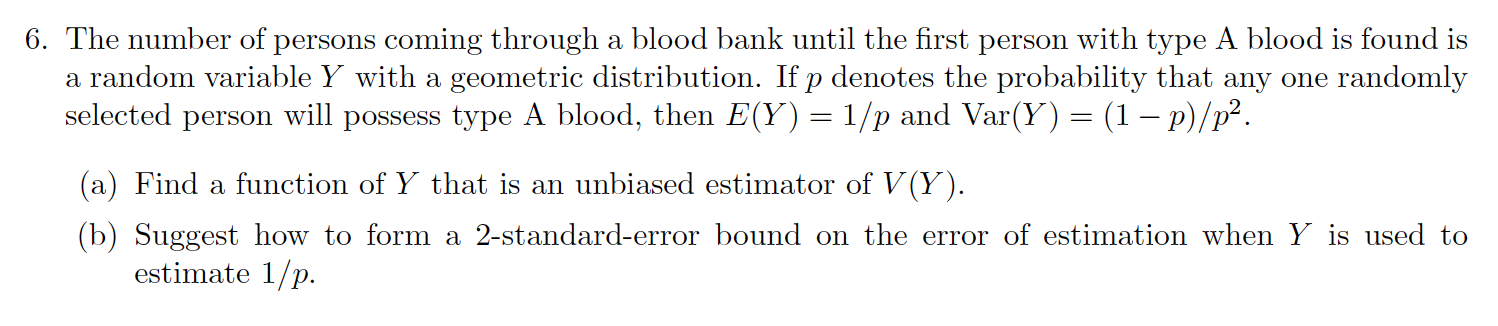
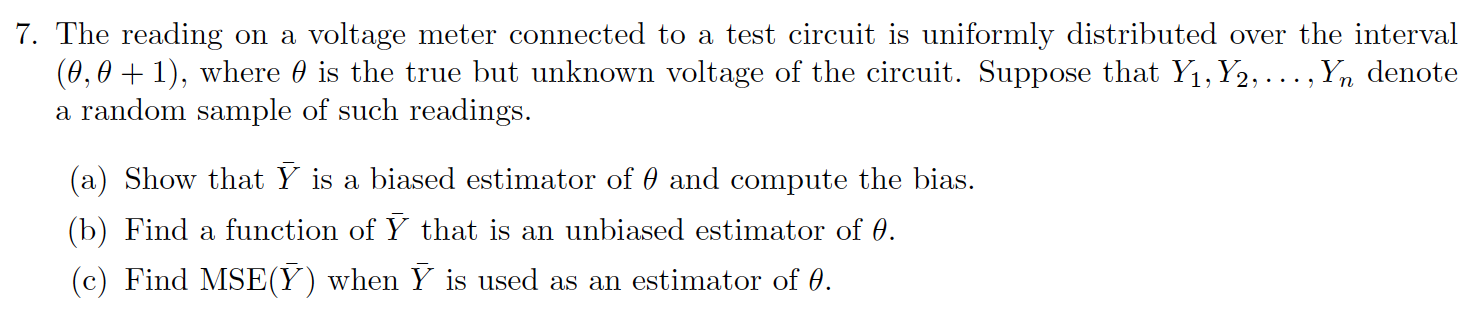
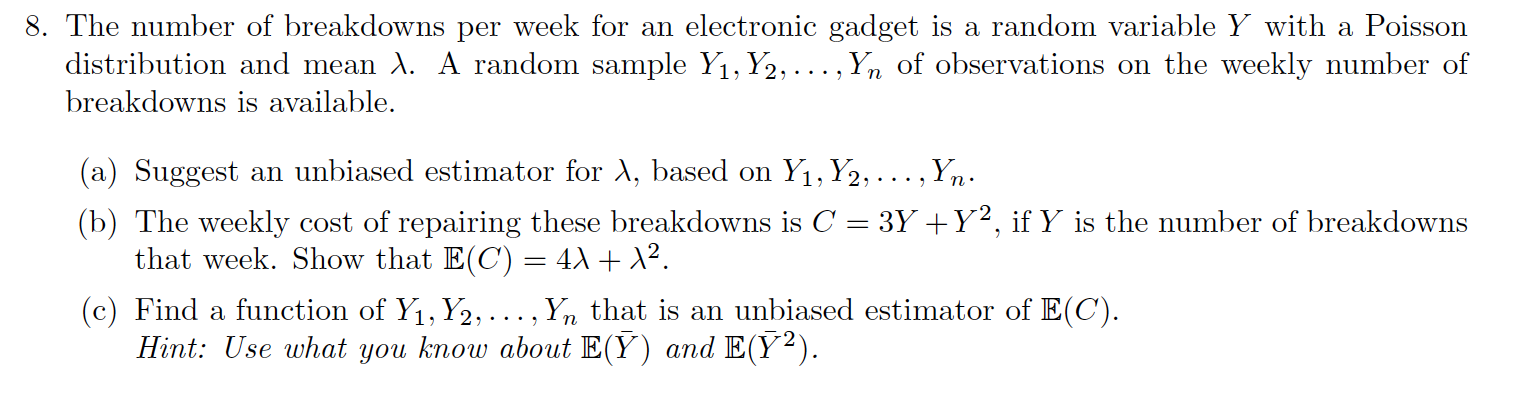
5. A survey is used to quantify the support for an increase in education spending. The pollster tells us that the approval rating for the increase is 60% with 4% standard error. Respondents have been modeled with independent identidally distributed Bernoulli random variables. 'STAT 120B, Spring 2017 Homework 4 Due May 15, 2017 (3.) Approximately how many people did the pollster survey? (b) Even if the true approval rating is 2 standard error away from the pollster's estimate, there would be majority support for the increased spending. What is the lower bound on the probability that approval rating is within 2 standard errors. Hint: use Chebyshev's inequality. 6. The number of persons coming through a blood bank until the rst person with type A blood is found is a random variable Y With a geometric distribution. If 3:) denotes the probability that any one randomly selected person will possess type A blood, then E (Y) = 1 / p and Var(Y) = (1 p)/p2. (a) Find a function of Y that is an unbiased estimator of V(Y). (b) Suggest how to form a 2standarderr0r bound on the error of estimation when Y is used to estimate 1/1). 7. The reading on a voltage meter connected to a test circuit is uniformly distributed over the interval (6, 9 + 1), where 9 is the true but unknown voltage of the circuit. Suppose that Y1, Y2, . . . ,Yn denote a random sample of such readings. (a) Show that if is a biased estimator of 6 and compute the bias. (b) Find a function of Y that is an unbiased estimator 0f 6. (c) Find M81307) When Y is used as an estimator of 6. 8. The number of breakdowns per week for an electronic gadget is a random variable Y with a Poisson distribution and mean A. A random sample Y1,l',...,Yn of observations on the weekly number of breakdowns is available. (a) Suggest an unbiased estimator for A, based on Y1, Y2, . . . ,Yn. (b) The weekly cost of repairing these breakdowns is C 2 SY +Y2, if Y is the number of breakdowns that week. Show that lE(C') = 4A + A2. (c) Find a function of Y1, Y2, . . . ,Yn that is an unbiased estimator of 151(0) Hint: Use what you know about IE(Y) and lE(Y2)