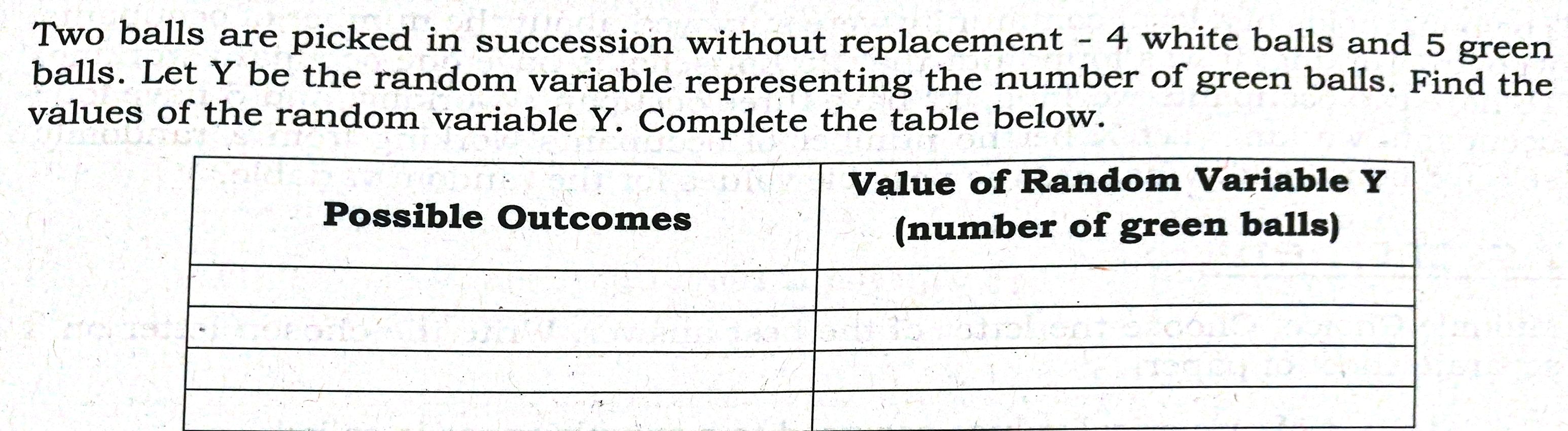
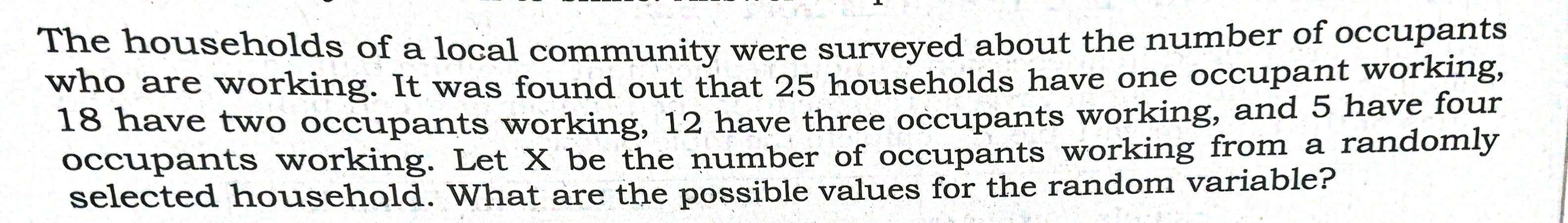5. Number of times the first card is face card and the second card is not a face cardTwo balls are picked in succession without replacement 4 white balls and. 5 green balls. Let Y be the random variable representing the number of green balls. Find the values of the random Variable Y. Complete the table belOW- . ' - ' .. _ ~ . Value of Random Variable Y , Possible Outcomes ' " (number 01' green balls) Four coins are toss. Let Y be the random variable representing the number of tails that occur. Find the values of the random variable Y. Value of Random Variable Y Possible Outcomes (number of tails)Give your answer in the following statements. 1. A is a set of possible values from a random experiment. For example, in tossing a coin, we can either get head or tail, such tossing a coin is an experiment where we can give values for such event. 2. A is one that may take on only a countable number of distinct values such as 0,1,2,3,4, etc. This variable is usually (but not necessarily) counts. It is a random variable that can take only a finite number of distinct values. 3. To find the values of a random variable, we have to follow these steps: first, list all in sample space; second, find the for each simple event; third, list the possible values for a random variable X and identify the value for each simple event and finally; find all simple events for which X = k, for each possible value k.The households of a local community were surveyed about the number of occupants who are working. It was found out that 25 households have one occupant working, 18 have two occupants working, 12 have three occupants working, and 5 have four occupants working. Let X be the number of occupants working from a randomly selected household. What are the possible values for the random variable?1.A set of numerical values assigned to a sample space is called A. Random sample C. Random variable B. Random process D. Random experiment 2. Which of the following is a true statement? A. Random variables can only have one value. B. The value of a random variable could not be zero. C. The probability of all the value of a random variable could be zero. D. The sum of all the probabilities in a probability distribution is not equal to one. 3.If a coin is tossed, what are the possible values of the random variable for the number of tails? A. 0, 1 C. 1, 2, 3 B. 0, 1, 2 D. O, 1, 2, 3 For numbers 4 - 9. Suppose you tossed three coins. 4. What are the sample spaces for the experiment above? A. HHH, TTT B. TTT, HHH, TTH, THT, HTH C. TTT, HHH, HHT, THT, HTH D. TTT, TTH, THT, HTT, HHT, HTH, THH, HHH 5. How many possible outcomes will occur from the experiment above? A. 2 C. 6 B. 4 D. 8 6. What are the possible values of the random variable for the number of heads? A. 0, 1 C. 1, 2, 3 B. 0, 1, 2 D. 0, 1, 2, 37- What are the'possible values of the random variable for the number of tails? ' A' 0' 1 c. 1, 2, 3 B.0,1,2' 110,1,2'3 8' WhiCh Of 1111!? fOllowing is NOT a possible value of the random variable for the number of heads? A" 1 - c. 3 Bl 2 D. 4 9- Web of the following is NOT a possible value of the random variable for the number of tails? A. 1 ' C. 3 'B- 2 i D. 4 For numbers 10 - '1 1. You decide to conduct a survey of families with two children. You are interested in counting the number of beys (out of 2 child' ren) in each family 10.. Is the above experiment i'counting the number of boys (out of 2 children) in each family\" a random variable? A. Yes, it. is a random variable. . ' C. Maybe. B. No, it is not'a random variable. 'D.' It cannot be determined. 1 1 .If the statement above shows ,a'random variable, then what are the possible values? ' ' - . A. Itsvaluecanbelorz. ' . ' ' B. Its value can be 0, 1, or 2. K C. Its value can be 2 or 4. l2). None, since it is not a random variable. ' For numbers .12 -~ 13.' Suppose tWo balls are drawn in' succession without y replacement from an urn containing 6 blue balls and 5 red balls. 12.HOW many possible outcomes will occur from the experiment? A.'2 ' ' V ' ' V _ . c. 6 . B. 4 . ' ' V , D. 8 . 13. Lot Z be the random variable representing the number of blue balls. What are the possible values of the random variable? - A.O,1 _ _ ' C.1,2,3 B. 0, 1,2 ' , ' D. 0, 1,2,3 7 For numbers l4 - 15. Suppose three laptops are'tested. Let D represent the defective laptop and N for the. non-defective laptop. . m; ' 14. How many possible outcomes will occur from the experiment? A. 3 C. 8 B. 4 D. 9 15. If we let X be the random variable representing the number of non-defective laptops. What are the possible values of the random variable? A. 0, 1 C. 1, 2, 3 B. 0, 1, 2 D. 0, 1, 2, 3Answer the following. An umbrella - check girl returns 2 umbrellas at random to 2 customers who had previously checked in, namely Andrea and Althea. If Andrea's umbrella was black and Althea's umbrella was Yellow: 1. list the elements of the sample space, S - all possible orders in which the umbrellas can be returned, and; 2. list all possible values of the random variable X, the number of correct matches.1. A set of numerical values assigned to a sample space is called A. Random sample B. Random variable C. Random Process A. Random experiment 2. Which of the following best describe variable that can be counted? A. Categorical B. Continuous C. Discrete D. Ordinal 3. Which of the following best describe variable that can be measured? A. Categorical B. Continuous C. Discrete D. Ordinal 4. You decided to order a pizza but you have to choose the type of crust and the toppings. If there are only 6 possible combinations of ordering a pizza, from which of the following should you choose? A. Crust: thin or deep dish Toppings: cheese or pepperoni B. Crust: thin or deep dish Toppings: cheese, bacon or pepperoni C. Crust: thin or deep dish Toppings: cheese, bacon, sausage or pepperoni D. Crust: thin or deep dish Toppings: cheese, bacon, sausage, pepperoni or hotdog 5. A drawer has 2 red ties, 3 black ties and 5 blue ties. A tie is picked at random. What is the total number of possible outcomes? A. 2 B. 3C. 5 D. 10 6. Which of the following statement describe a continuous random variable? A. The number of students present in a class B. The average distance traveled by a jeep in a week C. The number of correct guesses on a multiple choice test D. The number of women taller than 68 inches in a random sample of 5 Women 7. Which of the following is a continuous random variable? A. Height of sunflower as measured each day B. Number of gadgets a business sell per day C. Number of people attending mass every Sunday D. Number of people eating at the restaurant everyday 8. Which of the following is discrete random variable? A. Hipolito weighs 65 kg B. Hipolito is 160 cm tall C. Hipolito has two brother D. Hipolito ran 100 meters in 10.2 seconds 9. Which of the following variable is discrete random variable? A. Lifetime of a AAA battery B. The amount of gasoline in a car C. Number of goals of a football team D. The time it takes to commute to word 10. Which of the following is NOT a discrete random variable? A. Height of rose as measured each day B. Number of appliances a business sell each day C. Number of people went to church every Sunday D. Number of students late in going to school each day 11. Which of the following statement DOES NOT describe a discrete random variable? A. The number of children who do household chores everyday B. The average amount of milk consumption of a baby each day C. The number of students who excelled in mathematics in a certain grade level D. The number of children who love to pray a rosary every 6 o'clock in the afternoon . 12. Which of the following statement DOES NOT describe a continuous random variable? A. The distance traveled by a truck in an hour B. The average height of a coconut tree each day C. The number of provinces belong to Region IV-A D. The intensity of an earthquake that happens last month 13. Which of the following is NOT a continuous random variable? A. The amount of water on a jug B. The length of time attending a "Webinar" C. The number of OFW who traveled abroad each day D. The height of the tallest mountain in the Philippine14. You decide to collect a bunch of cans of soda and measure the volume of soda in each can. Let x be the number of soda in each can. What type of variable is x? A. x is a constant B. x is a place holder C. x is a discrete random variable . D. x is acontinuous random variable , y a 15. You decided. to conduct a survey of families With tWo children. You are interested in counting the number Of girls (out of 2 children) in each family. Is this a random variable? ' A. Yes, it is a random variable B. No, it is not a random variable C. Maybe ' D. Cannot be determine 1. A quantity resulting from an experiment by chance that can assume different values is called A. Ran dom sample B. Random variable C. Random process D. Random experiment 2. Which of the following is NOT a true statement? A. Random variables can only have one value. B. The value of a random variable could be zero. C. The probability of all the value of a random variable could be zero. D. The sum of all the probabilities in a probability distribution is always equal to one. 3. If a coin is tossed, what are the possible values of the random variable for th number of heads? A. O, 1 B. 0, 1, 2 C. 1, 2, 3 D. 0, 1, 2, 3 For numbers 4 - 9. Suppose you tossed two coins. 4. What are the sample spaces for the experiment above? A. HH, TT B. HH, HT, TT C. HH, TH, TT D. HH, HT, TT, TH 5. How many possible outcomes will occur from the experiment above? A. 2 B. 3 C. 4 D. 5 6. What are the possible values of the random variable for the number of heads? A. 0, 1 B. 0, 1, 2C. 1, 2, 3 D. O, 1, 2, 3 7. What are the possible values of the random variable for the number of tails? A. O, 1 B. O, 1, 2 C. 1, 2, 3 D. O, 1, 2, 3 8. Which of the following is NOT a possible value of the random variable for the number of heads? A. 1 B. 2 C. 3 D. 4 9. Which of the following is NOT a possible value of the random variable for the number of tails? A. 4 B. 3 C. 2 D. 1 For numbers 10 - 11. You decide to conduct a survey of families with two children. You are interested in counting the number of girls (out of 2 children) in each family. 10. Is the above experiment "counting the number of girls (out of 2 children in each family" a random variable? A. Yes, it is a random variable. B. No, it is not a random variable. C. Maybe. D. It cannot be determined. 11. If the experiment above shows a random variable, then what are the possible values of it? A. Its value can be 1 or 2. B. Its value can be 0, 1, or 2. C. Its value can be 2 or 4. D. None, since it is not a random variable. 12. In a local community, a couple was asked the questions, "Are you satisfied with the work of the current president?" If the husband and the wife both said "yes", the response is written as YY. If the husband said yes and the wife said "no", the response is YN. Let X be the number of "yes" responses, what are the possible values of the random variables? A. 0, 1, 2 B. 1, 2, 3 C. 2, 3, 4 D. 1, 1, 2 For numbers 13 - 15. Suppose two laptops are tested. Let D represent the defective laptop and N represent the non-defective laptop.13. What are the possible sample spaces for the experiment? A. DD, NN B. DD, DN, NN C. DD, ND, NN D. DD, DN, NN, ND 14. How many possible outcomes will occur from the experiment? A. 2 B. 3 C. 4 D. 5 15. If we let X be the random variable representing the number of defective laptops. What are the possible values of the random variable? A. 0, 1 B. 0, 1, 2 C. 1, 2, 3 D. 0, 1, 2, 3Suppose three laptops are tested at random. We want to find out the number of non-defective laptops. Thus, to each outcome in the sample space we shall assign a value. These are 0, 1, 2, or 3. If there is no defective laptop, we assign the number O; if there is 1 non-defective laptop, we assign the number 1; if there are two non- defective laptops, we assign the number 2 and 3, if there are three non- defective laptops. The number of non-defective laptops is a random variable. Let D represents the defective laptop and N represents the non-defective laptop. If we let X be the random variable representing the number of non-defective laptops, show the values of the random variable X. Complete the table below to show the values of the random variable. Value of the Random Variable X Possible Outcomes (number of non-defective laptops) This time, you need to reflect on the following questions because it will help you to understand the appropriate way in finding the possible values of a random variable. Answer the following questions: 1. How do you find the activity? 2. Were you able to complete the table? If yes, how? If no, why? 3. Will you be able to find the values of a random variable? If yes, how? If no, why? 4. In your own words, how will you describe a random variable? 5. How do you find the possible values of a random variable?Understanding Possible Values of Random Variables. Answer the following questions. 1. How do you find the values of a random variable? 2. How is this variable, as described in Algebra, similar to a random variable? How do they differ