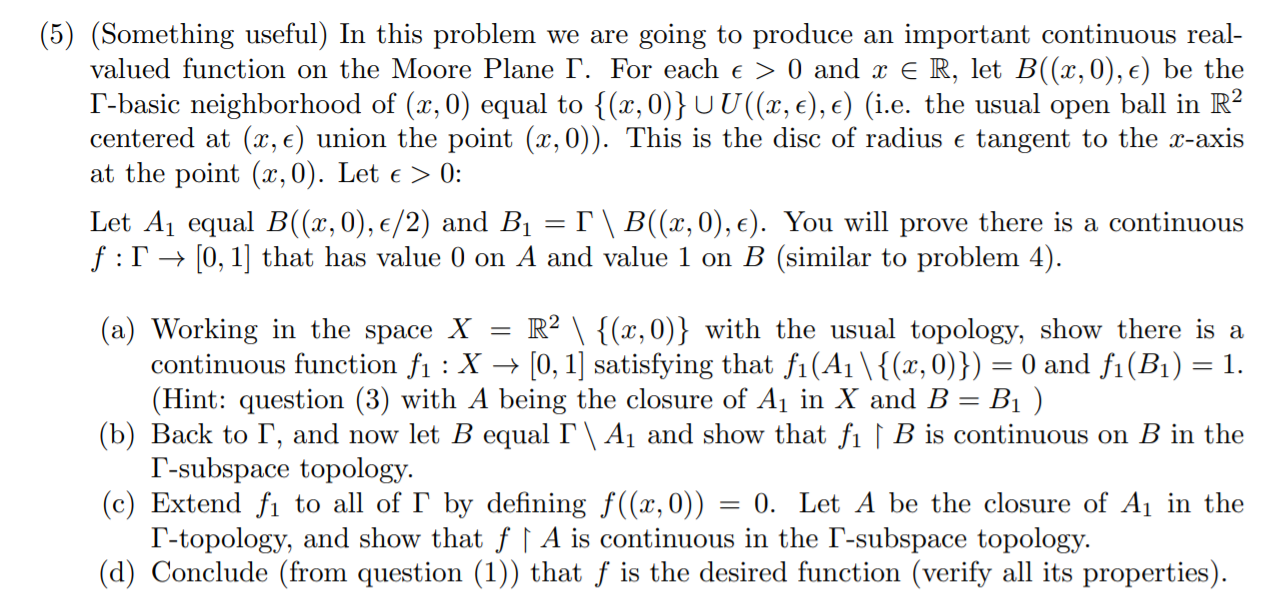
(5) (Something useful) In this problem we are going to produce an important continuous real- valued function on the Moore Plane T. For each e > 0 and x R, let B((x,0), e) be the T-basic neighborhood of (x,0) equal to {(x, 0)} U U((x, ), e) (i.e. the usual open ball in R2 centered at (x,c) union the point (4,0)). This is the disc of radius e tangent to the x-axis at the point (x,0). Let e > 0: Let A1 equal B((x,0), e/2) and Bi T\B((x,0), e). You will prove there is a continuous f:I [0, 1] that has value 0 on A and value 1 on B (similar to problem 4). (a) Working in the space X R2 \ {(x,0)} with the usual topology, show there is a continuous function f : X [0, 1] satisfying that f(A1\{(x,0)}) = 0 and fi(B1) = 1. (Hint: question (3) with A being the closure of Aj in X and B = Bi ) (b) Back to I, and now let B equal I \ A1 and show that fi | B is continuous on in the T-subspace topology. (c) Extend fi to all of I by defining f((x,0)) 0. Let A be the closure of A1 in the T-topology, and show that f | A is continuous in the T-subspace topology. (d) Conclude (from question (1)) that f is the desired function (verify all its properties). (5) (Something useful) In this problem we are going to produce an important continuous real- valued function on the Moore Plane T. For each e > 0 and x R, let B((x,0), e) be the T-basic neighborhood of (x,0) equal to {(x, 0)} U U((x, ), e) (i.e. the usual open ball in R2 centered at (x,c) union the point (4,0)). This is the disc of radius e tangent to the x-axis at the point (x,0). Let e > 0: Let A1 equal B((x,0), e/2) and Bi T\B((x,0), e). You will prove there is a continuous f:I [0, 1] that has value 0 on A and value 1 on B (similar to problem 4). (a) Working in the space X R2 \ {(x,0)} with the usual topology, show there is a continuous function f : X [0, 1] satisfying that f(A1\{(x,0)}) = 0 and fi(B1) = 1. (Hint: question (3) with A being the closure of Aj in X and B = Bi ) (b) Back to I, and now let B equal I \ A1 and show that fi | B is continuous on in the T-subspace topology. (c) Extend fi to all of I by defining f((x,0)) 0. Let A be the closure of A1 in the T-topology, and show that f | A is continuous in the T-subspace topology. (d) Conclude (from question (1)) that f is the desired function (verify all its properties)