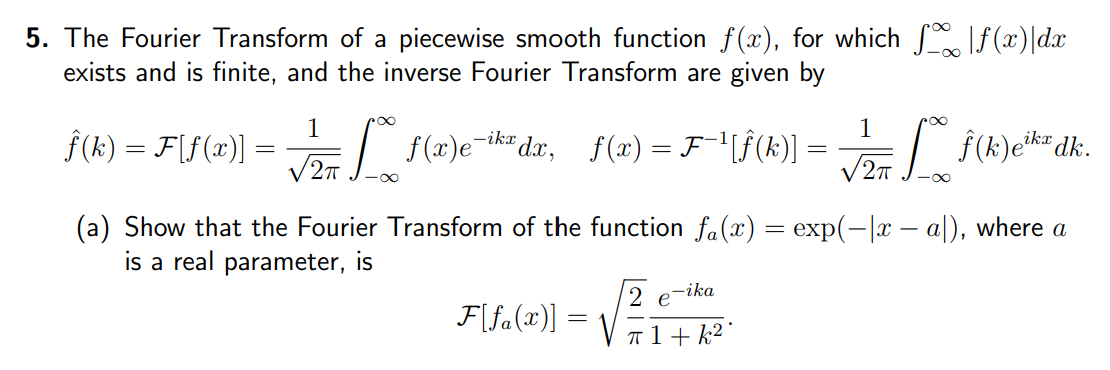
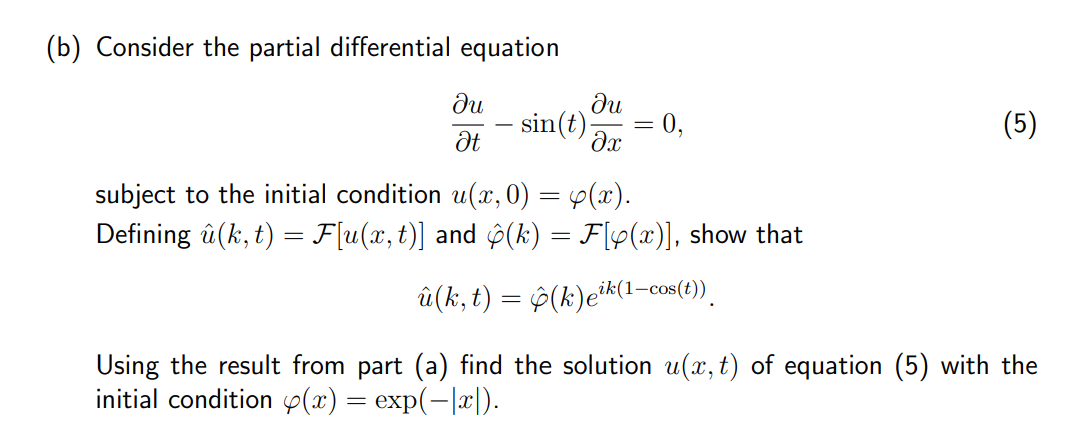
5. The Fourier Transform of a piecewise smooth function f(x), for which f(x)|dx exists and is finite, and the inverse Fourier Transform are given by (k) = F[(x)] = /12/20 /2 1 (x)eikdx,_(x) = F[(k)] = 2/(k) e dk. (k)eikz - (a) Show that the Fourier Transform of the function f(x) is a real parameter, is F[fa(x)] = = e-ika 1+k = = exp(-x - a), where a (b) Consider the partial differential equation - sin(t). t x = 0, subject to the initial condition u(x, 0) = 4(x). Defining (k, t) = F[u(x,t)] and (k) = F[y(x)], show that (k, t) = (k)ek(1-cos(t)) (5) Using the result from part (a) find the solution u(x, t) of equation (5) with the initial condition (x) = exp(-|x|).