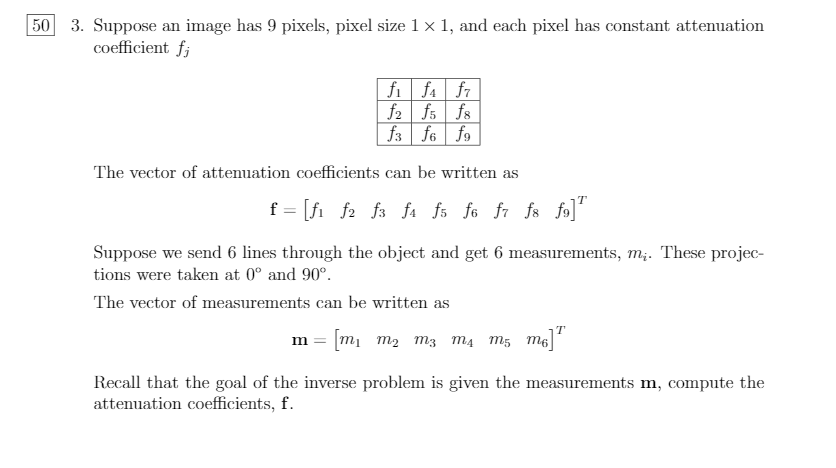
50 3. Suppose an image has 9 pixels, pixel size 1 x 1, and each pixel has constant attenuation coefficient fi fi f1 f1 f2 f5 fo f3 f6 f. The vector of attenuation coefficients can be written as f = [fi f2 f; fa fs fo f7 fo fa]" Suppose we send 6 lines through the object and get 6 measurements, mi. These projec- tions were taken at 0 and 90. The vector of measurements can be written as m [m m2 m3 m4 m5 me]" Recall that the goal of the inverse problem is given the measurements m, compute the attenuation coefficients, f. (e) Compute a basis for the row space of A: R(AT). (f) Use your computed vectors and the Fundamental Theoreom of Linear Algebra to construct a basis for R. (g) The next question investigates how many projections we need until Af = m admits a unique solution, assuming that a solution exists. To answer this question, find the measurement matrices corresponding to more projection images and use Matlab to compute the ranks of these matrices. Hint: Start with 2 projection images (matrix from question 1) and compute the rank of A. Add a projection at 45 (see class notes) to get 3 projection images. Continue to add more projections until A has full column rank. 50 3. Suppose an image has 9 pixels, pixel size 1 x 1, and each pixel has constant attenuation coefficient fi fi f1 f1 f2 f5 fo f3 f6 f. The vector of attenuation coefficients can be written as f = [fi f2 f; fa fs fo f7 fo fa]" Suppose we send 6 lines through the object and get 6 measurements, mi. These projec- tions were taken at 0 and 90. The vector of measurements can be written as m [m m2 m3 m4 m5 me]" Recall that the goal of the inverse problem is given the measurements m, compute the attenuation coefficients, f. (e) Compute a basis for the row space of A: R(AT). (f) Use your computed vectors and the Fundamental Theoreom of Linear Algebra to construct a basis for R. (g) The next question investigates how many projections we need until Af = m admits a unique solution, assuming that a solution exists. To answer this question, find the measurement matrices corresponding to more projection images and use Matlab to compute the ranks of these matrices. Hint: Start with 2 projection images (matrix from question 1) and compute the rank of A. Add a projection at 45 (see class notes) to get 3 projection images. Continue to add more projections until A has full column rank