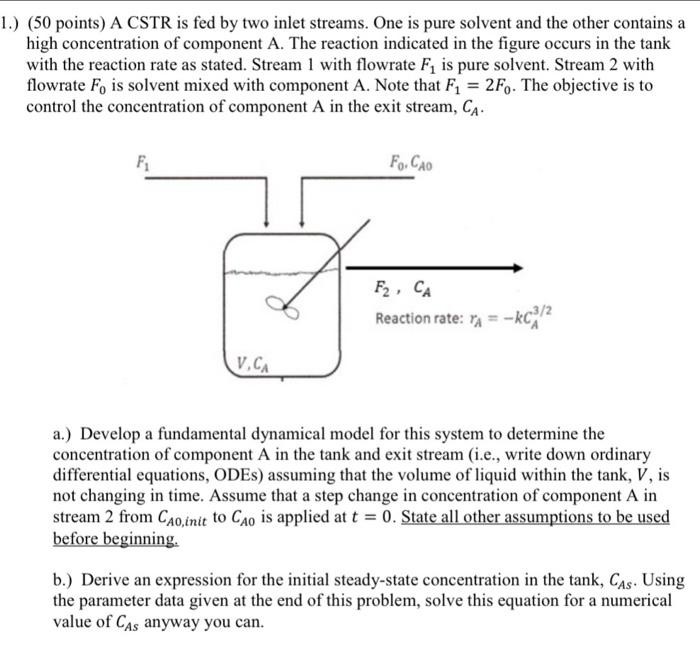
(50 points) A CSTR is fed by two inlet streams. One is pure solvent and the other contains a high concentration of component A. The reaction indicated in the figure occurs in the tank with the reaction rate as stated. Stream 1 with flowrate F1 is pure solvent. Stream 2 with flowrate F0 is solvent mixed with component A. Note that F1=2F0. The objective is to control the concentration of component A in the exit stream, CA. a.) Develop a fundamental dynamical model for this system to determine the concentration of component A in the tank and exit stream (i.e., write down ordinary differential equations, ODEs) assuming that the volume of liquid within the tank, V, is not changing in time. Assume that a step change in concentration of component A in stream 2 from CA0,init to CA0 is applied at t=0. State all other assumptions to be used before beginning. b.) Derive an expression for the initial steady-state concentration in the tank, CAs. Using the parameter data given at the end of this problem, solve this equation for a numerical value of CAS anyway you can. c.) Is the ODE of part a.) linear or nonlinear? If it is nonlinear, write down a linearized approximation of the differential equation around the initial steady-state reactor concentration, CAs. d.) Express the linear system in terms of deviation variables (CA and CA0) with respect to CAs and the initial inlet concentration, CA0,init. e.) What is the expression for the time constant of the linearized system? f.) Solve the linearized equation for CA(t). g.) What is the expression for the steady-state gain of the process? h.) Using the values of the parameters tabulated below, make a plot of the linearized solution CA(t) for t[0,20] (min) using an MS Excel spreadsheet. i.) Rewrite the original (nonlinear) ODE for CA(t) in terms of the deviation variables CA(t) and CA0, and then solve the ODE numerically using Euler's forward finite difference method in MS Excel. Plot the solution CA(t) for t[0,20] (min) on the same plot as the linearized solution in part h.). Be sure to write out the formula you are using for Euler's method on your exam page. j.) How long does it take to achieve 95% of the final steady-state tank concentration using both the linear and nonlinear models? Data: F0=0.085m3/min;V=2.1m3;CA0,init=0.925mol/m3;CA0=0.925mol/m3;CA0=1.85mol/m3(afterstep);F1=2F0;k=0.050(m3)1/2(minmol1/2)1