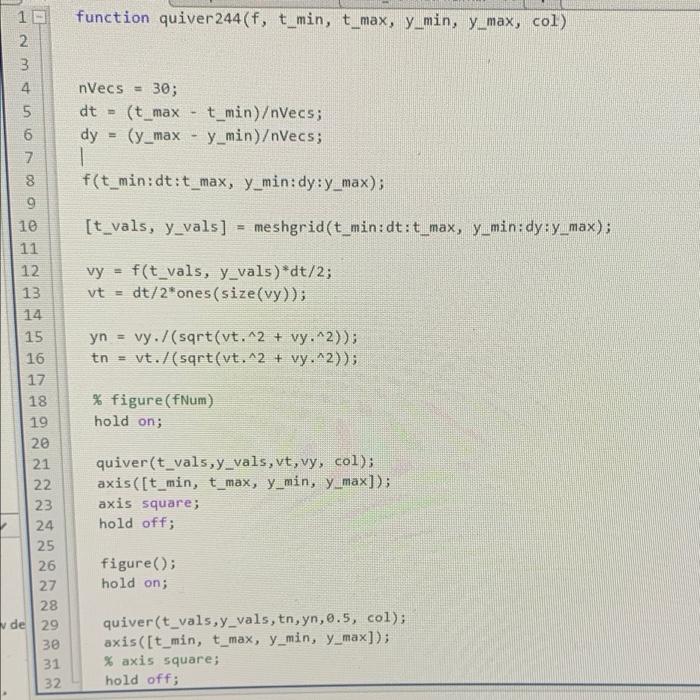
6. Consider the differential equntion dy dt U quivor244m to draw a quiver plot of this differentiat untion on and 10. What does it look like the solution will to If we hart with y(0) 3? What about for y(0) = 0 pr y(0) 8? Provide a description of what will happen to the solution. Note: For putting in together, remember thint the anonymous fonction needs to be written AR O(t,y), and the order of the varinbles is important. function quiver 244(f, t_min, t_max, y_min, y_max, col) - 1 2 3 4 5 6 7 8 9 10 11 12. 13 14 15 nVecs = 30; dt = (t_max t_min)Vecs; dy = (y_max y_min)Vecs; 1 f(t_min:dt:t_max, y_min:dy:y_max); [t_vals, y_vals] = meshgrid(t_min:dt:t_max, y min:dy:y_max): vy = f(t_vals, y_vals)*dt/2; vt = dt/2*ones (size(vy)); yn = tn = vy./(sqrt(vt. ^2 + vy. -2)); vt./(sqrt(vt.^2 + vy.^2)); 16 % figure (fNum) hold on; 17 18 19 20 21 22 23 24 25 26 27 28 de 29 3e 31 32 quiver(t_vals,y_vals, vt,vy, col); axis ([t_min, t_max, y_min, y_max]); axis square; hold off; figure(); hold on; quiver(t_vals,y_vals, tn, yn, 0.5, col); axis([t_min, t_max, y_min, y_max]); % axis square; hold off; 6. Consider the differential equntion dy dt U quivor244m to draw a quiver plot of this differentiat untion on and 10. What does it look like the solution will to If we hart with y(0) 3? What about for y(0) = 0 pr y(0) 8? Provide a description of what will happen to the solution. Note: For putting in together, remember thint the anonymous fonction needs to be written AR O(t,y), and the order of the varinbles is important. function quiver 244(f, t_min, t_max, y_min, y_max, col) - 1 2 3 4 5 6 7 8 9 10 11 12. 13 14 15 nVecs = 30; dt = (t_max t_min)Vecs; dy = (y_max y_min)Vecs; 1 f(t_min:dt:t_max, y_min:dy:y_max); [t_vals, y_vals] = meshgrid(t_min:dt:t_max, y min:dy:y_max): vy = f(t_vals, y_vals)*dt/2; vt = dt/2*ones (size(vy)); yn = tn = vy./(sqrt(vt. ^2 + vy. -2)); vt./(sqrt(vt.^2 + vy.^2)); 16 % figure (fNum) hold on; 17 18 19 20 21 22 23 24 25 26 27 28 de 29 3e 31 32 quiver(t_vals,y_vals, vt,vy, col); axis ([t_min, t_max, y_min, y_max]); axis square; hold off; figure(); hold on; quiver(t_vals,y_vals, tn, yn, 0.5, col); axis([t_min, t_max, y_min, y_max]); % axis square; hold off